本文主要是介绍[POJ3070]Fibonacci,希望对大家解决编程问题提供一定的参考价值,需要的开发者们随着小编来一起学习吧!
Fibonacci
Time Limit: 1000MS Memory Limit: 65536K
Description
In the Fibonacci integer sequence, F0 = 0, F1 = 1, and Fn = Fn − 1 + Fn − 2 for n ≥ 2. For example, the first ten terms of the Fibonacci sequence are:
0, 1, 1, 2, 3, 5, 8, 13, 21, 34, …
An alternative formula for the Fibonacci sequence is
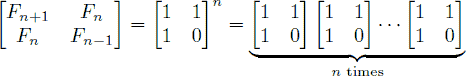
.
Given an integer n, your goal is to compute the last 4 digits of Fn.
Input
The input test file will contain multiple test cases. Each test case consists of a single line containing n (where 0 ≤ n ≤ 1,000,000,000). The end-of-file is denoted by a single line containing the number −1.
Output
For each test case, print the last four digits of Fn. If the last four digits of Fn are all zeros, print ‘0’; otherwise, omit any leading zeros (i.e., print Fn mod 10000).
Sample Input
0
9
999999999
1000000000
-1
Sample Output
0
34
626
6875
Hint
As a reminder, matrix multiplication is associative, and the product of two 2 × 2 matrices is given by
.
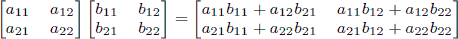
Also, note that raising any 2 × 2 matrix to the 0th power gives the identity matrix:
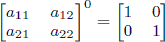
题解
- 矩阵乘法入门
- (不要脸地骗一道AC…..)
varx:array[0..10000]of longint;i,j,k:longint;t,y:array[1..2,1..2]of int64;n:longint;
function g(b:longint):longint;
var c,d,e,f:int64;
beginif b<0 then exit(0);t[1,1]:=1; t[1,2]:=0; t[2,1]:=0; t[2,2]:=1;y[1,1]:=0; y[1,2]:=1; y[2,1]:=1; y[2,2]:=1;while b<>0 dobeginif (b and 1)=1then beginc:=(t[1,1]*y[1,1]+t[1,2]*y[2,1])mod k;d:=(t[1,1]*y[1,2]+t[1,2]*y[2,2])mod k;e:=(t[2,1]*y[1,1]+t[2,2]*y[2,1])mod k;f:=(t[2,1]*y[1,2]+t[2,2]*y[2,2])mod k;t[1,1]:=c; t[1,2]:=d; t[2,1]:=e; t[2,2]:=f;end;c:=(y[1,1]*y[1,1]+y[1,2]*y[2,1])mod k;d:=(y[1,1]*y[1,2]+y[1,2]*y[2,2])mod k;e:=(y[2,1]*y[1,1]+y[2,2]*y[2,1])mod k;f:=(y[2,1]*y[1,2]+y[2,2]*y[2,2])mod k;y[1,1]:=c; y[1,2]:=d; y[2,1]:=e; y[2,2]:=f;b:=b shr 1;end;exit((t[2,1]*x[0]+t[2,2]*x[1])mod k);
end;begink:=10000;readln(n);while n<>-1 dobeginx[0]:=0; x[1]:=1;writeln(g(n-1));readln(n);end;
end.这篇关于[POJ3070]Fibonacci的文章就介绍到这儿,希望我们推荐的文章对编程师们有所帮助!






