poj3070--Fibonacci(矩阵的快速幂)

In the Fibonacci integer sequence, F₀ = 0, F₁ = 1, and F_n = F_{n − 1} + F_{n − 2} for n ≥ 2. For example, the first ten terms of the Fibonacci sequence are:

0, 1, 1, 2, 3, 5, 8, 13, 21, 34, …

An alternative formula for the Fibonacci sequence is

.

Given an integer n, your goal is to compute the last 4 digits of F_n.

The input test file will contain multiple test cases. Each test case consists of a single line containing n (where 0 ≤ n ≤ 1,000,000,000). The end-of-file is denoted by a single line containing the number −1.

For each test case, print the last four digits of F_n. If the last four digits of F_n are all zeros, print ‘0’; otherwise, omit any leading zeros (i.e., print F_n mod 10000).

As a reminder, matrix multiplication is associative, and the product of two 2 × 2 matrices is given by

.

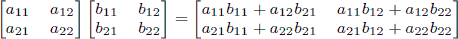Also, note that raising any 2 × 2 matrix to the 0th power gives the identity matrix:

.

#include <cstdio>
#include <cstring>
#include <algorithm>
using namespace std;
#define LL long long
struct node{
LL s11 , s12 , s21 , s22 ;
};
node f(node a,node b)
{
node p ;
p.s11 = (a.s11*b.s11 + a.s12*b.s21)%10000 ;
p.s12 = (a.s11*b.s12 + a.s12*b.s22)%10000 ;
p.s21 = (a.s21*b.s11 + a.s22*b.s21)%10000 ;
p.s22 = (a.s21*b.s12 + a.s22*b.s22)%10000 ;
return p ;
}
node pow(node p,int n)
{
node q ;
q.s11 = q.s22 = 1 ;
q.s12 = q.s21 = 0 ;
if(n == 0)
return q ;
q = pow(p,n/2);
q = f(q,q);
if( n%2 )
q = f(q,p);
return q ;
}
int main()
{
int n ;
node p ;
while(scanf("%d", &n) && n != -1)
{
p.s11 = p.s12 = p.s21 = 1 ;
p.s22 = 0 ;
p = pow(p,n);
printf("%d\n", p.s12);
}
return 0;
}