C/C++，优化算法——双离子推销员问题（Bitonic Travelling Salesman Problem）的计算方法与源代码

本文主要是介绍C/C++，优化算法——双离子推销员问题（Bitonic Travelling Salesman Problem）的计算方法与源代码，希望对大家解决编程问题提供一定的参考价值，需要的开发者们随着小编来一起学习吧！

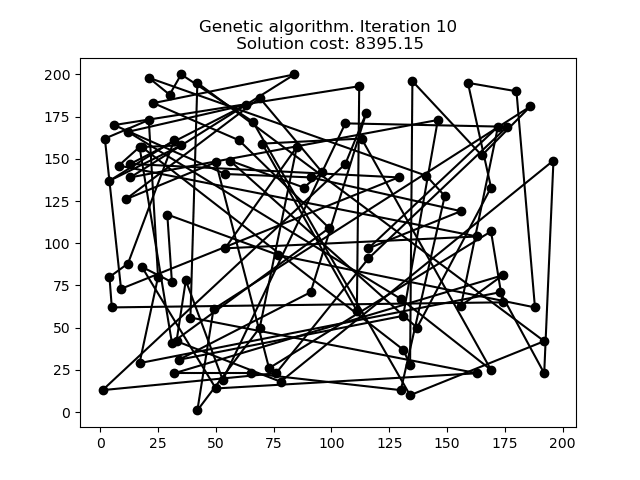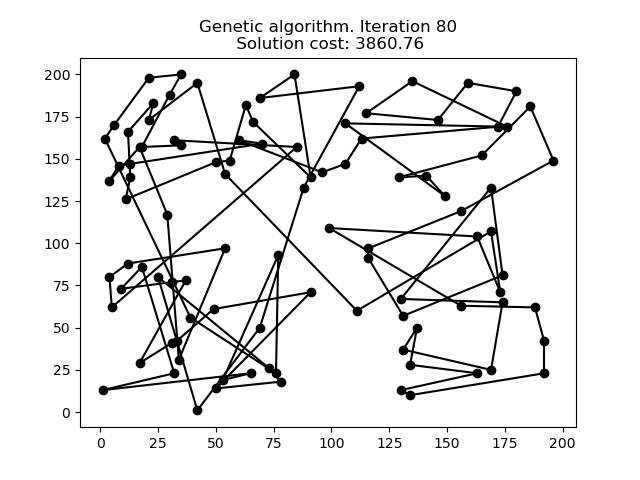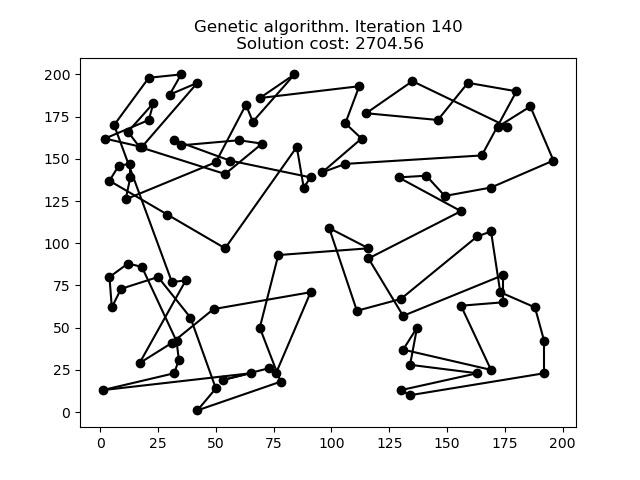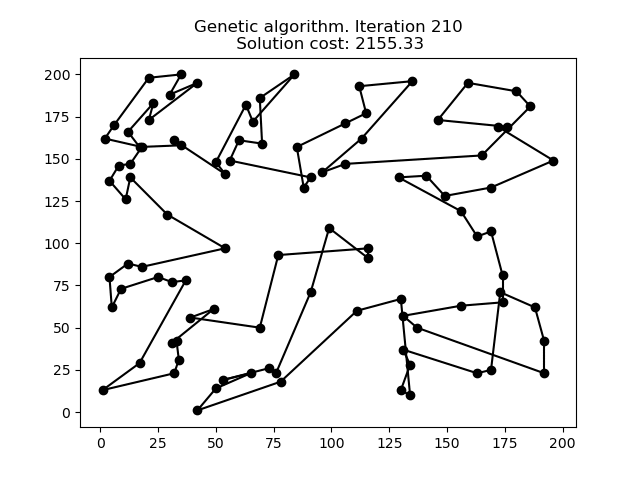
1 文本格式

// C++ program for the above approach
#include <bits/stdc++.h>
using namespace std;

// Size of the array a[]
const int mxN = 1005;

// Structure to store the x and
// y coordinates of a point
struct Coordinates {
    double x, y;
} a[mxN];

// Declare a 2-D dp array
float dp[mxN][mxN];

// Function to calculate the
// distance between two points
// in a Euclidian plane
float distance(int i, int j)
{
    // Return the distance
    return sqrt(
      (a[i].x - a[j].x) * (a[i].x - a[j].x)
    + (a[i].y - a[j].y) * (a[i].y - a[j].y));
}

// Utility recursive function to find
// the bitonic tour distance
float findTourDistance(int i, int j)
{
    // Memoization
    if (dp[i][j] > 0)
        return dp[i][j];

    // Update dp[i][j]
    dp[i][j] = min(
    findTourDistance(i + 1, j) + distance(i, i + 1),
    findTourDistance(i + 1, i) + distance(j, i + 1));

    return dp[i][j];
}

// Function to find the
// bitonic tour distance
void bitonicTSP(int N)
{
    // Initialize the dp array
    memset(dp, 0, sizeof(dp));

    // Base Case
    for (int j = 1; j < N - 1; j++)
        dp[N - 1][j] = distance(N - 1, N)
              + distance(j, N);

    // Print the answer
    printf("%.2f\n", findTourDistance(1, 1));
}

// Driver Code
int main()
{
    // Given Input
    int N = 3;
    a[1].x = 1, a[1].y = 1;
    a[2].x = 2, a[2].y = 3;
    a[3].x = 3, a[3].y = 1;

    // Function Call
    bitonicTSP(N);
}

2 代码格式

// C++ program for the above approach
#include <bits/stdc++.h>
using namespace std;// Size of the array a[]
const int mxN = 1005;// Structure to store the x and
// y coordinates of a point
struct Coordinates {double x, y;
} a[mxN];// Declare a 2-D dp array
float dp[mxN][mxN];// Function to calculate the
// distance between two points
// in a Euclidian plane
float distance(int i, int j)
{// Return the distancereturn sqrt((a[i].x - a[j].x) * (a[i].x - a[j].x)+ (a[i].y - a[j].y) * (a[i].y - a[j].y));
}// Utility recursive function to find
// the bitonic tour distance
float findTourDistance(int i, int j)
{// Memoizationif (dp[i][j] > 0)return dp[i][j];// Update dp[i][j]dp[i][j] = min(findTourDistance(i + 1, j) + distance(i, i + 1),findTourDistance(i + 1, i) + distance(j, i + 1));return dp[i][j];
}// Function to find the
// bitonic tour distance
void bitonicTSP(int N)
{// Initialize the dp arraymemset(dp, 0, sizeof(dp));// Base Casefor (int j = 1; j < N - 1; j++)dp[N - 1][j] = distance(N - 1, N)+ distance(j, N);// Print the answerprintf("%.2f\n", findTourDistance(1, 1));
}// Driver Code
int main()
{// Given Inputint N = 3;a[1].x = 1, a[1].y = 1;a[2].x = 2, a[2].y = 3;a[3].x = 3, a[3].y = 1;// Function CallbitonicTSP(N);
}

这篇关于C/C++，优化算法——双离子推销员问题（Bitonic Travelling Salesman Problem）的计算方法与源代码的文章就介绍到这儿，希望我们推荐的文章对编程师们有所帮助！

---