散点拟合曲线方程，并求点到曲线的最小距离

1、通过散点拟合出曲线方程并绘制出图形：

#coding=utf-8import matplotlib.pyplot as plt
import numpy as npx = [-1675, -1662, -1648, -1632, -1616, -1602, -1590, -1579, -1567, -1556, -1545, -1534, -1525, -1515, -1506, -1496, -1485, -1473, -1462, -1450, -1439, -1428, -1417, -1405, -1399, -1389, -1379]
y = [-3902, -3865, -3825, -3776, -3724, -3673, -3629, -3583, -3532, -3484, -3428, -3375, -3326, -3269, -3221, -3167, -3114, -3050, -2990, -2927, -2868, -2808, -2750, -2684, -2653, -2600, -2547]
z1 = np.polyfit(x, y, 4) #用4次多项式拟合，输出系数从高到0
#print(z1)
p1 = np.poly1d(z1) #使用次数合成多项式
y_pre = p1(x)plt.plot(x,y,'.')
plt.plot(x,y_pre)
plt.show()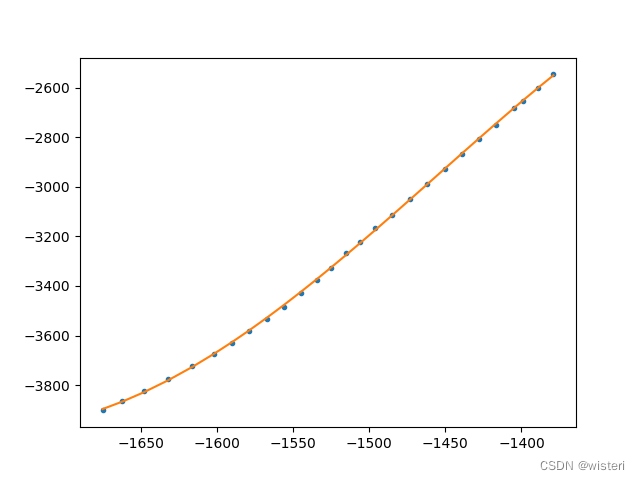

 2、求点到拟合曲线的最小距离并绘图显示

#coding=utf-8import numpy as np  
import matplotlib.pyplot as pltx = [-1675, -1662, -1648, -1632, -1616, -1602, -1590, -1579, -1567, -1556, -1545, -1534, -1525,-1515, -1506, -1496, -1485, -1473, -1462, -1450, -1439, -1428, -1417, -1405, -1399, -1389, -1379]
y = [-3902, -3865, -3825, -3776, -3724, -3673, -3629, -3583, -3532, -3484, -3428, -3375, -3326,-3269, -3221, -3167, -3114, -3050, -2990, -2927, -2868, -2808, -2750, -2684, -2653, -2600, -2547]
z1 = np.polyfit(x, y, 4) #用4次多项式拟合，输出系数从高到0
p1 = np.poly1d(z1) #使用次数合成多项式def distance(x, y, x0, y0):"""Return distance between pointP[x0,y0] and a curve (x,y)"""d_x = x - x0d_y = y - y0dis = np.sqrt( d_x**2 + d_y**2 )return disdef min_distance(x, y, P, precision=5):"""Compute minimum/a distance/s betweena point P[x0,y0] and a curve (x,y)rounded at `precision`.ARGS:x, y      (array)P         (tuple)precision (int)Returns min indexes and distances array."""# compute distanced = distance(x, y, P[0], P[1])d = np.round(d, precision)# find the minimaglob_min_idxs = np.argwhere(d==np.min(d)).ravel()return glob_min_idxs, ddef f(x):return p1(x)x = np.linspace(-1700, -1200, 1000)
y = f(x)P = (-1662, -3848)min_idxs, dis = min_distance(x, y, P)fig, ax = plt.subplots(figsize=(7, 7))ax.plot(x, y, lw=4)
for idx in min_idxs:ax.plot([P[0], x[idx]],[P[1], y[idx]],'--', lw=1,label=f'distance {dis[idx]:.2f}')
ax.plot(*P, 'or')
ax.text(P[0], P[1], f"  P ({P[0]}, {P[1]})", ha='left', va='center',fontsize=15
)
ax.set(xlim=(-1700, -1200),ylim=(-4000, -2400),
)
ax.legend()
plt.show()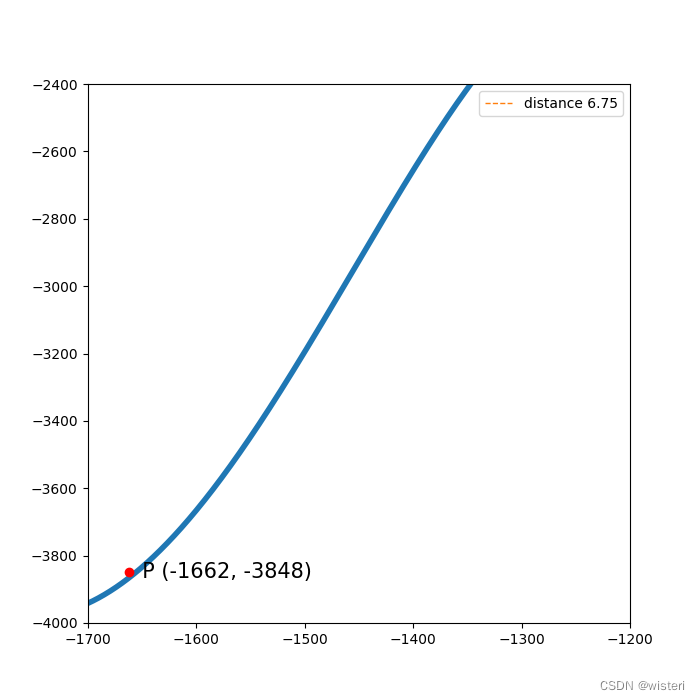

 3、返回点到拟合曲线的最小距离值

#coding=utf-8import numpy as np  
import matplotlib.pyplot as pltx = [-1675, -1662, -1648, -1632, -1616, -1602, -1590, -1579, -1567, -1556, -1545, -1534,-1525, -1515, -1506, -1496, -1485, -1473, -1462, -1450, -1439, -1428, -1417, -1405, -1399, -1389, -1379]
y = [-3902, -3865, -3825, -3776, -3724, -3673, -3629, -3583, -3532, -3484, -3428, -3375,-3326, -3269, -3221, -3167, -3114, -3050, -2990, -2927, -2868, -2808, -2750, -2684, -2653, -2600, -2547]
z1 = np.polyfit(x, y, 4) #用4次多项式拟合，输出系数从高到0
f1 = np.poly1d(z1) #使用次数合成多项式def distance(x, y, x0, y0):"""Return distance between pointP[x0,y0] and a curve (x,y)"""d_x = x - x0d_y = y - y0dis = np.sqrt( d_x**2 + d_y**2 )return disdef min_distance(x, y, P, precision=5):"""Compute minimum/a distance/s betweena point P[x0,y0] and a curve (x,y)rounded at `precision`.ARGS:x, y      (array)P         (tuple)precision (int)Returns min indexes and distances array."""# compute distanced = distance(x, y, P[0], P[1])d = np.round(d, precision)# find the minimaglob_min_idxs = np.argwhere(d==np.min(d)).ravel()return glob_min_idxs, ddef f1_min_distance(P):x = np.linspace(-1700, -1200, 1000)y = f1(x)min_idxs, dis = min_distance(x, y, P)return min(dis)if __name__ == '__main__':P = (-1662, -3848)d = f1_min_distance(P)print(d)

返回值 f1_min_distance(P) = 6.74534