A micro Lie theory for state estimation in robotics005:The tangent spaces and the Lie algebra

本文主要是介绍A micro Lie theory for state estimation in robotics005:The tangent spaces and the Lie algebra，希望对大家解决编程问题提供一定的参考价值，需要的开发者们随着小编来一起学习吧！

C. The tangent spaces and the Lie algebra

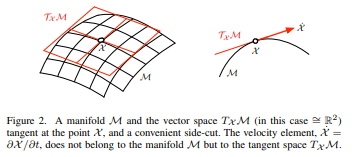
Given X (t) a point moving on a Lie group’s manifold M, its velocity $\overline{X}$ = ∂X /∂t belongs to the space tangent to M at X (Fig. 2), which we note TXM. The smoothness of the manifold, i.e., the absence of edges or spikes, implies the existence of a unique tangent space at each point. The structure of such tangent spaces is the same everywhere.
给定 X (t) 一个点在李群的流形 M 上移动，它的速度 $\overline{X}$ = ∂X /∂t 属于在 X 处与 M 相切的空间（图 2），即 TXM。 流形的平滑性，即没有边缘或尖峰，意味着每个点都存在唯一的切线空间。 这种切线空间的结构在任何地方都是一样的。

1- $TheLiealgebram:$

$Thetangentspaceattheidentity,T_{ϵ}M,iscalledtheLiealgebraofM,andnotedm,$
1-$李代数m：恒等式T_{ϵ}M处的切空间称为M的李代数，记为m，$
$$Liealgebra:m\triangleq T_{ϵ}M.(8)$$

Every Lie group has an associated Lie algebra. We relate the Lie group with its Lie algebra through the following facts [5] (see Figs. 1 and 6):
每个李群都有一个相关的李代数。 我们通过以下事实将李群与其李代数联系起来 [5]（见图 1 和图 6）：

$$•TheLiealgebramisavectorspace{.}^{注释1}Assuch,itselementscanbeidentifiedwithvectorsin{R}^m,whosedimensionmisthenumberofdegreesoffreedomofM.$$
• 李代数 m 是一个向量空间¹。 如此一来，其元素可以与Rm 中的向量等同，其维数 m 是 M 的自由度数。

$$•Theexponentialmap,exp:m\to M,exactlyconvertselementsoftheLiealgebraintoelementsofthegroup.Thelogmapistheinverseoperation.$$
• 指数映射exp : m → M，准确地将李代数的元素转换为群的元素。对数映射log是逆运算。

$$•VectorsofthetangentspaceatXcanbetransformedtothetangentspaceattheidentityEthroughalineartransform.Thistransformiscalledtheadjoint$$
•X 处的切线空间的向量可以通过线性变换转换为单位元 E 处的切线空间。 这种变换称为伴随

$$\begin{gathered} 一点z\in S^1恒定转速\omega ,z(t)=cos\omega t+i\sin \omega t.它通过1和z时的速度在各自的切线空间中:T_1{S}^1和T_z{S}^1. \\ 在T_{z}S1,速度\bar{z}=zi\omega =-\omega sin\omega t+i\omega cos\omega t当以全局坐标表示时,局部表达{时}^z{V}^{\wedge }=i\omega . \\ 他们之间的关系通{过}^z{V}^{\wedge }=z^{-1}\bar{z}=z\ast \bar{z}. \\ 在T_1{S}^1的例子中,关系就是等{式}^1{V}^{\wedge }=\bar{z}=i\omega . \\ 显然，所有切空间的结构都是iR，即李代数。这也是\bar{z}在单位处的结构，这就是为什么李代数被定义为单位处的切空间 \end{gathered}$$

Lie algebras can be defined locally to a tangent point X ,establishing local coordinates for TXM (Fig. 5). We shall
denote elements of the Lie algebras with a ‘hat’ decorator,
李代数可以局部定义到切点 X，为 TXM 建立局部坐标（图 5）。 我们将用“帽子”装饰器表示李代数的元素，
$suchas{V}^{\wedge }forvelocitiesor{\tau }^{\wedge }=(Vt{)}^{\wedge }=V^{\wedge }tforgeneralelements.$

A left superscript may also be added to specify the precise tangent space, e.g.,
也可以添加左上标来指定精确的切线空间，例如，
${}^x{V}^{\wedge }\in T_{x}Mand{}^{ϵ}{V}^{\wedge }\in T_{ϵ}M.$

The structure of the Lie algebra can be found (see Examples 3 and 5) by time-differentiating the group constraint (3).
通过对群要求的约束 (3) 进行时间微分，可以找到李代数的结构（参见示例 3 和 5）。

约束3是存在逆元

For multiplicative groups this yields the new constraint $x^{-1}\bar{x}+{\bar{x}}^{-1}x=0$, which applies to the elements tangent at X (the term X˙−1 is the derivative of the inverse). The elements of the Lie algebra are therefore of the form,${}^2$
对于乘法群来说这产生了新的约束:$x^{-1}\bar{x}+{\bar{x}}^{-1}x=0$,这适用于在 X 处相切的元素（项${\bar{x}}^{-1}$是逆的导数）。 因此，李代数的元素具有以下形式，²

$\bar{x}代表导数，本来是\dot{x}$

$$V^{\wedge }=x^{-1}\dot{x}=-\dot{x^{-1}}x(9)$$

2- $TheCartesianvectorspace{R}^m:$

The elements ${\tau }^{\wedge }$ of the Lie algebra have non-trivial structures (skew-symmetric matrices, imaginary numbers, pure quaternions, see Table I)
but the key aspect for us is that they can be expressed as linear combinations of some base elements Ei
, where Ei are called the generators of m (they are the derivatives of X around the origin in the i-th direction).
It is then handy to manipulate just the coordinates as vectors in Rm, which we shall note simply τ .
We may pass from m to Rm and vice versa through two mutually inverse linear maps or isomorphisms, commonly called hat and vee (see Fig. 6),
2-笛卡尔向量空间Rm：
元素 ${\tau }^{\wedge }$的李代数具有非平凡结构（斜对称矩阵、虚数、纯四元数，见表 I）
但对我们来说关键在于它们可以表示为一些基本元素 Ei 的线性组合
，其中 Ei 称为 m 的生成元（它们是 X 在第 i 个方向上围绕原点的导数）。
然后将坐标作为 Rm 中的向量进行操作是很方便handy的，我们将简单地记为 τ 。
我们可以通过两个互逆的线性映射或同构从 m 传递到 Rm，反之亦然，通常称为 hat 和 vee（见图 6），
$$\begin{gathered} Hat:R^m\to m;\tau \to {\tau }^{\wedge }=\sum _{i=1}^m{\tau }_i{E}_i(10) \\ Vee:m\to R^m;{\tau }^{\wedge }\to ({\tau }^{\wedge }{)}^{\vee }=\tau =\sum _{i=1}^m{\tau }_i{e}_i(11) \end{gathered}$$

图6:Mappings between the manifold M and the representations of its tangent space at the origin T_EM (Lie algebra m and Cartesian Rm).Maps hat (·)∧ and vee (·)∨ are the linear invertible maps or isomorphisms (10–11),
exp(·) and log(·) map the Lie algebra to/from the manifold, and Exp(·) and Log(·) are shortcuts to map directly the vector space Rm to/from M.
图6:流形 M 与其原点 T_EM 处的切空间表示（李代数 m 和笛卡尔 Rm）之间的映射。映射 hat (·)∧ 和 vee (·)∨ 是线性可逆映射或同构 (10-11)，
exp(·) 和 log(·) 将李代数映射到/从流形，而 Exp(·) 和 Log(·) 是直接映射向量空间 Rm 到/从 M 的快捷方式。

with eithe vectors of the base of Rm (we have $e_i^{\wedge }=E_i$).This means that m is isomorphic to the vector space Rm —one writes $m\simeq R^m$ , or ${\tau }^{\wedge }\simeq \tau$ .
Vectors τ ∈ Rm are handier for our purposes than their isomorphics τ∧ ∈ m, since they can be stacked in larger state vectors, and more importantly,manipulated with linear algebra using matrix operators. In this work, we enforce this preference of Rm over m, to the point that most of the operators and objects that we define (specifically: the adjoint, the Jacobians, the perturbations and their covariances matrices, as we will see soon) are on Rm

对于Rm的基向量基（我们有$e_i^{\wedge }=E_i$)这意味着m与向量空间Rm是同构的即:$m\simeq R^m$ ,或 ${\tau }^{\wedge }\simeq \tau$ .
对我们的目的来说，向量 τ ∈ Rm 比它们的同构 τ∧ ∈ m 更方便，因为它们可以堆叠在更大的状态向量中，更重要的是， 使用矩阵运算符使用线性代数进行操作。 在这项工作中，我们强制 Rm 优先于 m，以至于我们定义的大多数运算符和对象都在 Rm 上（特别是：伴随矩阵、雅可比矩阵、扰动及其协方差矩阵，我们很快就会看到 )

---

1. In a Lie algebra, the vector space is endowed with a non-associative product called the Lie bracket. In this work, we will not make use of it.在李代数中，向量空间被赋予一个称为李括号的非结合积。在这项工作中，我们不会利用它。 ↩︎

2. For additive Lie groups the constraint X −X = 0 differentiates to $\bar{x}=\bar{x}$ ,that is, no constraint affects the tangent space. This means that the tangent space is the same as the group space. See App. E for more details. ↩︎

这篇关于A micro Lie theory for state estimation in robotics005:The tangent spaces and the Lie algebra的文章就介绍到这儿，希望我们推荐的文章对编程师们有所帮助！

---