spaces专题

White spaces are required between publicId and systemId.

在配置applicationContext.xml时，编译器报错。是因为XML的标签顺序导致。 <?xml version="1.0" encoding="UTF-8"?> <beans xmlns="http://www.springframework.org/schema/beans" xmlns:xsi="http://www.w3.org/2001/XMLSchema

论文浅读之Mamba: Linear-Time Sequence Modeling with Selective State Spaces

介绍这篇论文提出了一种新型的"选择性状态空间模型"(Selective State Space Model, S6)来解决之前结构化状态空间模型(SSM)在离散且信息密集的数据（如文本）上效果较差的问题。 Mamba 在语言处理、基因组学和音频分析等领域的应用中表现出色。其创新的模型采用了线性时间序列建模架构，结合了选择性状态空间，能够在语言、音频和基因组学等不同模式中提供卓越的性能。这种突破

High-variance latent spaces

“High-variance latent spaces” 这个术语通常出现在机器学习和人工智能领域，特别是在涉及到生成模型、表示学习和特征学习的时候。在这个上下文中，“latent space” 指的是一个多维空间，其中每个点代表输入数据的一个潜在表示或特征向量。这个空间是“潜在”的，因为它不是直接观察到的数据，而是通过某种学习算法从原始数据中提取出来的。 “High-variance” 描述

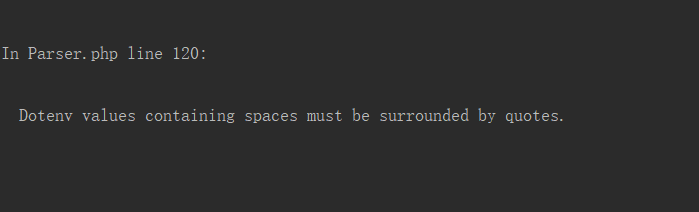
Laravel 报错: Dotenv values containing spaces must be surrounded by quotes.

报错信息如下: 原因: .env文件配置中包含空格的配置信息,用双引号""引起来即可。我是在配置项后面添加注释前面有空格，换行后显示正常。

python常见错误(1)TabError: inconsistent use of tabs and spaces in indentation

https://blog.csdn.net/Guopiqiang/article/details/73555898 问题：Python文件运行时报TabError: inconsistent use of tabs and spaces in indentation 原因：说明Python文件中混有Tab和Space用作格式缩进。这通常是使用外部编辑器编辑Python文件时，自动采用Tab进行

$Embeddings between Hölder spaces C^{0,β}↪C^{0,α}$

Embeddings between Hölder spaces C^{0,β}↪C^{0,α}

See https://math.stackexchange.com/questions/2617513/embeddings-between-h%c3%b6lder-spaces-c0-beta-hookrightarrow-c0-alpha

Java P2P Unleashed: With JXTA, Web Services, XML, Jini, JavaSpaces, and J2EE

WebStorm格式化vue错误解决 Expected indentation of 0 spaces but found 2

使用ctrl+alt+L格式化vue代码后，如果开启了eslint检查，会在script标签下看到一堆红色报警，通过下面的配置可以解决自动格式化后的这个问题。 File => Setting => Editor => Code Style => HTML，找到Other选项卡，Do not indent children of 的选项，添加 script 标签。然后再使用快捷键ctrl+alt+

error Mixed spaces and tabs no-mixed-spaces-and-tabs报错

vue尚硅谷todolist案例中报错如下： ERROR Failed to compile with 1 error 21:18:11 Module Error (from ./node_modules/e

ubuntu22.04@laptop OpenCV Get Started: 007_color_spaces

ubuntu22.04@laptop OpenCV Get Started: 007_color_spaces 1. 源由2. 颜色空间2.1 RGB颜色空间2.2 LAB颜色空间2.3 YCrCb颜色空间2.4 HSV颜色空间 3 代码工程结构3.1 C++应用Demo3.2 Python应用Demo 4. 重点分析4.1 interactive_color_detect4.2 inter

TabError:Inconsistent use of tabs and spaces in indentation

在NotePad++中为了让Tab键符号和空格键符号能区别的显示出来，需要进行设置。如果混用了空格符和制表符，需要进行替换。

【长文阅读】MAMBA作者博士论文＜MODELING SEQUENCES WITH STRUCTURED STATE SPACES＞-Chapter2

Gu A. Modeling Sequences with Structured State Spaces[D]. Stanford University, 2023. 本文是MAMBA作者的博士毕业论文，为了理清楚MAMBA专门花时间拜读这篇长达330页的博士论文，由于知识水平有限，只能尽自己所能概述记录，并适当补充一些相关数学背景，欢迎探讨与批评指正。内容多，分章节更新以免凌乱。 Chap

msn spaces被live了，不爽

Spaces又升级快一个月了。　　一个月来，上来看了很多次，可每次都觉得不爽，不现实的希望没有升这次级。从开通msn spaces以来，msn spaces升级了很多次，光是URL都换了好多个，阿风这云翼空间的URL，就有下面这好几个： http://spaces.msn.com/member/caizy 　　 http://spaces.msn.com/caizy 　　 http://

《Mamba: Linear-Time Sequence Modeling with Selective State Spaces》阅读笔记

论文标题《Mamba: Linear-Time Sequence Modeling with Selective State Spaces》利用选择性状态空间的线性时间序列建模作者 Albert Gu 和 Tri Dao Albert Gu 来自卡内基梅隆大学机器学习系，Mamba 脱胎于 Albert Gu 的前作 S4 架构。 Tri Dao 来自普林斯顿大学计算机科学系，M

mpvue 报错 Expected indentation of 2 spaces but found 4解决办法

当 mpvue 出现 Expected indentation of 2 spaces but found 4 类似报错的时候是因为开启了默认开启了 eslint 验证，它对 JS 中进行了缩进检查缩。解决方案就是，打开项目目录下的 build/webpack.base.conf.js，将其中的配置项暂时注释即可。注意：⚠️ 注释之后一定要重启项目，否则配置不会生效。

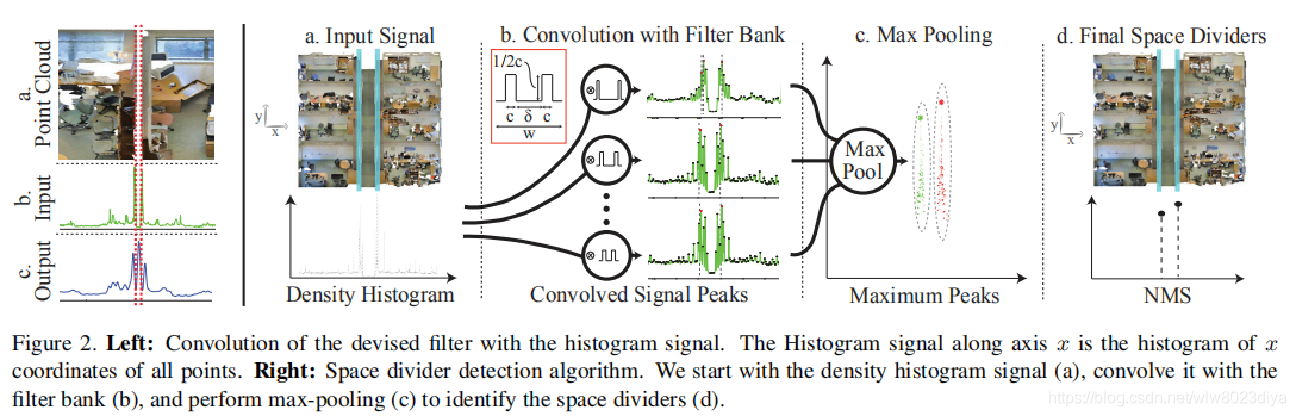
3D Semantic Parsing of Large-Scale Indoor Spaces

在本文中，作者提出了一种使用分层方法对整个建筑物的三维点云进行语义解析的方法：首先，将原始数据解析为语义有意义的空间(E 。g。房间等)对齐成一个典型的参考坐标系。第二，空间被解析成它们的结构和建筑元素(例如。墙壁、柱子等)。执行这些wi 全球三维空间的一个强有力的概念是我们方法的支柱。第一步中的对齐将强3D先验从正则坐标系注入到第二步以进行发现要素。这允许不同的具有挑战性的场景，因

《Mamba: Linear-Time Sequence Modeling with Selective State Spaces》阅读笔记

论文标题《Mamba: Linear-Time Sequence Modeling with Selective State Spaces》作者 Albert Gu 和 Tri Dao 初读摘要 Transformer 架构及其核心注意力模块地位：目前深度学习领域普遍的基础模型。为了解决 Transformers 在长序列上的计算效率低下的问题，已经开发了许多次二次时间

【多停车场车位预测】Prediction of Vacant Parking Spaces in Multiple Parking Lots:A DWT-ConvGRU-BRC Model

基于DWT-ConvGRU-BRC模型的多停车场车位预测期刊：applied science Received: 21 February 2023 Revised: 14 March 2023 Accepted: 15 March 2023 Published: 16 March 2023 摘要对于城市来说，“停车难、停车乱”的问题增加了碳排放，降低了生活质量。准确有效地预测空车位

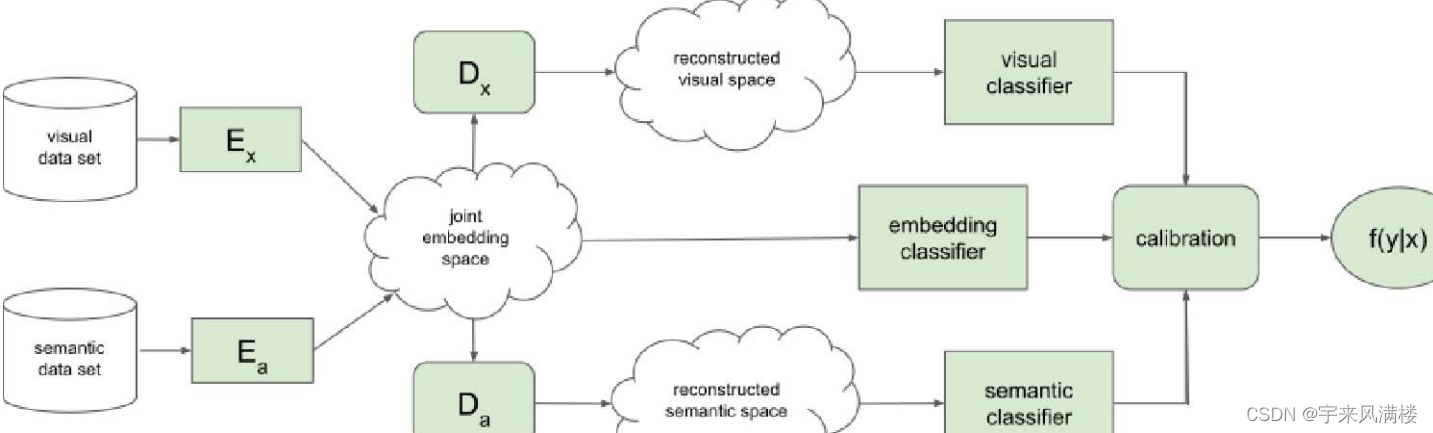
Generalised Zero-shot Learning with Multi-modal Embedding Spaces

分类器被 τ \tau τ校准辅助信息作者未提供代码

05 MIT线性代数-转置,置换,向量空间Transposes, permutations, spaces

1. Permutations P: execute row exchanges becomes PA = LU for any invertible A Permutations P = identity matrix with reordered rows m=n (n-1) ... (3) (2) (1) counts recordings, counts all nxn permu

LF.281.Remove Spaces

Given a string, remove all leading/trailing/duplicated empty spaces.Assumptions:The given string is not null.Examples:“ a” --> “a”“ I love MTV ” --> “I love MTV” 1 public String removeSpaces

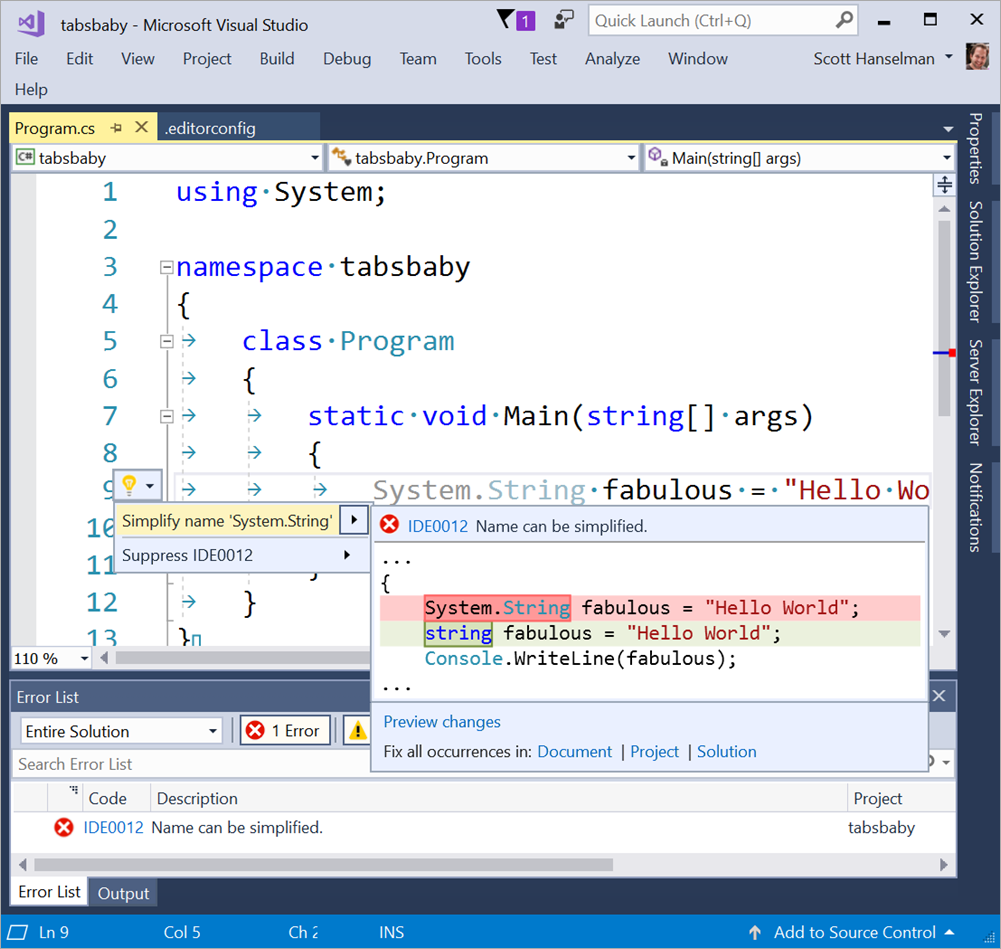
Tabs vs Spaces-在Visual Studio中使用EditorConfig进行和平解决。加上.NET扩展！

The culture wars continue. The country is divided with no end in sight. Tabs or spaces? There's even an insane (IMHO) assertion that the spaces people make more money. 文化大战仍在继续。这个国家分裂了，没有尽头。制表符或空格？

Confluence的An error occurred while searching for spaces引起的无法备份问题解决办法

由于施工原因，导致本地confluence服务器断电，重启后，confluence虽然有自动启动，使用上也没有问题，但是缺无法自动备份，一直报无法备份数据库，导致自动备份功能无法使用。根据报错日志提示缺少枚举啥的，搞了好几天，后面根据一直提示的空间问题，去空间搜索，发现无法搜索，发现报错“An error occurred while searching for spaces”，后排查发现可能

python出现错误： Sorry: TabError: inconsistent use of tabs and spaces in indentation

解决方案：需要统一输入格式，要不全用tab缩进，要不全用空格，不统一，会报错

A micro Lie theory for state estimation in robotics005:The tangent spaces and the Lie algebra

C. The tangent spaces and the Lie algebra Given X (t) a point moving on a Lie group’s manifold M, its velocity X ‾ \overline{X} X = ∂X /∂t belongs to the space tangent to M at X (Fig. 2), which we n