Project Euler 题解 #18 #67 Maximum path sum

本文主要是介绍Project Euler 题解 #18 #67 Maximum path sum，希望对大家解决编程问题提供一定的参考价值，需要的开发者们随着小编来一起学习吧！

题目：Maximum path sum I Maximum path sum II

By starting at the top of the triangle below and moving to adjacent numbers on the row below, the maximum total from top to bottom is 23.

3
7 4
2 4 6
8 5 9 3

That is, 3 + 7 + 4 + 9 = 23.

Find the maximum total from top to bottom in triangle.txt, a 15K text file containing a triangle with one-hundred rows.

这道题实质为路径搜索，可以用动态规划解决，但不能用暴力搜索。

数学描述

取层数为N，规模数为2^N。

这里采用"层降法"思想，O(N) = N²。

具体如下：

当处理到最后一层时，遍历一遍即可得到最大值。

由于一层一层的处理，那么代码实现时可以不用存储所有的数据，只需存储相邻的两层。

代码实现

//https://projecteuler.net/problem=18
//Maximum path sum I
//https://projecteuler.net/problem=67
//Maximum path sum II
#include "stdafx.h"
#include <iostream>
#include <memory>
#include <fstream>
#include <iomanip>
using namespace std;
#define MAX(a,b) ((a)>(b)? (a) : (b))
int _tmain(int argc, _TCHAR* argv[])
{
int N = 15; //数据的层数
int *old_line = new int[N];
int *new_line = new int[N];
memset(old_line, 0, N*sizeof(int));
memset(new_line, 0, N*sizeof(int));
fstream file("triangle.txt");
for (int i = 0; i< N; ++i)
{
for (int j = 0; j <= i; ++j)
{
file>>new_line[j];
cout<<setw(2)<<setfill('0')<<new_line[j]<<"  ";
if (i == 0 && j == 0)
{
continue;
}
else if (i > 0 && j == 0)
{
new_line[j] += old_line[j];
}
else if (i > 0 && j == i)
{
new_line[j] += old_line[j - 1];
}
else
{
new_line[j] += MAX(old_line[j-1], old_line[j]);
}
}
cout<<endl;
memcpy_s(old_line, N*sizeof(int), new_line, N*sizeof(int));
memset(new_line, 0, N*sizeof(int));
}
file.close();
int max_sum = 0;
for (int i = 0; i < N; ++i)
{
if (old_line[i] > max_sum)
{
max_sum = old_line[i];
}
}
cout<<"Max path sum = "<<max_sum<<endl;
delete old_line;
delete new_line;
system("pause");
return 0;
}

输入：

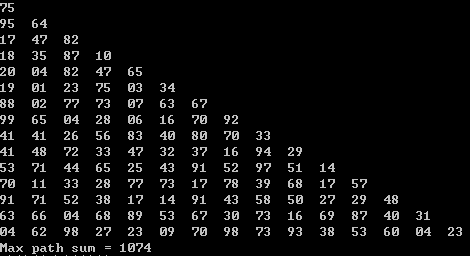
N = 15

75
95 64
17 47 82
18 35 87 10
20 04 82 47 65
19 01 23 75 03 34
88 02 77 73 07 63 67
99 65 04 28 06 16 70 92
41 41 26 56 83 40 80 70 33
41 48 72 33 47 32 37 16 94 29
53 71 44 65 25 43 91 52 97 51 14
70 11 33 28 77 73 17 78 39 68 17 57
91 71 52 38 17 14 91 43 58 50 27 29 48
63 66 04 68 89 53 67 30 73 16 69 87 40 31
04 62 98 27 23 09 70 98 73 93 38 53 60 04 23

输出：