本文主要是介绍【Codeforces Round 333 (Div 2)E】【期望DP概率做法 树状数组转前缀和】Kleofáš and the n-thlon n场比赛m个人获得总名次的期望，希望对大家解决编程问题提供一定的参考价值，需要的开发者们随着小编来一起学习吧！
Kleofáš is participating in an n-thlon - a tournament consisting of n different competitions in n different disciplines (numbered 1 throughn). There are m participants in the n-thlon and each of them participates in all competitions.
In each of these n competitions, the participants are given ranks from 1 to m in such a way that no two participants are given the same rank - in other words, the ranks in each competition form a permutation of numbers from 1 to m. The score of a participant in a competition is equal to his/her rank in it.
The overall score of each participant is computed as the sum of that participant's scores in all competitions.
The overall rank of each participant is equal to 1 + k, where k is the number of participants with strictly smaller overall score.
The n-thlon is over now, but the results haven't been published yet. Kleofáš still remembers his ranks in each particular competition; however, he doesn't remember anything about how well the other participants did. Therefore, Kleofáš would like to know his expected overall rank.
All competitors are equally good at each discipline, so all rankings (permutations of ranks of everyone except Kleofáš) in each competition are equiprobable.
The first line of the input contains two space-separated integers n (1 ≤ n ≤ 100) and m (1 ≤ m ≤ 1000) — the number of competitions and the number of participants respectively.
Then, n lines follow. The i-th of them contains one integer xi (1 ≤ xi ≤ m) — the rank of Kleofáš in the i-th competition.
Output a single real number – the expected overall rank of Kleofáš. Your answer will be considered correct if its relative or absolute error doesn't exceed 10 - 9.
Namely: let's assume that your answer is a, and the answer of the jury is b. The checker program will consider your answer correct, if 
.
4 10 2 1 2 1
1.0000000000000000
5 5 1 2 3 4 5
2.7500000000000000
3 6 2 4 2
1.6799999999999999
In the first sample, Kleofáš has overall score 6. Nobody else can have overall score less than 6 (but it's possible for one other person to have overall score 6 as well), so his overall rank must be 1.
【Codeforces Round 333 (Div 2)E】【期望DP概率做法 树状数组转前缀和】Kleofáš and the n-thlon n场比赛m个人获得总名次的期望 250ms
#include<stdio.h>
#include<string.h>
#include<ctype.h>
#include<math.h>
#include<iostream>
#include<string>
#include<set>
#include<map>
#include<vector>
#include<queue>
#include<bitset>
#include<algorithm>
#include<time.h>
using namespace std;
void fre(){freopen("c://test//input.in","r",stdin);freopen("c://test//output.out","w",stdout);}
#define MS(x,y) memset(x,y,sizeof(x))
#define MC(x,y) memcpy(x,y,sizeof(x))
#define MP(x,y) make_pair(x,y)
#define ls o<<1
#define rs o<<1|1
typedef long long LL;
typedef unsigned long long UL;
typedef unsigned int UI;
template <class T> inline void gmax(T &a,T b){if(b>a)a=b;}
template <class T> inline void gmin(T &a,T b){if(b<a)a=b;}
const int N=100+5,M=1000+5,S=1e5+5,Z=1e9+7,ms63=1061109567;
int n,m,k;
int TOP;
struct BIT
{double f[S];double query(int x){if(++x<=0)return 0;double tmp=0;for(;x;x-=x&-x)tmp+=f[x];return tmp;}void modify(int x,double v){if(++x<=0)return;for(;x<=TOP+1;x+=x&-x)f[x]+=v;}
}bit[2];
int main()
{while(~scanf("%d%d",&n,&m)){if(m==1){puts("1");return 0;}TOP=n*m;double mu=1.0/(m-1);MS(bit,0);bit[0].modify(0,1);int mysco=0;int pre=0,now=1;for(int i=1;i<=n;++i){scanf("%d",&k);mysco+=k;MS(bit[now].f,0);int top=i*m;for(int j=i;j<=top;++j){double rate=(bit[pre].query(j-1)-bit[pre].query(j-m-1))-(bit[pre].query(j-k)-bit[pre].query(j-k-1));bit[now].modify(j,rate*mu);}pre^=1;now^=1;}double ans=bit[pre].query(mysco-1);ans=ans*(m-1)+1;printf("%.12f\n",ans);}return 0;
}
/*
【trick&&吐槽】
1，这道题看似很难，然而只要沉下心来思考，发现问题细细化简后，还是完全可思考的。
2，复杂度看似很高，然而就是可以这样暴力过掉，真是暴力出奇迹的有效印证啊！
3，所以说敢想敢试是ACMer的基本素养>_<~~4，CF多组数据一定要确保完全读入才能用continue。否则是坑自己多次输出，还不如用return 0 TwT
5，树状数组的下标要从1开始不要忘记了。
6，DP的边界处理，再树状数组内部特判一下，写起来就很方便啦。【题意】
这题比较奇怪。题意是说——
有m（1000）个人，他们一同参加了n（100）场比赛。
对于每场比赛，m个人的排名都构成[1,m]的全排列。排名第i的人会得到分数i。
我们知道自己在每场比赛中的名次（设第i场的名次为a[i]），自然，也就知道了自己在每场比赛中的得分。
现在我们想要求，我们最终的比赛名次的期望。最终的比赛名次是指，如果最终时刻有k个人的分数严格比我们小，那么我们的名次便是k+1。现在求得就是这个值的期望。【类型】
期望DP【分析】
设计到期望的题目，并不一定就难得不可做。
我们发现，一共有m个人。除了我们自己之外，还有m-1个人。这m-1个人，每个人的期望得分的状况其实都是相同的。
更加具体而言，我们还有——每个人的可能得分都必然是整数。整数的范围是[n*1,n*m].
如果我们暴力一点，求得每个人对于每个得分x的概率p[x]，我们自己的得分是u的话，那答案就是p[1,u)*(m-1)+1
这个显然成立，因为：一个人比我们分数低的概率*人数=比我们分数低的人数的期望，+1后就是我们名次的期望。于是问题就只剩下——p[x]怎么求？
n和m并不大，甚至说，我们感觉到人数n * 分数的上限n*m 也不过1e7。
于是，我们DP一个人的得分状况——
定义f[i][j]表示"经过了前i次考试，这个人得分为j的概率"。那么有——
f[i][j]=(f[i-1][j-1]+f[i-1][j-2]+...+f[i-1][m]-f[i-1][j-a[i]])/(m-1)这个DP的意义很简单，就是说，我们枚举这个状态，然后看看有哪些状态可以到达它。
而之前的每个可以转移状态，转移到当前状态的当步转移概率都是均等的，都是1/(m-1)。
于是，只要这一个简单的DP，就能实现题目的要求。
然而这个DP的时间复杂度却是O(n * n*m * m) 要爆炸！这里涉及到区间和，于是我们想到用树状数组优化——
把DP的时间复杂度可达O(n*n*m log(m))，然而却依然可以无压力在250ms内AC啦，啦啦啦啦！然而，树状数组还是傻叉做法。
因为这里又不涉及到动态修改，我为什么用树状数组？！
直接搞一个前缀和就好啦！【时间复杂度&&优化】
O(n*n*m log(m)) -> O(n*n*m）*/
【Codeforces Round 333 (Div 2)E】【期望DP概率做法 前缀和】Kleofáš and the n-thlon n场比赛m个人获得总名次的期望 46ms
#include<stdio.h>
#include<string.h>
#include<ctype.h>
#include<math.h>
#include<iostream>
#include<string>
#include<set>
#include<map>
#include<vector>
#include<queue>
#include<bitset>
#include<algorithm>
#include<time.h>
using namespace std;
void fre(){freopen("c://test//input.in","r",stdin);freopen("c://test//output.out","w",stdout);}
#define MS(x,y) memset(x,y,sizeof(x))
#define MC(x,y) memcpy(x,y,sizeof(x))
#define MP(x,y) make_pair(x,y)
#define ls o<<1
#define rs o<<1|1
typedef long long LL;
typedef unsigned long long UL;
typedef unsigned int UI;
template <class T> inline void gmax(T &a,T b){if(b>a)a=b;}
template <class T> inline void gmin(T &a,T b){if(b<a)a=b;}
const int N=100+5,M=1000+5,S=1e5+5,Z=1e9+7,ms63=1061109567;
int n,m,k,pre,now;
double s[2][S+M];
inline double query(int x)
{return x<0?0:s[pre][x];
}
int main()
{while(~scanf("%d%d",&n,&m)){if(m==1){puts("1");return 0;}int top=n*m;double mu=1.0/(m-1);for(int i=0;i<=top;++i)s[0][i]=1;int mysco=0;pre=0;now=1;for(int i=1;i<=n;++i){scanf("%d",&k);mysco+=k;for(int j=0;j<i;++j)s[now][j]=0;for(int j=i;j<=top;++j){double rate=(query(j-1)-query(j-m-1))-(query(j-k)-query(j-k-1));s[now][j]=s[now][j-1]+rate*mu;}pre^=1;now^=1;}double ans=s[pre][mysco-1];ans=ans*(m-1)+1;printf("%.12f\n",ans);}return 0;
}
/*
【trick&&吐槽】
1，这道题看似很难，然而只要沉下心来思考，发现问题细细化简后，还是完全可思考的。
2，复杂度看似很高，然而就是可以这样暴力过掉，真是暴力出奇迹的有效印证啊！
3，所以说敢想敢试是ACMer的基本素养>_<~~4，CF多组数据一定要确保完全读入才能用continue。否则是坑自己多次输出，还不如用return 0 TwT
5，树状数组的下标要从1开始不要忘记了。
6，DP的边界处理，再树状数组内部特判一下，写起来就很方便啦。【题意】
这题比较奇怪。题意是说——
有m（1000）个人，他们一同参加了n（100）场比赛。
对于每场比赛，m个人的排名都构成[1,m]的全排列。排名第i的人会得到分数i。
我们知道自己在每场比赛中的名次（设第i场的名次为a[i]），自然，也就知道了自己在每场比赛中的得分。
现在我们想要求，我们最终的比赛名次的期望。最终的比赛名次是指，如果最终时刻有k个人的分数严格比我们小，那么我们的名次便是k+1。现在求得就是这个值的期望。【类型】
期望DP【分析】
设计到期望的题目，并不一定就难得不可做。
我们发现，一共有m个人。除了我们自己之外，还有m-1个人。这m-1个人，每个人的期望得分的状况其实都是相同的。
更加具体而言，我们还有——每个人的可能得分都必然是整数。整数的范围是[n*1,n*m].
如果我们暴力一点，求得每个人对于每个得分x的概率p[x]，我们自己的得分是u的话，那答案就是p[1,u)*(m-1)+1
这个显然成立，因为：一个人比我们分数低的概率*人数=比我们分数低的人数的期望，+1后就是我们名次的期望。于是问题就只剩下——p[x]怎么求？
n和m并不大，甚至说，我们感觉到人数n * 分数的上限n*m 也不过1e7。
于是，我们DP一个人的得分状况——
定义f[i][j]表示"经过了前i次考试，这个人得分为j的概率"。那么有——
f[i][j]=(f[i-1][j-1]+f[i-1][j-2]+...+f[i-1][m]-f[i-1][j-a[i]])/(m-1)这个DP的意义很简单，就是说，我们枚举这个状态，然后看看有哪些状态可以到达它。
而之前的每个可以转移状态，转移到当前状态的当步转移概率都是均等的，都是1/(m-1)。
于是，只要这一个简单的DP，就能实现题目的要求。
然而这个DP的时间复杂度却是O(n * n*m * m) 要爆炸！这里涉及到区间和，于是我们想到用树状数组优化——
把DP的时间复杂度可达O(n*n*m log(m))，然而却依然可以无压力在250ms内AC啦，啦啦啦啦！然而，树状数组还是傻叉做法。
因为这里又不涉及到动态修改，我为什么用树状数组？！
直接搞一个前缀和s[2][S]就好啦！【时间复杂度&&优化】
O(n*n*m log(m)) -> O(n*n*m）*/这篇关于【Codeforces Round 333 (Div 2)E】【期望DP概率做法 树状数组转前缀和】Kleofáš and the n-thlon n场比赛m个人获得总名次的期望的文章就介绍到这儿，希望我们推荐的文章对编程师们有所帮助！
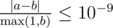