stein专题
复分析——第3章——亚纯函数和对数(E.M. Stein R. Shakarchi)
第3章 亚纯函数和对数 (Meromorphic Functions and the Logarithm) One knows that the differential calculus, which has contributed so much to the progress of analysis, is founded on the consideration of diffe
HDU2028 Lowest Common Multiple Plus【stein算法】【水题】
Lowest Common Multiple Plus Time Limit: 2000/1000 MS (Java/Others) Memory Limit: 65536/32768 K (Java/Others) Total Submission(s): 36800 Accepted Submission(s): 15116 Probl
复分析——第2章——Cauchy定理及其应用(E.M. Stein R. Shakarchi)
第2章 Cauchy定理及其应用 The solution of a large number of problems can be reduced, in the last analysis, to the evaluation of definite integrals; thus mathematicians have been much occupied with this ta
centos7安装与配置OpenStack-Zun组件(Stein版)
文章目录 一、基本环境参数二、controller节点zun安装2.1 创建数据库2.2 创建openstack用户、服务、端点2.3 安装、启动zun服务2.3.1 创建用户、组2.3.2 创建目录2.3.3 安装zun2.3.4 生成配置文件并配置2.3.5 填充数据库2.3.6 创建启动文件2.3.7启动服务 2.4 Etcd安装与配置2.4.1 Etcd安装2.4.2 配置Etcd2
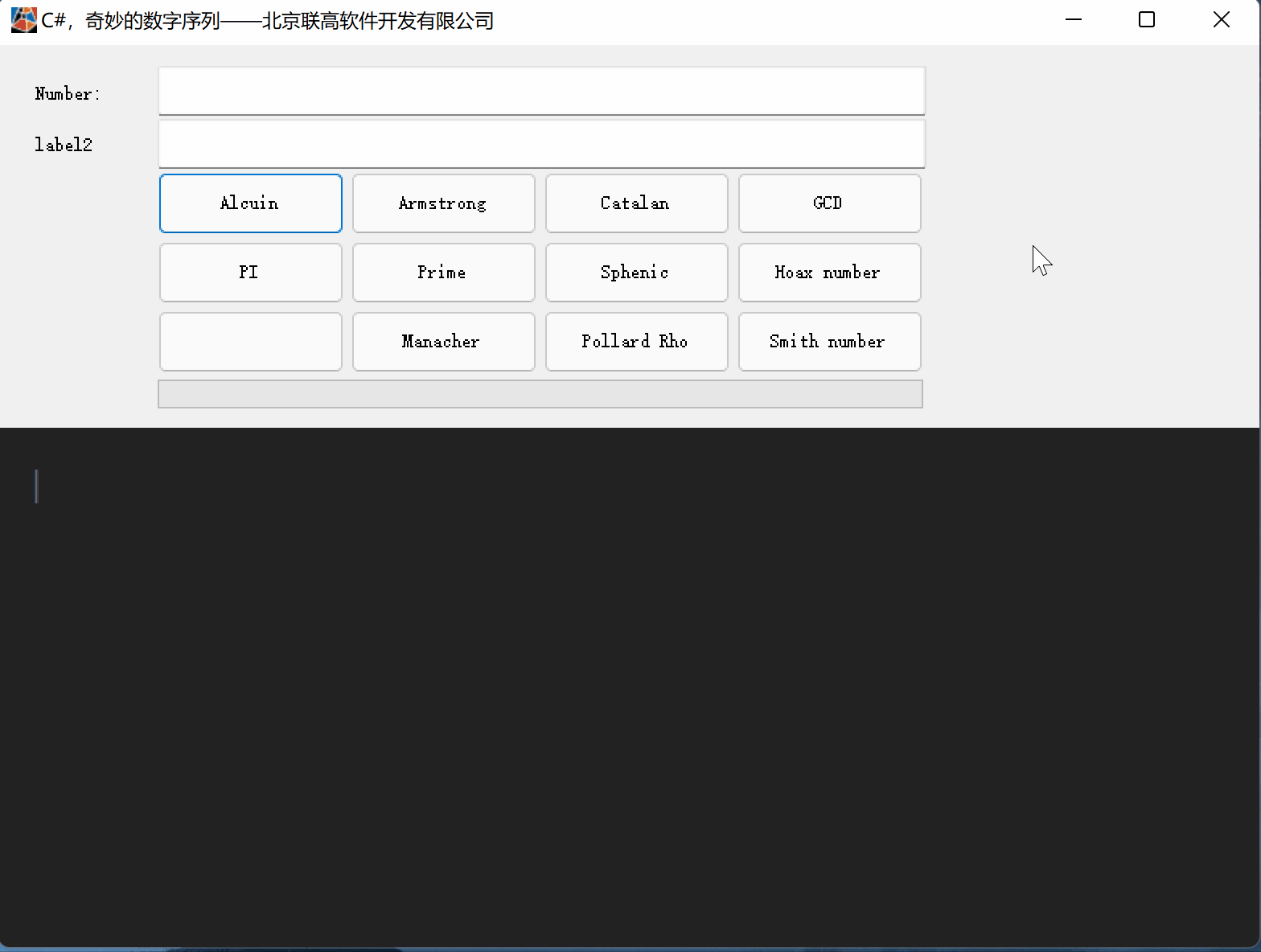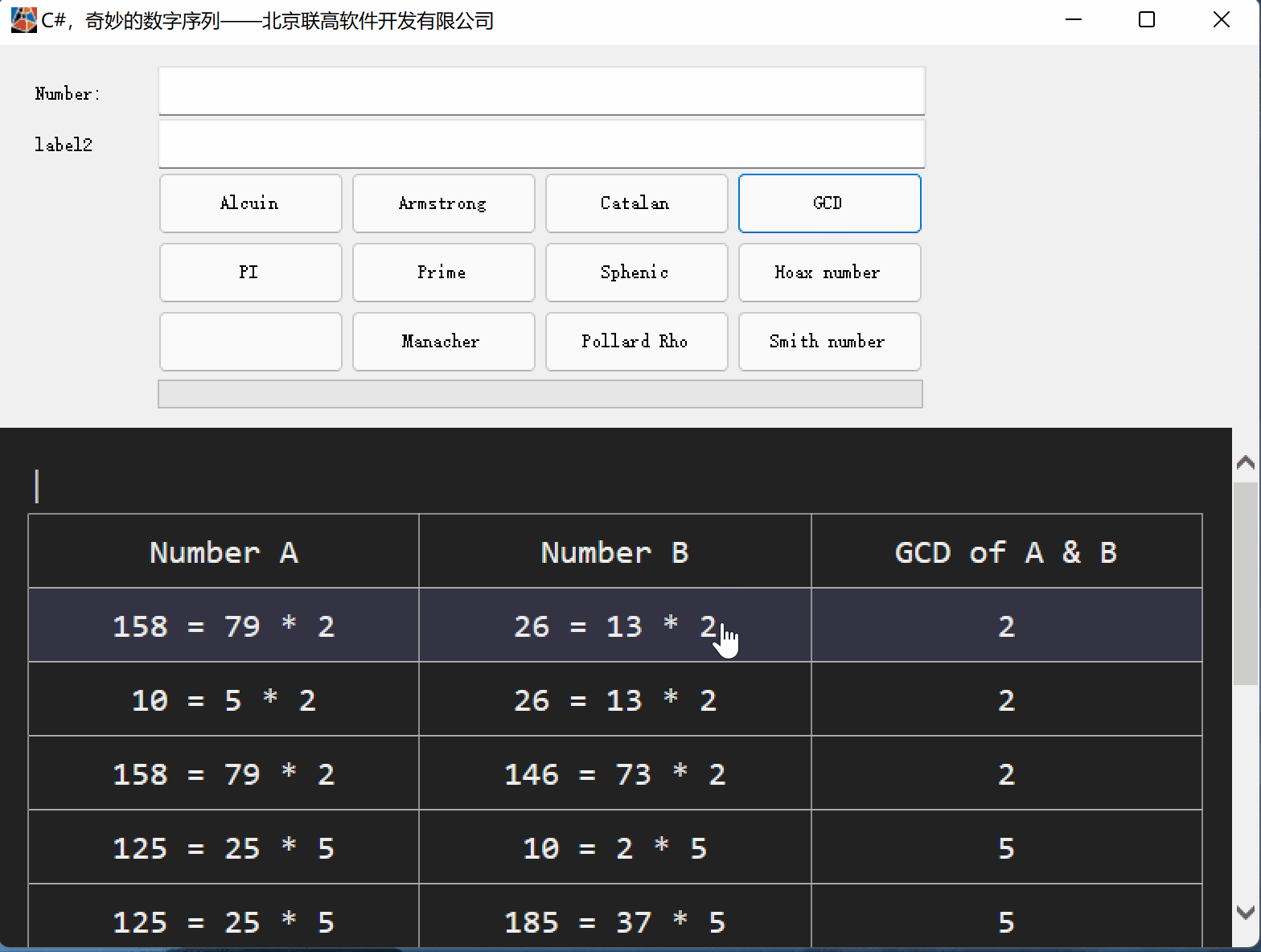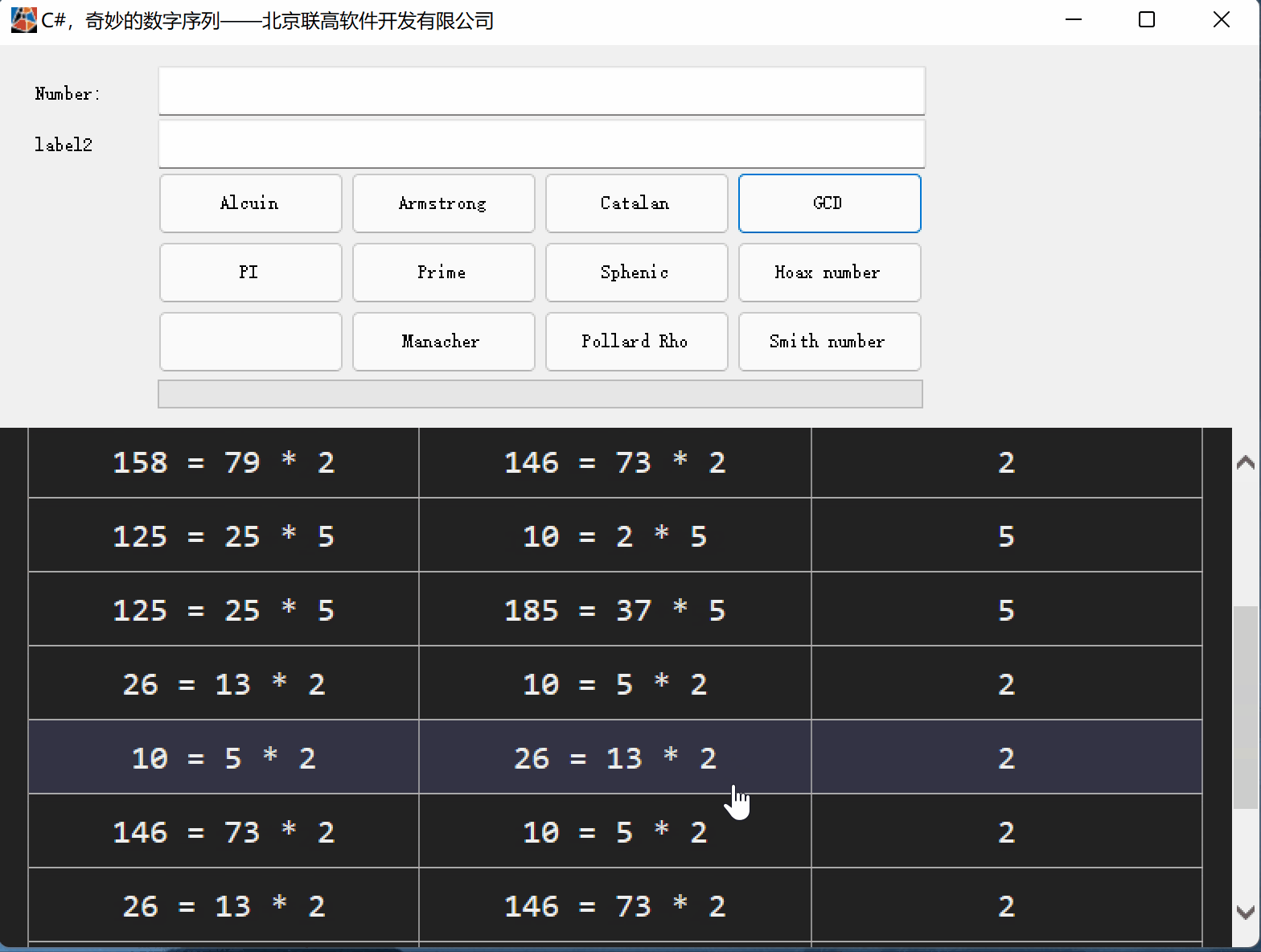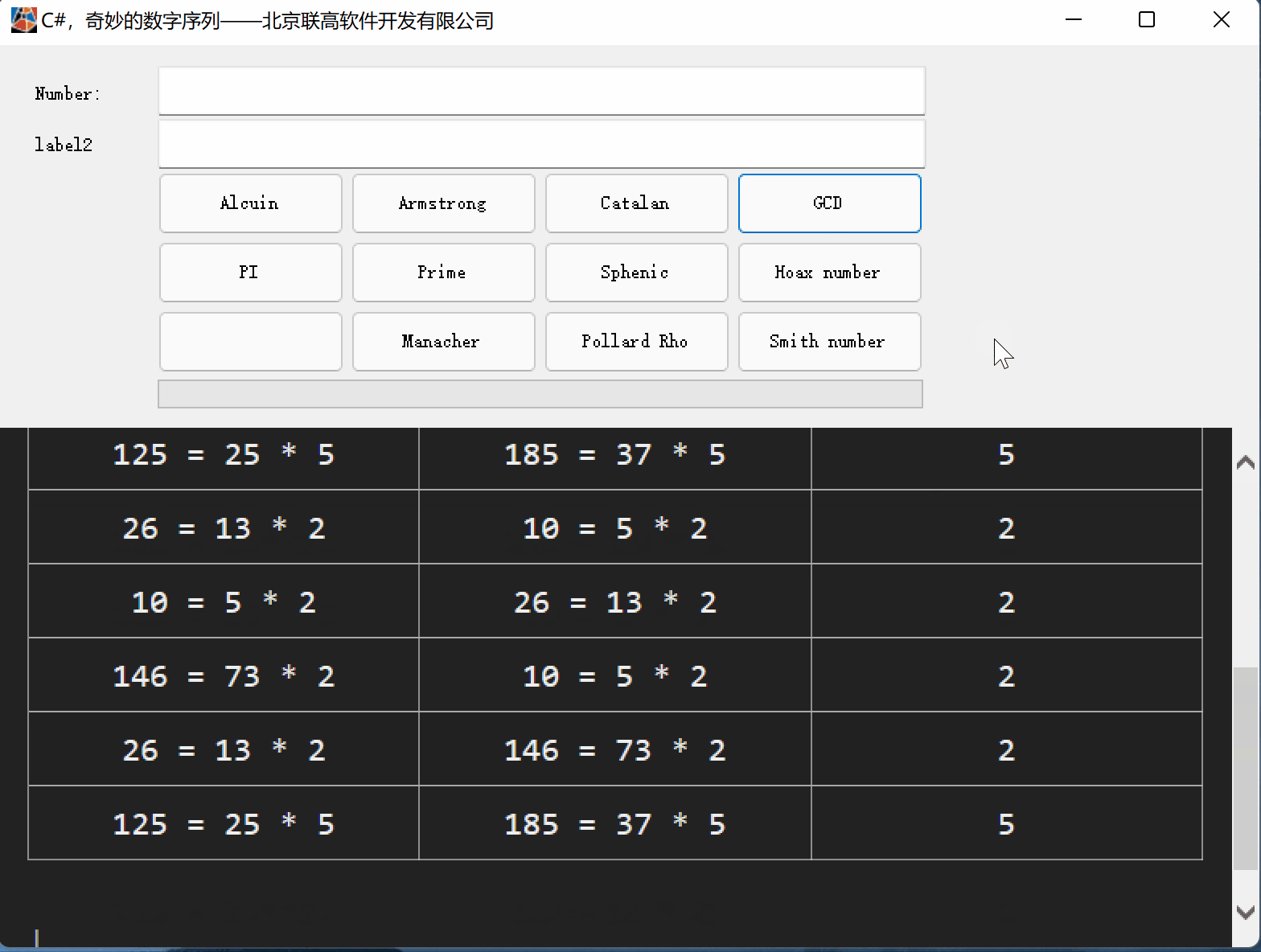
C#,最大公约数(GCD)斯坦因(Stein)算法的源代码
Stein 算法或二进制 GCD 算法是计算两个非负整数的最大公约数的算法。 Stein 的算法用算术移位、比较和减法代替除法。 using System; using System.Text; namespace Legalsoft.Truffer.Algorithm { public static class GCD { /// <summa
复分析——第1章——复分析准备知识(E.M. Stein R. Shakarchi)
第一章 复分析准备知识 (Preliminaries to Complex Analysis) The sweeping development of mathematics during the last two centuries is due in large part to the introduction of complex numbers; paradox
Fourier分析导论——第7章——有限Fourier分析(E.M. Stein R. Shakarchi)
第7章 有限Fourier分析 This past year has seen the birth, or rather the re- birth, of an exciting revolution in computing Fourier transforms. A class of algorithms known as the fast Fourier transform or
Fourier分析导论——第3章——Fourier级数的收敛性(E.M. Stein R. Shakarchi)
第 3 章 Fourier级数的收敛性(Convergence of Fourier Series) The sine and cosine series, by which one can represent an arbitrary function in a given interval, enjoy among other remarkable properties that of
Fourier分析导论——第2章——Fourier级数的基本属性(E.M. Stein R. Shakarchi)
第 2 章 Fourier级数的基本属性(Basic Properties of Fourier Series) Nearly fifty years had passed without any progress on the question of analytic representation of an arbitrary function, when an assertion o