保研机试之【动态规划】

本文主要是介绍保研机试之【动态规划】，希望对大家解决编程问题提供一定的参考价值，需要的开发者们随着小编来一起学习吧！

本文为博客：动态规划解题套路框架 | labuladong 的算法笔记 的笔记

前言

动态规划问题的一般形式就是求最值，求解动态规划的核心问题是穷举。动态规划三要素为：最优子结构、重叠子问题、状态转移方程。首先要判断，该问题是否具有重复子问题，如果有则可以用动态规划求解。动态规划问题存在「重叠子问题」，如果暴力穷举的话效率会很低，所以需要你使用「备忘录」或者「DP table」来优化穷举过程；动态规划只有列出正确的「状态转移方程」，才能正确地穷举。

理解重复子问题：https://leetcode.cn/problems/fibonacci-number/description/

递归算法的时间复杂度怎么计算？就是用子问题个数乘以解决一个子问题需要的时间。

暴力求解（注意树的形状）

​

​

会存在很多计算重复的情况，这也是为什么不采用备忘录会导致效率低下，时间复杂度为O()，因为子问题个数为，解决一个子问题需要的时间（加法）为1。

带备忘录的递归解法（注意树的剪枝）

​

最后就剩类似红色方框的形状：

​

时间复杂度为O(n)，因为子问题个数为n，解决一个子问题需要的时间（加法）为1。

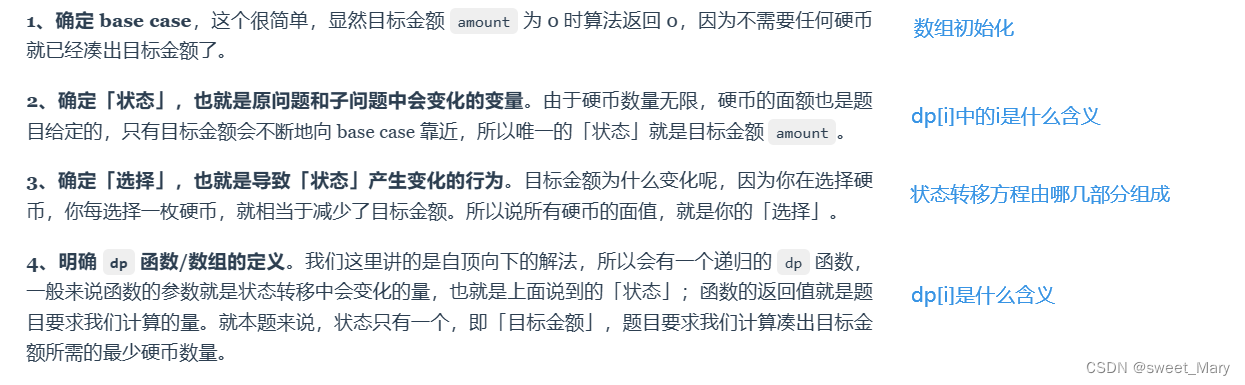
dp 数组的迭代（递推）解法

​

​

理解状态转移方程：动态规划解题套路框架 | labuladong 的算法笔记

暴力求解

class Solution {
public:int coinChange(vector<int>& coins, int amount) {if(amount==0){return 0;}if(amount<0){return -1;}int ans=INT_MAX;for(int i:coins){int temp=coinChange(coins,amount-i);if(temp==-1){continue;}ans=min(ans,temp+1);}if(ans==INT_MAX){return -1;}else{return ans;}}
};

直接超时：

带备忘录的递归

class Solution {
public:unordered_map<int,int> hmap;int coinChange(vector<int>& coins, int amount) {if(amount==0){return 0;}if(amount<0){return -1;}if(hmap.find(amount)!=hmap.end()){return hmap[amount];}int ans=INT_MAX;for(int i:coins){int temp;if(hmap.find(amount-i)!=hmap.end()){temp=hmap[amount-i];}else{temp=coinChange(coins,amount-i);if(temp==-1){continue;}else{hmap[amount-i]=temp;}}ans=min(ans,temp+1);}if(ans==INT_MAX){return -1;}else{return ans;}}
};

但是！

dp 数组的迭代解法

class Solution {
public:int coinChange(vector<int>& coins, int amount) {vector<int> g(10010,amount+1);g[0]=0;for(int i=1;i<=amount;i++){for(auto j:coins){if(i-j>=0){g[i]=min(g[i],g[i-j]+1);}}}if(g[amount]>amount){return -1;}return g[amount];}
};

这篇关于保研机试之【动态规划】的文章就介绍到这儿，希望我们推荐的文章对编程师们有所帮助！

---