本文主要是介绍BD202311夏日漫步(最少步数,BFS或者 Dijstra),希望对大家解决编程问题提供一定的参考价值,需要的开发者们随着小编来一起学习吧!
本题链接:码蹄集
题目:
夏日夜晚,小度看着庭院中长长的走廊,萌发出想要在上面散步的欲望,小度注意到月光透过树荫落在地砖上,并且由于树荫的遮蔽度不通,所以月光的亮度不同,为了直观地看到每个格子的亮度,小度用了一些自然数来表示它们的亮度。亮度越高则数字越大,亮度相同的数字相同。
走廊是只有一行地砖的直走廊。上面一共有 nn 个格子,每个格子都被小度给予了一个数字 a_iai 来表示它的亮度。
小度现在站在 11 号格子,想要去到 nn 号格子。小度可以正向或反向移动到相邻的格子,每次需要花费 11 的体力。
同时小度还有瞬移的能力,其可以花费 11 的体力来瞬移到与当前格子亮度相同的格子上。而且由于小度视野有限,只能瞬移到在当前格子后的第一次亮度相同的格子上。这也意味着不能反向瞬移。
小度想知道,到达 nn 号格子需要花费的最小体力是多少。以此制定一个最优秀的散步方案。

样例:
|
| 3 |
思路:
根据题意,我们将小度移动方向,看成一块图,相邻格子之间作为路线,连接线,我们就可以很方便的,根据 BFS 或者 Dijkstra 来获取最少步数的操作了。
注意!以后遇到最少步数,最少操作这些关键字,思路得要联想到 BFS 和 Dijkstra 这些最少操作数方面的算法啦!
Dijstra代码详解如下:
#include <iostream>
#include <vector>
#include <queue>
#include <cstring>
#include <algorithm>
#include <unordered_map>
#include <unordered_set>
#define endl '\n'
#define x first
#define y second
#define int long long
#define YES puts("YES")
#define NO puts("NO")
#define INF 0x3f3f3f3f3f3f
#define umap unordered_map
#define uset unordered_set
#define All(x) x.begin(),x.end()
#pragma GCC optimize(3,"Ofast","inline")
#define IOS std::ios::sync_with_stdio(false),cin.tie(0), cout.tie(0)
using namespace std;
const int N = 2e5 + 10;
inline void solve();signed main()
{
// freopen("a.txt", "r", stdin);IOS;int _t = 1;
// cin >> _t;while (_t--){solve();}return 0;
}int n,ans;using PII = pair<int,int>;
umap<int,vector<int>>g,cnt; // g 记录连接线 cnt 记录可瞬移的点// Dijkstra 最短路求值
inline void Dijkstra()
{vector<int>dist(n + 1,INF);vector<bool>st(n + 1,false);dist[0] = 0;priority_queue<PII,vector<PII>,greater<PII>>q;q.emplace(PII(0,0));while(q.size()){PII now = q.top();q.pop();if(st[now.y])continue;st[now.y] = true;int a = now.y,dis = now.x;for(int i = 0;i < (int)g[a].size();++i){int j = g[a][i];if(dist[j] > dis + 1) dist[j] = dis + 1;q.emplace(PII(dist[j],j));}}ans = dist[n - 1];
}inline void solve()
{cin >> n;uset<int>node; // 记录 所有点for(int i = 0,x;i < n;++i){cin >> x;node.emplace(x);cnt[x].emplace_back(i); // 记录可瞬移的点if(i - 1 >= 0){// 小度可以正向或反向移动到相邻的格子g[i].emplace_back(i - 1);g[i - 1].emplace_back(i);}}for(auto &i:node){for(int j = 0;j + 1 < (int)cnt[i].size();++j){// 不能反向瞬移,只能瞬移到在当前格子后的第一次亮度相同的格子上// 所以我们只连接相邻可瞬移的g[cnt[i][j]].emplace_back(cnt[i][j + 1]);}}Dijkstra();cout << ans << endl;
}最后提交: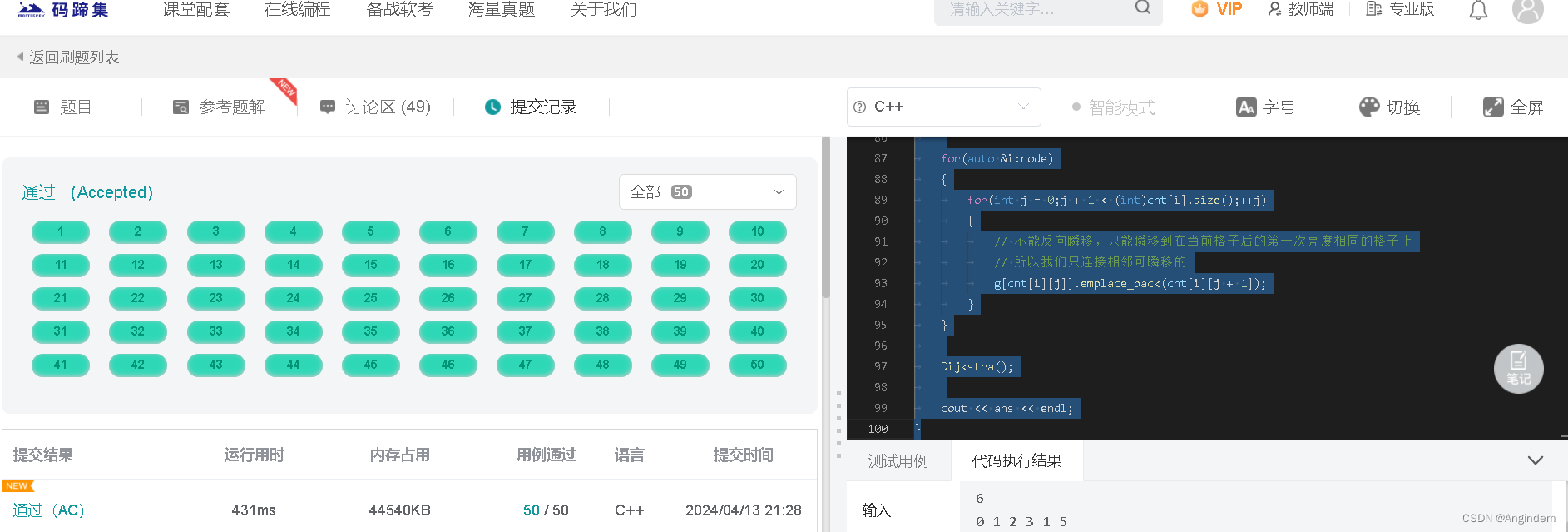
BFS代码详解如下:
#include <iostream>
#include <vector>
#include <queue>
#include <cstring>
#include <algorithm>
#include <unordered_map>
#include <unordered_set>
#define endl '\n'
#define x first
#define y second
#define int long long
#define YES puts("YES")
#define NO puts("NO")
#define INF 0x3f3f3f3f3f3f
#define umap unordered_map
#define uset unordered_set
#define All(x) x.begin(),x.end()
#pragma GCC optimize(3,"Ofast","inline")
#define IOS std::ios::sync_with_stdio(false),cin.tie(0), cout.tie(0)
using namespace std;
const int N = 2e5 + 10;
inline void solve();signed main()
{
// freopen("a.txt", "r", stdin);IOS;int _t = 1;
// cin >> _t;while (_t--){solve();}return 0;
}int n,ans;using PII = pair<int,int>;
umap<int,vector<int>>g,cnt; // g 记录连接线 cnt 记录可瞬移的点// BFS 求最短路
inline int BFS()
{vector<bool>st(N,false);queue<int>q;int step = 0;q.emplace(0);while(q.size()){int sz = q.size();while(sz--){int now = q.front();q.pop();st[now] = true;if(now == n - 1) return step;for(int i = 0;i < (int)g[now].size();++i){int j = g[now][i];if(!st[j]){st[j] = true;q.emplace(j);}}}++step;}return 0;
}inline void solve()
{cin >> n;uset<int>node; // 记录 所有点for(int i = 0,x;i < n;++i){cin >> x;node.emplace(x);cnt[x].emplace_back(i); // 记录可瞬移的点if(i - 1 >= 0){// 小度可以正向或反向移动到相邻的格子g[i].emplace_back(i - 1);g[i - 1].emplace_back(i);}}for(auto &i:node){for(int j = 0;j + 1 < (int)cnt[i].size();++j){// 不能反向瞬移,只能瞬移到在当前格子后的第一次亮度相同的格子上// 所以我们只连接相邻可瞬移的g[cnt[i][j]].emplace_back(cnt[i][j + 1]);}}int ans = BFS();cout << ans << endl;
}最后提交:
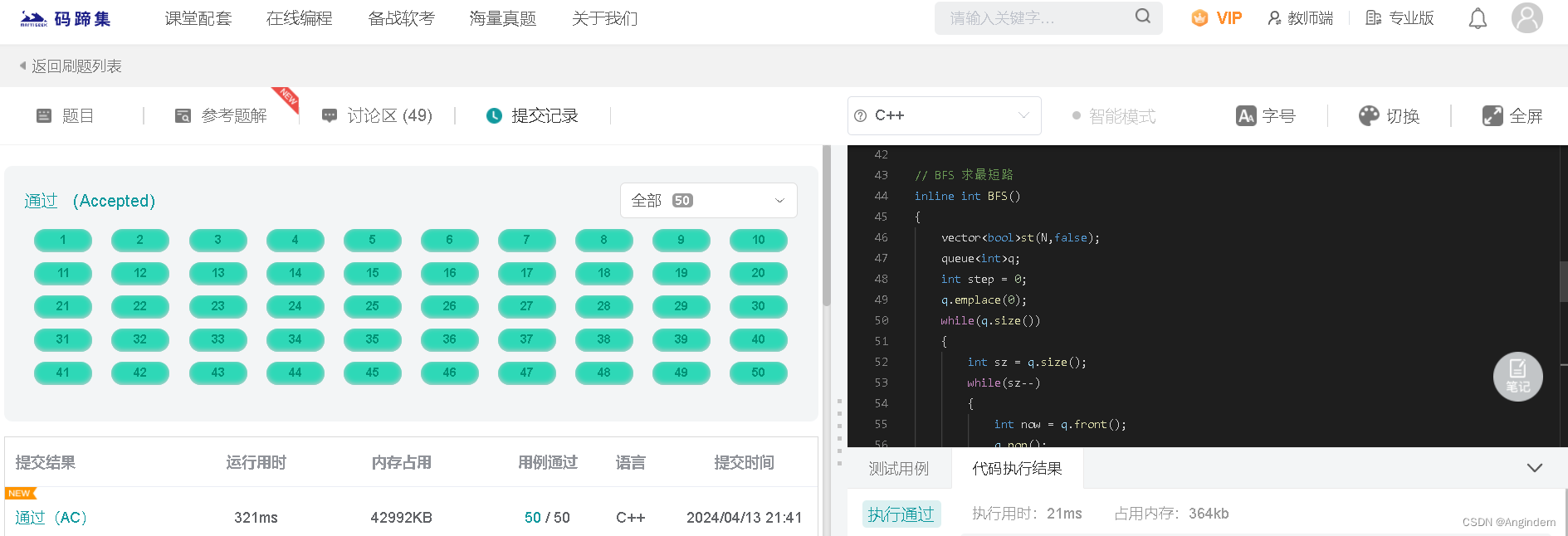
这篇关于BD202311夏日漫步(最少步数,BFS或者 Dijstra)的文章就介绍到这儿,希望我们推荐的文章对编程师们有所帮助!



