本文主要是介绍Hilditch 细化算法,希望对大家解决编程问题提供一定的参考价值,需要的开发者们随着小编来一起学习吧!
Hilditch 细化算法是经典的二值图像细化算法,然而,在网上却很难找到一个详细、正确的介绍和实现。可以找到一辆个 Hilditch 算法的C实现,但缺乏注释,代码可读性也很差。在期刊网上找到几篇论文,提及了Hilditch 算法,结果一篇说的罗哩罗嗦根本看不懂,另一篇说的说的易懂,却是错误的!拿来主义是行不通了,于是只好结合着这几个论文和代码,从头写 Hilditch 细化算法。
假设像素p的3×3邻域结构为:
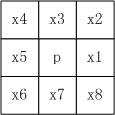
Hilditch 细化算法的步骤为:
对图像从左向右从上向下迭代每个像素,是为一个迭代周期。在每个迭代周期中,对于每一个像素p,如果它同时满足6个条件,则标记它。在当前迭代周期结束时,则把所有标记的像素的值设为背景值。如果某次迭代周期中不存在标记点(即满足6个条件的像素),则算法结束。假设背景值为0,前景值为1,则:
6个条件为:
(I):p 为1,即p不是背景;
(2):x1,x3,x5,x7不全部为1(否则把p标记删除,图像空心了);
(3):x1~x8 中,至少有2个为1(若只有1个为1,则是线段的端点。若没有为1的,则为孤立点);
(4):p的8连通联结数为1;
联结数指在像素p的3*3邻域中,和p连接的图形分量的个数:

上图中,左图的4连通联结数是2,8连通联结数是1,而右图的4联通联结数和8联通联结数都是2。
4连通联结数计算公式是:
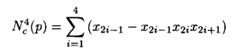
8连通联结数计算公式是:
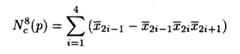 其中,
其中,
![]()
至于公式怎么来的就不管了,直接用就行了。
(5)假设x3已经标记删除,那么当x3为0时,p的8联通联结数为1;
(6)假设x5已经标记删除,那么当x5为0时,p的8联通联结数为1。
代码如下:
======
在程序中,我使用的是这样的邻域编码:
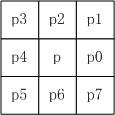
为了方便计算联结数,以0作为前景,1作为背景。程序如下(完整程序见:http://smartimage.googlecode.com/svn/trunk/src/Orc.SmartImage.Common/UnmanagedImage/ImageU8.cs):
/// <summary>
/// 计算八联结的联结数,计算公式为:
/// (p6 - p6*p7*p0) + sigma(pk - pk*p(k+1)*p(k+2)), k = {0,2,4)
/// </summary>
/// <param name="list"></param>
/// <returns></returns>
private unsafe Int32 DetectConnectivity(Int32* list)
{
Int32 count = list[6] - list[6] * list[7] * list[0];
count += list[0] - list[0] * list[1] * list[2];
count += list[2] - list[2] * list[3] * list[4];
count += list[4] - list[4] * list[5] * list[6];
return count;
}private unsafe void FillNeighbors(Byte* p, Int32* list, Int32 width, Byte foreground = 255)
{
// list 存储的是补集,即前景点为0,背景点为1,以方便联结数的计算list[0] = p[1] == foreground ? 0 : 1;
list[1] = p[1 - width] == foreground ? 0 : 1;
list[2] = p[-width] == foreground ? 0 : 1;
list[3] = p[-1 - width] == foreground ? 0 : 1;
list[4] = p[-1] == foreground ? 0 : 1;
list[5] = p[-1 + width] == foreground ? 0 : 1;
list[6] = p[width] == foreground ? 0 : 1;
list[7] = p[1 + width] == foreground ? 0 : 1;
}/// <summary>
/// 使用 hilditch 算法进行细化
/// </summary>
public unsafe void Thinning(Byte foreground = 255)
{
Byte* start = this.Start;
Int32 width = this.Width;
Int32 height = this.Height;
Int32* list = stackalloc Int32[8];
Byte background = (Byte)(255 - foreground);
Int32 length = this.Length;using (ImageU8 mask = new ImageU8(this.Width, this.Height))
{
mask.Fill(0);Boolean loop = true;
while (loop == true)
{
loop = false;
for (Int32 r = 1; r < height - 1; r++)
{
for (Int32 c = 1; c < width - 1; c++)
{
Byte* p = start + r * width + c;// 条件1:p 必须是前景点
if (*p != foreground) continue;// p3 p2 p1
// p4 p p0
// p5 p6 p7
// list 存储的是补集,即前景点为0,背景点为1,以方便联结数的计算
FillNeighbors(p, list, width, foreground);// 条件2:p0,p2,p4,p6 不皆为前景点
if (list[0] == 0 && list[2] == 0 && list[4] == 0 && list[6] == 0)
continue;// 条件3: p0~p7至少两个是前景点
Int32 count = 0;
for (int i = 0; i < 8; i++)
{
count += list[i];
}if (count > 6) continue;
// 条件4:联结数等于1
if (DetectConnectivity(list) != 1) continue;// 条件5: 假设p2已标记删除,则令p2为背景,不改变p的联结数
if (mask[r - 1, c] == 1)
{
list[2] = 1;
if (DetectConnectivity(list) != 1)
continue;
list[2] = 0;
}// 条件6: 假设p4已标记删除,则令p4为背景,不改变p的联结数
if (mask[r, c - 1] == 1)
{
list[4] = 1;
if (DetectConnectivity(list) != 1)
continue;
}
mask[r, c] = 1; // 标记删除
loop = true;
}
}for (int i = 0; i < length; i++)
{
if (mask[i] == 1)
{
this[i] = background;
}
}
}
}
}
======
我的代码如下:
void HilditchThinning(int w,int h,BYTE *imgBuf)< xmlnamespace prefix ="o" ns ="urn:schemas-microsoft-com:office:office" />
{
// p3 p2 p1
// 8近邻 p4 p p0
// p5 p6 p7
int neighbor[8];
BYTE *mask=new BYTE[w*h];
memset(mask,0,w*h);
BOOL loop=TRUE;
int x,y,k,index;
while(loop)
{
loop=FALSE;
loopNum++;
for(y=0;y<h;y++)
{
for(x=0;x<w;x++)
{
index=y*w+x; ;
//条件1:p必须是前景点
if(imgBuf[index]==0 ) continue;
neighbor[0]=x+1<w ? imgBuf[y*w+x+1] : 0;
neighbor[1]=y-1>0&&x+1<w ? imgBuf[(y-1)*w+x+1] : 0;
neighbor[2]=y-1>0 ? imgBuf[(y-1)*w+x] : 0;
neighbor[3]=y-1>0&&x-1<0 ? imgBuf[(y-1)*w+x-1] : 0;
neighbor[4]=x-1>0 ? imgBuf[y*w+x-1] : 0;
neighbor[5]=x-1>0&&y+1<h ? imgBuf[(y+1)*w+x-1] : 0;
neighbor[6]=y+1<h ? imgBuf[(y+1)*w+x] : 0;
neighbor[7]=y+1<h&&x+1<w ? imgBuf[(y+1)*w+x+1] : 0;
//条件2:p0,p2,p4,p6不全为前景色(否则把点p删了,图像空心)
if(neighbor[0]&&neighbor[2]&&neighbor[4]&&neighbor[6])
continue;
//条件3:p0~p7中,至少有个为前景色
//(若只有一个为,则为端点,若没有为的,则为孤立点)
int count=0;
for(int i=0;i<8;i++)
{
if(neighbor[i]==255)
count++;
}
if(count<2)
{
continue;
}
//条件4:p的八近邻连接数必须为1
if(Get8Connectivity(neighbor)!=1) continue;
//条件5:若p2已经被标记删除,则当p2为背景色时,P的连接数仍需为1
k=(y-1)*w+x;
if(y-1>0 && mask[k]==1)
{
imgBuf[k]=0;
if(Get8Connectivity(neighbor)!=1) continue;
imgBuf[k]=1;
}
//条件6:若p4已经被标记删除,则当p4为背景色时,P的连接数仍需为1
k=y*w+x-1;
if(x-1>0 && mask[k]==1)
{
imgBuf[k]=0;
if(Get8Connectivity(neighbor)!=1) continue;
imgBuf[k]=1;
}
//标记删除
mask[w*y+x]=1;
loop=TRUE;
}
}
//将标记删除的点置为背景色
for(y=0;y<h;y++)
{
for(x=0;x<w;x++)
{
k=y*w+x;
if(mask[k]==1) imgBuf[k]=0;
}
}
}
}
// p3 p2 p1
//*************计算近邻的连接数 p4 p p0
// p5 p6 p7
int Get8Connectivity(int* neighbor)
{
//计算补集x^=1-x;
for(int i=0;i<8;i++)
{
neighbor[i]=neighbor[i]==0?1:0;
}
int count= neighbor[0]-(neighbor[0]&neighbor[1]&neighbor[2]);
count+= neighbor[2]-(neighbor[2]&neighbor[3]&neighbor[4]);
count+= neighbor[4]-(neighbor[4]&neighbor[5]&neighbor[6]);
count+= neighbor[6]-(neighbor[6]&neighbor[7]&neighbor[0]);
return count;
}
这个细化算法,细化后会产生毛刺
这篇关于Hilditch 细化算法的文章就介绍到这儿,希望我们推荐的文章对编程师们有所帮助!








