Verilog练习：HDLBits笔记10

三、Circuits

Combinational logic-Karnaugh Map to Circuit

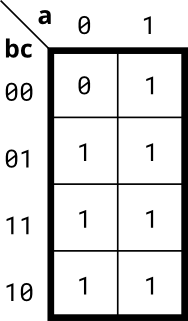
1、3-variable

Problem Statement：

mplement the circuit described by the Karnaugh map below.

module top_module(input a,input b,input c,output out  
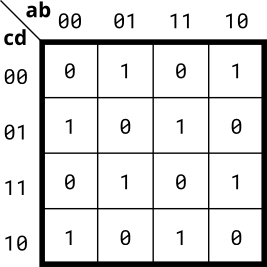
);assign out = a | b | c;endmodule

2、4-variable 

Problem Statement：

Implement the circuit described by the Karnaugh map below.

module top_module(input a,input b,input c,input d,output out  
); assign out = ~a & ~d | ~b & ~c | b & c & d | a & ~b & d | ~a & b & c;endmodule

 3、4-variable 

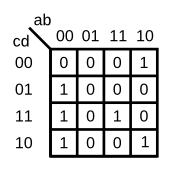
Problem Statement：

Implement the circuit described by the Karnaugh map below.

module top_module(input a,input b,input c,input d,output out  
);assign out = a | ~b & c;endmodule

  4、4-variable 

Problem Statement：

Implement the circuit described by the Karnaugh map below.

module top_module(input a,input b,input c,input d,output out  
);assign out = a^b^c^d;endmodule

 5、Minimum SOP and POS

Problem Statement：

A single-output digital system with four inputs (a,b,c,d) generates a logic-1 when 2, 7, or 15 appears on the inputs, and a logic-0 when 0, 1, 4, 5, 6, 9, 10, 13, or 14 appears. The input conditions for the numbers 3, 8, 11, and 12 never occur in this system. For example, 7 corresponds to a,b,c,d being set to 0,1,1,1, respectively.

Determine the output out_sop in minimum SOP form, and the output out_pos in minimum POS form.

module top_module (input a,input b,input c,input d,output out_sop,output out_pos
); assign out_sop = c & d | ~a & ~ b & c;assign out_pos = ~((~c | ~d) & (a | b | ~c));endmodule

 6、Karnaugh map

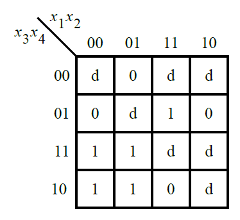
Problem Statement：

Consider the function f shown in the Karnaugh map below.

Implement this function. d is don't-care, which means you may choose to output whatever value is convenient.

module top_module (input [4:1] x, output f 
);assign f = ~x[1] & x[3] | x[2] & x[4];endmodule

7、Karnaugh map

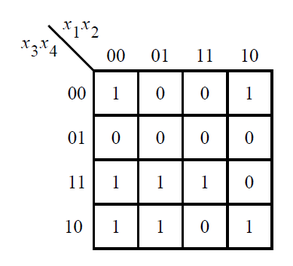
Problem Statement：

Consider the function f shown in the Karnaugh map below. Implement this function.

(The original exam question asked for simplified SOP and POS forms of the function.)

module top_module (input [4:1] x,output f
); assign f = ~x[1]&x[3] | ~x[2]&~x[4] | x[2]&x[3]&x[4];endmodule

8、K-map implemented with a multiplexer

Problem Statement：

For the following Karnaugh map, give the circuit implementation using one 4-to-1 multiplexer and as many 2-to-1 multiplexers as required, but using as few as possible. You are not allowed to use any other logic gate and you must use a and b as the multiplexer selector inputs, as shown on the 4-to-1 multiplexer below.

You are implementing just the portion labelled top_module, such that the entire circuit (including the 4-to-1 mux) implements the K-map.

module top_module (input c,input d,output [3:0] mux_in
); always @(*) begincase({c,d})2'b00:mux_in = 4'b0100;2'b01:mux_in = 4'b0001;2'b11:mux_in = 4'b1001;2'b10:mux_in = 4'b0101;default:;endcaseendendmodule