本文主要是介绍Total Variation Denosing,希望对大家解决编程问题提供一定的参考价值,需要的开发者们随着小编来一起学习吧!
(来自wiki total variation denoising)
In signal processing, Total Variation denoising, also known as total variation regularization is a process, most often used in digital image processing that has applications in noise removal. It is based on the principle that signals with excessive and possibly spurious detail have high total variation, that is, the integral of the absolute gradient of the signal is high. According to this principle, reducing the total variation of the signal subject to it being a close match to the original signal, removes unwanted detail whilst preserving important details such as edges.
Total Variation,顾名思义,即是信号的变化程度之和。对一维信号yn,TV项为
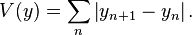
总体来讲,由于噪音信号(图像)相邻信号间不平滑,随机变化较大,故total variation比较大,而平滑信号则TV项比较小。通过minimize total variation,可以去除噪音,平滑信号。TV相比median filter和linear smooth的优点是不像这两个一样,在去除噪音的时候同时也会把边缘smooth了,median filter和linear smooth是不会区分边缘和噪音的。而total variation似乎只对噪音比较敏感,而能保留一定得边缘信息(why?)。
如果给定一个带随机噪音的信号 xn, 想找一个接近xn的信号yn, 但具有更小的total variation. 可以用下面的式子来衡量:
the sum of square errors:
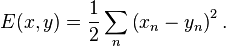
最后整个问题就变为求下式最小值:
E(x,y) + λV(y)
一共是两个分量,前者是fidelity constraint,后者为TV项。λ用来调整权重。可用极端思想来考虑:若λ为0,则TV项完全没有起到惩罚作用,求得的信号x等于原信号y;反之,若λ-->无穷,则完全是TV惩罚项起主导作用,求得的信号x会尽可能的满足TV项很小,但fidelity就会很差,可能偏离原先信号很远,甚至连原信号x的基本structure也无法体现,就没法取得消除噪音的效果了。这个式子对y进行求求偏导,可以构造出拉格朗日方程来求解。
考虑2维信号的情况(e.g. 图像)
设y是一幅图像,total variation norm -->
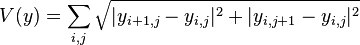
或者也可以求解下面方程:
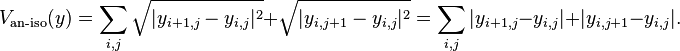
同理,最后整个问题就变为求下式最小值:
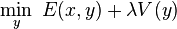
这篇关于Total Variation Denosing的文章就介绍到这儿,希望我们推荐的文章对编程师们有所帮助!