leetcode - 935. Knight Dialer

本文主要是介绍leetcode - 935. Knight Dialer，希望对大家解决编程问题提供一定的参考价值，需要的开发者们随着小编来一起学习吧！

Description

The chess knight has a unique movement, it may move two squares vertically and one square horizontally, or two squares horizontally and one square vertically (with both forming the shape of an L). The possible movements of chess knight are shown in this diagaram:

A chess knight can move as indicated in the chess diagram below:

We have a chess knight and a phone pad as shown below, the knight can only stand on a numeric cell (i.e. blue cell).

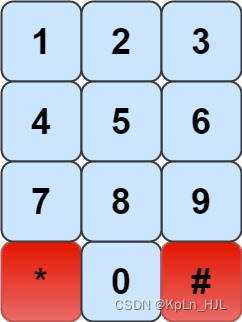
Given an integer n, return how many distinct phone numbers of length n we can dial.

You are allowed to place the knight on any numeric cell initially and then you should perform n - 1 jumps to dial a number of length n. All jumps should be valid knight jumps.

As the answer may be very large, return the answer modulo 10^9 + 7.

Example 1:

Input: n = 1
Output: 10
Explanation: We need to dial a number of length 1, so placing the knight over any numeric cell of the 10 cells is sufficient.

Example 2:

Input: n = 2
Output: 20
Explanation: All the valid number we can dial are [04, 06, 16, 18, 27, 29, 34, 38, 40, 43, 49, 60, 61, 67, 72, 76, 81, 83, 92, 94]

Example 3:

Input: n = 3131
Output: 136006598
Explanation: Please take care of the mod.

Constraints:

1 <= n <= 5000

Solution

Dynamic programming, let f(x, n) denote how many distinct numbers with x as the start and n numbers, then we have: f(x, n) = f(a, n - 1) + f(b, n - 1), where a and b are other numbers that the knight could jump.

The number map is as below:

number_map = {1: (6, 8),2: (7, 9),3: (4, 8), 4: (3, 9, 0),5: (),6: (1, 7, 0),7: (2, 6), 8: (1, 3),9: (2, 4),0: (4, 6)
}

Time complexity: $o(n)$
Space complexity: $o(n)$

Code

class Solution:def knightDialer(self, n: int) -> int:memo = {(i, 1): 1 for i in range(10)}number_map = {1: (6, 8),2: (7, 9),3: (4, 8), 4: (3, 9, 0),5: (),6: (1, 7, 0),7: (2, 6), 8: (1, 3),9: (2, 4),0: (4, 6)}for k in range(2, n + 1):for i in range(10):memo[(i, k)] = 0for next_position in number_map[i]:memo[(i, k)] += memo[(next_position, k - 1)]memo[(i, k)] %= 1000000007return sum(memo[(i, n)] for i in range(10)) % 1000000007

这篇关于leetcode - 935. Knight Dialer的文章就介绍到这儿，希望我们推荐的文章对编程师们有所帮助！

---