LibreOJ - 2589 Hankson 的趣味题

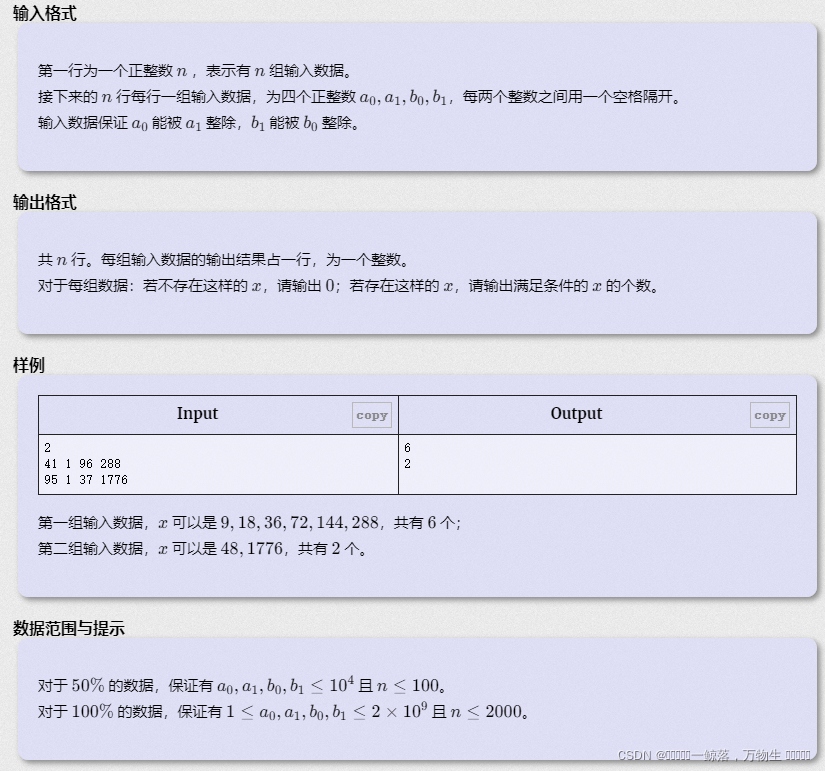
解题思路：

因为x和b0的最小公倍数是b1，所以x一定是b1的约数，我们可以枚举出b1所有的约数，依次对给定的2个条件进行判断，如果符合条件答案加一，现在的问题是如何枚举b1的所有约数，如果暴力从1开始枚举必定会超时，稍微改进一下采用试除法，其时间复杂度为：，依旧会超时，再次改进，我们可以先求出根号2e9内的质数，用这些质数对b1进行质因数分解，得到其质因子和相应的次数，通过质因子和次数可以将b1所有的约数枚举出来判断条件即可，这里先普及一个知识点，1~n中的质数的个数约为n/ln(n)个，所以这样做的时间复杂度为：,这样一来时间复杂度终于是足够了，下面就是激动人心的实现环节了。

#include <iostream>
#include <cstring>
#include <algorithm>
#include <cstdio>
using namespace std;
const int N=50010;
int primes[50010],dcnt,fcnt,cnt;    
struct node{int p;int s;
}factor[10];
int dividor[1610];
int n;
bool st[N];
void init(int n)
{for(int i=2;i<=n;i++){if(!st[i])primes[cnt++]=i;for(int j=0;i*primes[j]<=n;j++){st[i*primes[j]]=true;if(i%primes[j]==0)break;}}
}
void dfs(int u,int p)
{if(u==fcnt){dividor[dcnt++]=p;return ;}for(int i=0;i<=factor[u].s;i++){dfs(u+1,p);p=p*factor[u].p;}
}
int gcd(int a,int b)
{return b?gcd(b,a%b):a;
}
int main()
{cin>>n;init(N-1);while(n--){int a,b,c,d;cin>>a>>b>>c>>d;fcnt=0;int t=d;//对最小公倍数进行质因数分解for(int i=0;primes[i]<=t/primes[i];i++){int p=primes[i];if(t%p==0){int s=0;while(t%p== 0)t/=p,s++;factor[fcnt++]={p,s};	}}if(t>1)factor[fcnt++]={t,1};dcnt=0;//枚举最小公倍数的所有约数dfs(0,1);int ans=0;for(int i=0;i<dcnt;i++){int x=dividor[i];if(gcd(a,x)==b&&(long long)c*x/gcd(c,x)==d)ans++;}cout<<ans<<endl;}return 0;
}