「清新题精讲」CF249D - Donkey and Stars

本文主要是介绍「清新题精讲」CF249D - Donkey and Stars，希望对大家解决编程问题提供一定的参考价值，需要的开发者们随着小编来一起学习吧！

更好的阅读体验

CF249D - Donkey and Stars

Description

给定 $n$ 个点 $x_i,y_i)$ 和 $a, b, c, d$ ，求出最多有多少个点依次连接而成的折线上线段的斜率在 $(\frac{a}{b},\frac{c}{d})$ ，其中第 $1$ 条折线的起点必须在坐标原点且坐标原点不计入答案。

$1\le n\le 10^5,0\le a,b,c,d\le 10^5,1\le x_i,y_i\le 10^5$

Solution

不难想到通过 DP 来求解，暴力 DP 时间复杂度为 $O(n^2)$ ，必然是过不去的。所以，考虑对于点 $i$ ，能够与 $i$ 连线的点 $j$ 应满足哪些性质？

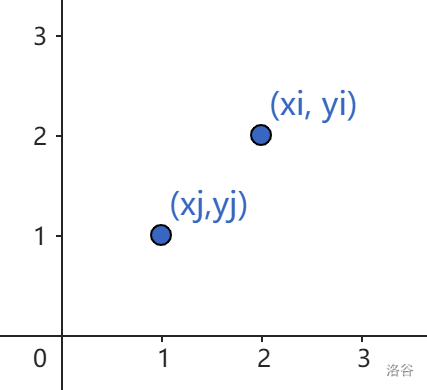
令 $i$ 点位于 $j$ 点的右上方，则有 $\frac{a}{b}< \frac{y_i-y_j}{x_i-x_j}<\frac{c}{d}$ ，通过简单的解不等式可以得到 $by_j-ax_j<by_i-ax_i$ 以及 $dy_i-cx_i<dy_j-cx_j$ ，满足该条件的即可转移。注意到，这其实就是二维偏序，所以只需要令数组按 $by_i-ax_i$ 排序，求最长下降子序列。不过，该题要求必须从原点开始，考虑从后往前枚举，这样相当于求最长上升子序列，最后输出在原点结束的最长长度即可（不要忘记 $- 1$ ）。

综上所述，可以做到 $O(n\log n)$ 。

Code

#include <bits/stdc++.h>
#define fi first
#define se second
#define int long longusing namespace std;typedef pair<int, int> PII;
typedef long long LL;const int N = 1e5 + 10;int n, a, b, c, d;
PII pnt[N];
int f[N];
std::vector<int> dct;
struct fenwick {int tr[N];void add(int x, int d) { for (int i = x; i < N; i += (i & -i)) tr[i] = max(tr[i], d); }int sum(int x) {int res = 0;if (!x) return res;for (int i = x; i; i -= (i & -i)) res = max(res, tr[i]);return res;}
}cnt;int find(int x) {return lower_bound(dct.begin(), dct.end(), x) - dct.begin() + 1;
}signed main() {scanf("%lld\n%lld/%lld %lld/%lld", &n, &a, &b, &c, &d);for (int i = 1; i <= n; i ++)scanf("%lld%lld", &pnt[i].fi, &pnt[i].se), dct.push_back(d * pnt[i].se - c * pnt[i].fi);pnt[0] = {0, 0};sort(dct.begin(), dct.end());dct.erase(unique(dct.begin(), dct.end()), dct.end());sort(pnt, pnt + 1 + n, [&](PII tmp1, PII tmp2) {return tmp1.se * b - tmp1.fi * a < tmp2.se * b - tmp2.fi * a;});for (int i = n, j = n; i >= 0; i --) {while (j > i && pnt[j].se * b - pnt[j].fi * a != pnt[i].se * b - pnt[i].fi * a)cnt.add(find(pnt[j].se * d - pnt[j].fi * c), f[j]), j --;f[i] = cnt.sum(find(pnt[i].se * d - pnt[i].fi * c) - 1) + 1;}for (int i = 0; i <= n; i ++)if (pnt[i] == make_pair(0ll, 0ll)) {cout << f[i] - 1 << endl;return 0;}return 0;}