本文主要是介绍Project Euler 题解 #11 Largest product in a grid,希望对大家解决编程问题提供一定的参考价值,需要的开发者们随着小编来一起学习吧!
题目:Largest product in a grid
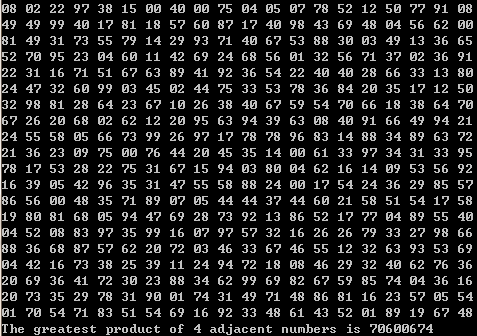
In the 20![]() 20 grid below, four numbers along a diagonal line have been marked in red.
20 grid below, four numbers along a diagonal line have been marked in red.
08 02 22 97 38 15 00 40 00 75 04 05 07 78 52 12 50 77 91 08
49 49 99 40 17 81 18 57 60 87 17 40 98 43 69 48 04 56 62 00
81 49 31 73 55 79 14 29 93 71 40 67 53 88 30 03 49 13 36 65
52 70 95 23 04 60 11 42 69 24 68 56 01 32 56 71 37 02 36 91
22 31 16 71 51 67 63 89 41 92 36 54 22 40 40 28 66 33 13 80
24 47 32 60 99 03 45 02 44 75 33 53 78 36 84 20 35 17 12 50
32 98 81 28 64 23 67 10 26 38 40 67 59 54 70 66 18 38 64 70
67 26 20 68 02 62 12 20 95 63 94 39 63 08 40 91 66 49 94 21
24 55 58 05 66 73 99 26 97 17 78 78 96 83 14 88 34 89 63 72
21 36 23 09 75 00 76 44 20 45 35 14 00 61 33 97 34 31 33 95
78 17 53 28 22 75 31 67 15 94 03 80 04 62 16 14 09 53 56 92
16 39 05 42 96 35 31 47 55 58 88 24 00 17 54 24 36 29 85 57
86 56 00 48 35 71 89 07 05 44 44 37 44 60 21 58 51 54 17 58
19 80 81 68 05 94 47 69 28 73 92 13 86 52 17 77 04 89 55 40
04 52 08 83 97 35 99 16 07 97 57 32 16 26 26 79 33 27 98 66
88 36 68 87 57 62 20 72 03 46 33 67 46 55 12 32 63 93 53 69
04 42 16 73 38 25 39 11 24 94 72 18 08 46 29 32 40 62 76 36
20 69 36 41 72 30 23 88 34 62 99 69 82 67 59 85 74 04 36 16
20 73 35 29 78 31 90 01 74 31 49 71 48 86 81 16 23 57 05 54
01 70 54 71 83 51 54 69 16 92 33 48 61 43 52 01 89 19 67 48
The product of these numbers is 26 ![]() 63
63![]() 78
78![]() 14 = 1788696.
14 = 1788696.
What is the greatest product of four adjacent numbers in the same direction (up, down, left, right, or diagonally) in the 20![]() 20 grid?
20 grid?
这道题目可以用暴力搜索,同时可根据数据全为非负数进行减枝。
数学描述
遍历:
从横、竖、左斜、右斜四个方向进行遍历。
左斜和右斜又分为:主对角、上三角、下三角。
减枝方法:
由于全为非负数,那么最大的乘积必>=0。当遍历到的数为0时,那么可直接跳过。
代码实现:
//https://projecteuler.net/problem=11
//Largest product in a grid
#include "stdafx.h"
#include <iostream>
#include <limits>
#include <fstream>
#include <iomanip>
#include <BaseTsd.h>
using namespace std;
template< class T > T MAX( T a, T b)
{
return a > b? a : b;
}
typedef short ElemType;
INT64 GetMaxProductFromLine(const ElemType *A, int N, int seq_num)
{
if (seq_num > N)
{
cout<<"N must be great than seq_num"<<endl;
return 0;
}
INT64 max_product = LLONG_MIN;
for (int i = 0; i< N - seq_num + 1; ++i)
{
INT64 product = A[i];
for (int j = 1; j < seq_num; ++j)
{
product *= A[i + j];
}
max_product = MAX(max_product, product);
}
return max_product;
}
INT64 GetMaxProductFromMatrix(ElemType **M, int N, int seq_num)
{
ElemType *A = new ElemType[N];
INT64 max = LLONG_MIN, temp = 0;
//横向,竖向
for (int i = 0; i < N; ++i)
{
for (int j = 0; j < N; ++j) //横向搜索
{
A[j] = M[i][j];
}
temp = GetMaxProductFromLine(A, N, seq_num);
max = MAX(max, temp);
for (int j = 0; j < N; ++j) //竖向搜索
{
A[j] = M[j][i];
}
temp = GetMaxProductFromLine(A, N, seq_num);
max = MAX(max, temp);
}
//右斜搜索
for (int i = 0; i < N; ++i) //主对角
{
A[i] = M[i][i];
}
temp = GetMaxProductFromLine(A, N, seq_num);
max = MAX(max, temp);
for (int i = 1; i < N - seq_num + 1; ++i)
{
for (int j = 0; j < N -i; ++j) //上三角
{
A[j] = M[j][j + i];
}
temp = GetMaxProductFromLine(A, N - i, seq_num);
max = MAX(max, temp);
for (int j = 0; j < N -i; ++j) //下三角
{
A[j] = M[j + i][j];
}
temp = GetMaxProductFromLine(A, N - i, seq_num);
max = MAX(max, temp);
}
//左斜搜索
for (int i = 0; i < N; ++i) //主对角
{
A[i] = M[i][N - 1 - i];
}
temp = GetMaxProductFromLine(A, N, seq_num);
max = MAX(max, temp);
for (int i = 1; i < N - seq_num + 1; ++i)
{
for (int j = 0; j < N -i; ++j) //上三角
{
A[j] = M[j][N - 1 - i - j];
}
temp = GetMaxProductFromLine(A, N - i, seq_num);
max = MAX(max, temp);
for (int j = 0; j < N -i; ++j) //下三角
{
A[j] = M[j + i][N - 1 - j];
}
temp = GetMaxProductFromLine(A, N - i, seq_num);
max = MAX(max, temp);
}
delete[] A;
return max;
}
void read_data(ElemType **M, int N)
{
fstream file("data.txt");
for (int i = 0; i < N; ++i)
{
for (int j = 0; j < N; ++j)
{
file>>M[i][j];
}
}
file.close();
}
int _tmain(int argc, _TCHAR* argv[])
{
//int N = 3, num = 2;
int N = 20, num = 4;
ElemType **M = new ElemType *[N];
for (int i = 0; i < N; ++i)
{
M[i] = new ElemType[N];
}
read_data(M, N);
for (int i = 0; i < N; ++i)
{
for (int j = 0; j < N; ++j)
{
cout<<setw(2)<<setfill('0')<<M[i][j]<<" ";
}
cout<<endl;
}
INT64 max = GetMaxProductFromMatrix(M, N, num);
cout<<"The greatest product of "<<num<<" adjacent numbers is "<<max<<endl;
for (int i = 0; i < N; ++i)
{
delete [] M[i];
}
delete M;
system("pause");
return 0;
}输出:
89![]() 94
94![]() 97
97![]() 87=70600674
87=70600674
这篇关于Project Euler 题解 #11 Largest product in a grid的文章就介绍到这儿,希望我们推荐的文章对编程师们有所帮助!