KAJIMA CORPORATION CONTEST 2024（AtCoder Beginner Contest 340）ABCDEF 视频讲解

本文主要是介绍KAJIMA CORPORATION CONTEST 2024（AtCoder Beginner Contest 340）ABCDEF 视频讲解，希望对大家解决编程问题提供一定的参考价值，需要的开发者们随着小编来一起学习吧！

这场比较郁闷，C题短路，连续4次WA，导致罚时太多

A - Arithmetic Progression

Problem Statement

Print an arithmetic sequence with first term $A$, last term $B$, and common difference $D$.
You are only given inputs for which such an arithmetic sequence exists.

Constraints

$1\le A\le B\le 100$
$1\le D\le 100$
There is an arithmetic sequence with first term $A$, last term $B$, and common difference $D$.
All input values are integers.

Input

The input is given from Standard Input in the following format:

$A$ $B$ $D$

Output

Print the terms of the arithmetic sequence with first term $A$, last term $B$, and common difference $D$, in order, separated by spaces.

Sample Input 1

3 9 2

Sample Output 1

3 5 7 9

The arithmetic sequence with first term $3$, last term $9$, and common difference $2$ is $(3,5,7,9)$.

Sample Input 2

10 10 1

Sample Output 2

10

The arithmetic sequence with first term $10$, last term $10$, and common difference $1$ is $(10)$.

Solution

具体见文末视频。

---

Code

#include <bits/stdc++.h>
#define int long longusing namespace std;typedef pair<int, int> PII;
typedef long long LL;signed main()
{cin.tie(0);cout.tie(0);ios::sync_with_stdio(0);int A, B, C;cin >> A >> B >> C;for (int i = A; i <= B; i += C)cout << i << " ";return 0;
}

B - Append

Problem Statement

You have an empty sequence $A$. There are $Q$ queries given, and you need to process them in the order they are given.

The queries are of the following two types:
1 x: Append $x$ to the end of $A$.
2 k: Find the $k$-th value from the end of $A$. It is guaranteed that the length of $A$ is at least $k$ when this query is given.

Constraints

$1\le Q\le 100$
In the first type of query, $x$ is an integer satisfying $1\le x\le 10^9$.
In the second type of query, $k$ is a positive integer not greater than the current length of sequence $A$.

Input

The input is given from Standard Input in the following format:

$Q$
${\mathrm{query}}_1$
${\mathrm{query}}_2$
$⋮$
${\mathrm{query}}_Q$

Each query is in one of the following two formats:

$1$ $x$

$2$ $k$

Output

Print $q$ lines, where $q$ is the number of queries of the second type.

The $i$-th line should contain the answer to the $i$-th such query.

Sample Input 1

5
1 20
1 30
2 1
1 40
2 3

Sample Output 1

30
20

Initially, $A$ is empty.
The first query appends $20$ to the end of $A$, making $A=(20)$.
The second query appends $30$ to the end of $A$, making $A=(20,30)$.
The answer to the third query is $30$, which is the $1$-st value from the end of $A=(20,30)$.
The fourth query appends $40$ to the end of $A$, making $A=(20,30,40)$.
The answer to the fifth query is $20$, which is the $3$-rd value from the end of $A=(20,30,40)$.

Solution

具体见文末视频。

Code

#include <bits/stdc++.h>
#define int long longusing namespace std;typedef pair<int, int> PII;
typedef long long LL;signed main()
{cin.tie(0);cout.tie(0);ios::sync_with_stdio(0);int Q;cin >> Q;std::vector<int> S;while (Q --){int Op, X;cin >> Op >> X;if (Op == 1)S.push_back(X);elsecout << S[S.size() - X] << endl;}return 0;
}

C - Divide and Divide

Problem Statement

There is a single integer $N$ written on a blackboard.

Takahashi will repeat the following series of operations until all integers not less than $2$ are removed from the blackboard:
Choose one integer $x$ not less than $2$ written on the blackboard.
Erase one occurrence of $x$ from the blackboard. Then, write two new integers $\lfloor \frac{x}{2}\rfloor$ and $\lceil \frac{x}{2}\rceil$ on the blackboard.
Takahashi must pay $x$ yen to perform this series of operations.
Here, $\lfloor a\rfloor$ denotes the largest integer not greater than $a$, and $\lceil a\rceil$ denotes the smallest integer not less than $a$.
What is the total amount of money Takahashi will have paid when no more operations can be performed?

It can be proved that the total amount he will pay is constant regardless of the order in which the operations are performed.

Constraints

$2\le N\le 10^{17}$

Input

The input is given from Standard Input in the following format:

$N$

Output

Print the total amount of money Takahashi will have paid, in yen.

Sample Input 1

3

Sample Output 1

5

Here is an example of how Takahashi performs the operations:
Initially, there is one $3$ written on the blackboard.
He chooses $3$. He pays $3$ yen, erases one $3$ from the blackboard, and writes $\lfloor \frac{3}{2}\rfloor =1$ and $\lceil \frac{3}{2}\rceil =2$ on the blackboard.
There is one $2$ and one $1$ written on the blackboard.
He chooses $2$. He pays $2$ yen, erases one $2$ from the blackboard, and writes $\lfloor \frac{2}{2}\rfloor =1$ and $\lceil \frac{2}{2}\rceil =1$ on the blackboard.
There are three $1$s written on the blackboard.
Since all integers not less than $2$ have been removed from the blackboard, the process is finished.
Takahashi has paid a total of $3+2=5$ yen for the entire process, so print $5$.

Sample Input 2

340

Sample Output 2

2888

Sample Input 3

100000000000000000

Sample Output 3

5655884811924144128

Solution

具体见文末视频。

---

Code

#include <bits/stdc++.h>using namespace std;inline __int128 read()
{char ch = getchar();__int128 x = 0, cf = 1;while(ch < '0' || ch > '9') {if(ch == '-') cf = -1;ch = getchar();}while(ch >= '0' && ch <= '9') {x = (x << 3) + (x << 1) + (ch ^ 48);ch = getchar();}return x * cf;
}void write(__int128 x)
{if(x<0)putchar('-'),x=-x;if(x>9)write(x/10);putchar(x%10+'0');return;
}__int128 Quick_Pow(__int128 a, __int128 b)
{__int128 Result = 1;while (b){if (b & 1) Result = Result * a;a = a * a;b >>= 1;}return Result;
}signed main()
{cin.tie(0);cout.tie(0);ios::sync_with_stdio(0);__int128 N = read();__int128 T = 0, T2 = 0;while (Quick_Pow(2, T + 1) <= 4 * N) T ++;while (Quick_Pow(2, T2 + 1) <= 2 * N) T2 ++;__int128 Result = N * T - Quick_Pow(2, T2);write(Result);return 0;
}

D - Super Takahashi Bros.

Problem Statement

Takahashi is playing a game.
The game consists of $N$ stages numbered $1,2,\dots ,N$. Initially, only stage $1$ can be played.
For each stage $i$ ( $1\le i\le N-1$ ) that can be played, you can perform one of the following two actions at stage $i$:
Spend $A_i$ seconds to clear stage $i$. This allows you to play stage $i+1$.
Spend $B_i$ seconds to clear stage $i$. This allows you to play stage $X_i$.
Ignoring the times other than the time spent to clear the stages, how many seconds will it take at the minimum to be able to play stage $N$?

Constraints

$2\le N\le 2\times 10^5$
$1\le A_i,B_i\le 10^9$
$1\le X_i\le N$
All input values are integers.

Input

The input is given from Standard Input in the following format:

$N$
$A_1$ $B_1$ $X_1$
$A_2$ $B_2$ $X_2$
$⋮$
$A_{N-1}$ $B_{N-1}$ $X_{N-1}$

Output

Print the answer.

Sample Input 1

5
100 200 3
50 10 1
100 200 5
150 1 2

Sample Output 1

350

By acting as follows, you will be allowed to play stage $5$ in $350$ seconds.
Spend $100$ seconds to clear stage $1$, which allows you to play stage $2$.
Spend $50$ seconds to clear stage $2$, which allows you to play stage $3$.
Spend $200$ seconds to clear stage $3$, which allows you to play stage $5$.

Sample Input 2

10
1000 10 9
1000 10 10
1000 10 2
1000 10 3
1000 10 4
1000 10 5
1000 10 6
1000 10 7
1000 10 8

Sample Output 2

90

Sample Input 3

6
1000000000 1000000000 1
1000000000 1000000000 1
1000000000 1000000000 1
1000000000 1000000000 1
1000000000 1000000000 1

Sample Output 3

5000000000

Solution

具体见文末视频。

---

Code

#include <bits/stdc++.h>
#define int long longusing namespace std;typedef pair<int, int> PII;
typedef long long LL;const int SIZE = 8e5 + 10;int N;
int A[SIZE], B[SIZE], X[SIZE];
int h[SIZE], w[SIZE], e[SIZE], ne[SIZE], idx;
bool st[SIZE];
int dist[SIZE];inline void add(int a, int b, int c)
{e[idx] = b, ne[idx] = h[a], w[idx] = c, h[a] = idx ++;
}inline int Dijkstra(int start, int finish)
{memset(dist, 0x3f, sizeof dist);priority_queue<PII, vector<PII>, greater<PII>> heap;heap.push({0, start});st[start] = 1;while (heap.size()){auto t = heap.top();heap.pop();int u = t.second, dis = t.first;for (int i = h[u]; ~i; i = ne[i])if (dist[e[i]] > w[i] + dis)dist[e[i]] = w[i] + dis, heap.push({dist[e[i]], e[i]});}if (dist[finish] == 0x3f3f3f3f) return -1;return dist[finish];
}signed main()
{cin.tie(0);cout.tie(0);ios::sync_with_stdio(0);memset(h, -1, sizeof h);cin >> N;for (int i = 1; i < N; i ++){cin >> A[i] >> B[i] >> X[i];add(i, i + 1, A[i]), add(i, X[i], B[i]);}cout << Dijkstra(1, N) << endl;return 0;
}

E - Mancala 2

Problem Statement

There are $N$ boxes numbered $0$ to $N-1$. Initially, box $i$ contains $A_i$ balls.
Takahashi will perform the following operations for $i=1,2,\dots ,M$ in order:
Set a variable $C$ to $0$.
Take out all the balls from box $B_i$ and hold them in hand.
While holding at least one ball in hand, repeat the following process:
Increase the value of $C$ by $1$.
Put one ball from hand into box $(B_i+C)\mathrm{mod}N$.
Determine the number of balls in each box after completing all operations.

Constraints

$1\le N\le 2\times 10^5$
$1\le M\le 2\times 10^5$
$0\le A_i\le 10^9$
KaTeX parse error: Expected 'EOF', got '&' at position 12: 0 \leq B_i &̲lt; N
All input values are integers.

Input

The input is given from Standard Input in the following format:

$N$ $M$
$A_0$ $A_1$ $\dots$ $A_{N-1}$
$B_1$ $B_2$ $\dots$ $B_M$

Output

Let $X_i$ be the number of balls in box $i$ after completing all operations. Print $X_0,X_1,\dots ,X_{N-1}$ in this order, separated by spaces.

Sample Input 1

5 3
1 2 3 4 5
2 4 0

Sample Output 1

0 4 2 7 2

The operations proceed as follows:

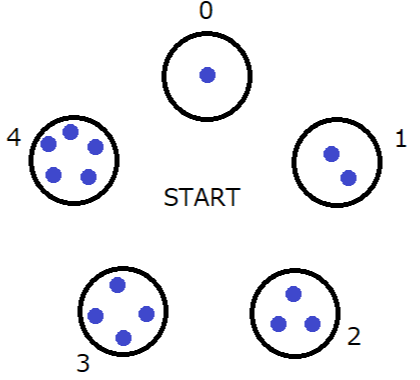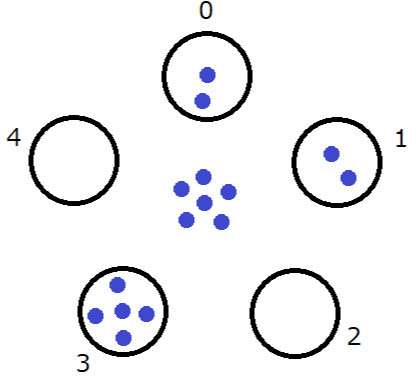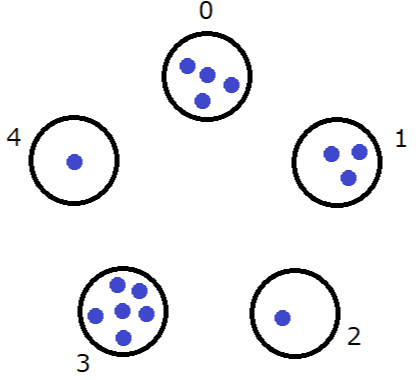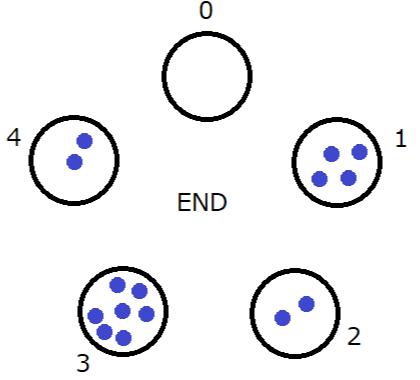
Sample Input 2

3 10
1000000000 1000000000 1000000000
0 1 0 1 0 1 0 1 0 1

Sample Output 2

104320141 45436840 2850243019

Sample Input 3

1 4
1
0 0 0 0

Sample Output 3

1

Solution

具体见文末视频。

---

Code

#include <bits/stdc++.h>
#define int long longusing namespace std;typedef pair<int, int> PII;
typedef long long LL;const int SIZE = 2e5 + 10;int N, M;
int A[SIZE], B[SIZE];
struct Segment
{struct Node{int l, r;LL Sum, Max, Min, Lazy;}Tree[SIZE << 2];void Pushup(int u){Tree[u].Sum = Tree[u << 1].Sum + Tree[u << 1 | 1].Sum;Tree[u].Max = max(Tree[u << 1].Max, Tree[u << 1 | 1].Max);Tree[u].Min = min(Tree[u << 1].Min, Tree[u << 1 | 1].Min);}void Pushdown(int u){if (Tree[u].Lazy){Tree[u << 1].Max += Tree[u].Lazy;Tree[u << 1].Min += Tree[u].Lazy;Tree[u << 1].Sum += (LL)(Tree[u << 1].r - Tree[u << 1].l + 1) * Tree[u].Lazy;Tree[u << 1].Lazy += Tree[u].Lazy;Tree[u << 1 | 1].Max += Tree[u].Lazy;Tree[u << 1 | 1].Min += Tree[u].Lazy;Tree[u << 1 | 1].Sum += (LL)(Tree[u << 1 | 1].r - Tree[u << 1 | 1].l + 1) * Tree[u].Lazy;Tree[u << 1 | 1].Lazy += Tree[u].Lazy;Tree[u].Lazy = 0;}}void Build(int u, int l, int r){Tree[u] = {l, r};if (l == r) return;int mid = l + r >> 1;Build(u << 1, l, mid), Build(u << 1 | 1, mid + 1, r);}void Modify(int u, int l, int r, int d){if (Tree[u].l >= l && Tree[u].r <= r){Tree[u].Sum += (LL)(Tree[u].r - Tree[u].l + 1) * d;Tree[u].Max += d, Tree[u].Min += d;Tree[u].Lazy += d;return;}Pushdown(u);int mid = Tree[u].l + Tree[u].r >> 1;if (mid >= l) Modify(u << 1, l, r, d);if (mid < r) Modify(u << 1 | 1, l, r, d);Pushup(u);}int Query(int u, int l, int r, int k){if (Tree[u].l >= l && Tree[u].r <= r){if (k == 1) return Tree[u].Sum;else if (k == 2) return Tree[u].Max;else return Tree[u].Min;}Pushdown(u);long long mid = Tree[u].l + Tree[u].r >> 1, Result;if (k == 1) Result = 0;else if (k == 2) Result = -1e18;else Result = 1e18;if (mid >= l) Result = Query(u << 1, l, r, k);if (mid < r){if (k == 1) Result += Query(u << 1 | 1, l, r, k);else if (k == 2) Result = max(Result, Query(u << 1 | 1, l, r, k));else Result = min(Result, Query(u << 1 | 1, l, r, k));}return Result;}int Sum(int l, int r) { return Query(1, l, r, 1); }int Max(int l, int r) { return Query(1, l, r, 2); }int Min(int l, int r) { return Query(1, l, r, 3); }
}Tool;signed main()
{cin.tie(0);cout.tie(0);ios::sync_with_stdio(0);cin >> N >> M;Tool.Build(1, 1, N);for (int i = 1; i <= N; i ++)cin >> A[i], Tool.Modify(1, i, i, A[i]);for (int i = 1; i <= M; i ++)cin >> B[i], B[i] ++;for (int i = 1; i <= M; i ++){int X = Tool.Sum(B[i], B[i]), Turn = X / N, Rest = X % N;Tool.Modify(1, 1, N, Turn);if (Rest && B[i] + Rest > N){if (B[i] + 1 <= N) Tool.Modify(1, B[i] + 1, N, 1);Tool.Modify(1, 1, Rest - N + B[i], 1);}else if (Rest) Tool.Modify(1, B[i] + 1, B[i] + Rest, 1);Tool.Modify(1, B[i], B[i], -X);}for (int i = 1; i <= N; i ++)cout << Tool.Sum(i, i) << " ";return 0;
}

F - S = 1

Problem Statement

You are given integers $X$ and $Y$, which satisfy at least one of $X\ne 0$ and $Y\ne 0$.

Find a pair of integers $(A,B)$ that satisfies all of the following conditions. If no such pair exists, report so.
$-10^{18}\le A,B\le 10^{18}$
The area of the triangle with vertices at points $(0,0),(X,Y),(A,B)$ on the $xy$-plane is $1$.

Constraints

$-10^{17}\le X,Y\le 10^{17}$
$(X,Y)\ne (0,0)$
$X$ and $Y$ are integers.

Input

The input is given from Standard Input in the following format:

$X$ $Y$

Output

If there is a pair of integers $(A,B)$ that satisfies the conditions, print it in the following format:

$A$ $B$

Otherwise, print -1.

Sample Input 1

3 5

Sample Output 1

1 1

The area of the triangle with vertices at points $(0,0),(3,5),(1,1)$ is $1$. Thus, $(A,B)=(1,1)$ satisfies the conditions.

Sample Input 2

-2 0

Sample Output 2

0 1

Sample Input 3

8752654402832944 -6857065241301125

Sample Output 3

-1

Solution

具体见文末视频。

---

Code

#include <bits/stdc++.h>
#define int long longusing namespace std;typedef pair<int, int> PII;
typedef long long LL;int Exgcd(int a, int b, int &x, int &y)
{if (!b){x = 1, y = 0;return a;}int d = Exgcd(b, a % b, y, x);y -= a / b * x;return d;
}signed main()
{cin.tie(0);cout.tie(0);ios::sync_with_stdio(0);int X, Y;cin >> X >> Y;if (((2 % __gcd(X, Y) + abs(__gcd(X, Y))) % __gcd(X, Y)) != 0)cout << -1 << endl;else{int A, B;int d = Exgcd(Y, X, A, B);cout << A * (2 / abs(d)) << " " << (-B) * (2 / abs(d)) << endl;}return 0;
}

G - Leaf Color

G题还没研究，等后面研究下。

视频题解

---

最后祝大家早日

这篇关于KAJIMA CORPORATION CONTEST 2024（AtCoder Beginner Contest 340）ABCDEF 视频讲解的文章就介绍到这儿，希望我们推荐的文章对编程师们有所帮助！

---