主动IRS（Active IRS）模型总结

本文主要是介绍主动IRS（Active IRS）模型总结，希望对大家解决编程问题提供一定的参考价值，需要的开发者们随着小编来一起学习吧！

active IRS 与 passive IRS模型的区别

A Framework for Transmission Design for Active RIS-Aided Communication with Partial CSI

2023.5 TWC

https://ieeexplore.ieee.org/abstract/document/10134546

As shown in Fig. 1, we consider an RIS-aided downlink multiple-input single-output (MISO) system, where an $\mathrm{N}$ antenna BS communicates with $K$ single-antenna users. The RIS is assumed to be equipped with $M$ reflecting elements, and its reflection coefficient matrix is given by ${\mathbf{\Lambda }}_{\mathbf{w}}=\mathrm{Diag}\left(w_1,\cdots ,w_M\right)\in {\mathbb{C}}^{M\times M}$. Here, $\angle w_m$ and $\left|w_m\right|$ denote the phase shift and the reflection gain of the $m$ -th RIS element, respectively. For passive RISs, each RIS element comprises an impedance adjustable circuit to vary the phase shift [19]. Thus, passive RISs are capable of reflecting the incident signal ${}^2$ without consuming direct-current (DC) power, which leads to a reflection gain of ${\left|w_m\right|}^2=1$ and negligible thermal noise. However, the multiplicative fading effect results in a weak received signal power for the passive RIS reflection link. To address this issue, the authors of [9] and [10] proposed a new active RIS architecture, where each active RIS element includes an active reflection-type amplifier to also amplify the incident signals. Therefore, the corresponding reflection gain is given by $1\le {\left|w_m\right|}^2\le a_{\max }$ , where $a_{\max }$ is the maximum amplification gain.

The BS transmits $K$ data symbols collected in vector $\mathbf{s}={\left[s_1,\cdots ,s_K\right]}^{\mathrm{T}}\in {\mathbb{C}}^{K\times 1}$ to the users by applying precoder matrix $\mathbf{F}=\left[{\mathbf{f}}_1,\cdots ,{\mathbf{f}}_K\right]\in {\mathbb{C}}^{N\times K}$ . Assuming independent complex Gaussian signals with $\mathbb{E}\left[{\mathbf{ss}}^{\mathrm{H}}\right]={\mathbf{I}}_N$ , the BS transmit power is given by $\mathbb{E}\left\{\Vert \mathbf{Fs}{\Vert }_2^2\right\}=\Vert \mathbf{F}{\Vert }_F^2\le P_N$ , where $P_N$ is the BS transmit power budget. Denote by ${\mathbf{H}}_{\mathrm{dr}}\in {\mathbb{C}}^{M\times N}$ the channel from the BS to the RIS, and by ${\mathbf{h}}_k\in {\mathbb{C}}^{N\times 1}$ and ${\mathbf{h}}_{\mathrm{r},k}\in {\mathbb{C}}^{M\times 1}$ the channels from user $k$ to the BS and to the RIS, respectively. Then, the signal received by user $k$ is given by

$$\begin{array}{rl}{y}_k& ={\mathbf{h}}_k^{\mathrm{H}}\mathbf{Fs}+{\mathbf{h}}_{\mathrm{r},k}^{\mathrm{H}}{\mathbf{\Lambda }}_{\mathbf{w}}\left({\mathbf{H}}_{\mathrm{dr}}\mathbf{Fs}+\mathbf{z}\right)+n_k\\ & =\left({\mathbf{h}}_k^{\mathrm{H}}+{\mathbf{h}}_{\mathrm{r},k}^{\mathrm{H}}{\mathbf{\Lambda }}_{\mathbf{w}}{\mathbf{H}}_{\mathrm{dr}}\right)\mathbf{Fs}+{\mathbf{h}}_{\mathrm{r},k}^{\mathrm{H}}{\mathbf{\Lambda }}_{\mathbf{w}}\mathbf{z}+n_k,\end{array}$$

where $n_k$ and $\mathbf{z}$ are the zero-mean additive white Gaussian noise (AWGN) at the user and the RIS, respectively, which follow distributions $n_k\sim \mathrm{CN}\left(0,{\sigma }_k^2\right)$ and $\mathbf{z}\sim \mathrm{CN}\left(0,{\sigma }_z^2{\mathbf{I}}_M\right)$ with noise powers ${\sigma }_k^2$ and ${\sigma }_z^2$ , respectively. Notice that the thermal noise $\mathbf{z}$ , which can be ignored in passive RIS-aided systems, has to be considered in active RIS-aided systems because of the amplification. The transmit power of the active RIS is given by

$$\mathbb{E}\left\{{\Vert {\mathbf{\Lambda }}_{\mathbf{w}}\left({\mathbf{H}}_{\mathrm{dr}}\mathbf{Fs}+\mathbf{z}\right)\Vert }_2^2\right\}={\Vert {\mathbf{\Lambda }}_{\mathbf{w}}{\mathbf{H}}_{\mathrm{dr}}\mathbf{F}\Vert }_F^2+\Vert \mathbf{w}{\Vert }_2^2{\sigma }_z^2,$$

where $\mathbf{w}={\left[w_1,\cdots ,w_M\right]}^{\mathrm{H}}$ . Furthermore, the achievable rate of user $k$ is given by

$$R_k(\mathbf{F},\mathbf{w})={\log }_2\left(1+\frac{1}{{\mathrm{IN}}_k}{\left|\left({\mathbf{h}}_k^{\mathrm{H}}+{\mathbf{h}}_{\mathrm{r},k}^{\mathrm{H}}{\mathbf{\Lambda }}_{\mathbf{w}}{\mathbf{H}}_{\mathrm{dr}}\right){\mathbf{f}}_k\right|}^2\right)$$

where ${\mathrm{IN}}_k={\sum }_{i=1,i\ne k}^K{\left|\left({\mathbf{h}}_k^{\mathrm{H}}+{\mathbf{h}}_{\mathrm{r},k}^{\mathrm{H}}{\mathbf{\Lambda }}_{\mathbf{w}}{\mathbf{H}}_{\mathrm{dr}}\right){\mathbf{f}}_i\right|}^2+{\sigma }_k^2+{\Vert {\mathbf{h}}_{\mathrm{r},k}^{\mathrm{H}}{\mathbf{\Lambda }}_{\mathbf{w}}\Vert }_2^2{\sigma }_z^2$ .

Hybrid active and passive IRS

统计信道状态信息和worst case信道状态信息有何区别。

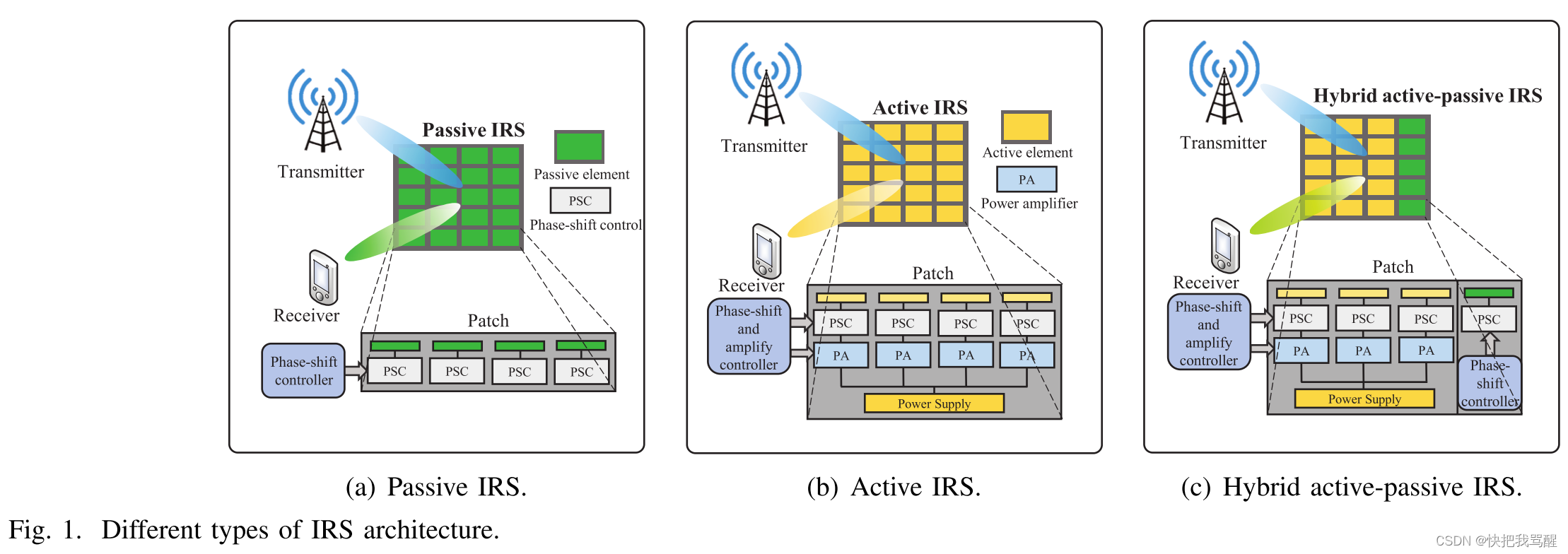
为了解决上述问题，最近提出了一种新型 IRS，称为主动 IRS（参见[20]-[24]）。具体地，有源IRS包括多个有源反射元件，每个有源反射元件配备有有源负载（或称为负电阻），例如隧道二极管和负阻抗转换器。如图 1（b）所示，每个有源负载都连接到一个附加电源以进行信号放大[25]、[26]。因此，有源 IRS 不仅能够像无源 IRS 一样实现可调节的相移，而且还允许在全双工模式下对入射信号进行幅度放大（即大于 1），尽管其硬件和能源成本比无源 IRS 稍高一些 [21]。另一方面，与将射频链连接到天线的有源中继相比，有源 IRS 不需要昂贵且耗电的射频链组件 [27]。最近的文献研究了主动式 IRS 和被动式 IRS 之间的性能比较。例如，给定主动 IRS 位置和功率预算，事实证明，主动 IRS 可以比被动 IRS 实现更高的频谱效率 [21]、[28]、能源效率 [24] 和可靠性 [29]。此外，[23] 中的作者进一步优化了被动和主动 IRS 辅助系统的 IRS 放置，以实现速率最大化。结果表明，当反射元件数量较大和/或有源元件放大功率较小时，无源 IRS 通过各自的优化放置实现了比有源 IRS 更高的速率性能。此外，[22]中的作者考虑了无源和有源IRS辅助系统相同的功率预算约束，其中在有源IRS的情况下，同时考虑了基站（BS）的发射功率和有源IRS的放大功率。结果表明，只有当反射元件的数量很少和/或有源IRS的放大功率足够大时，有源IRS才优于无源IRS。

总而言之，现有的 IRS 工作表明，被动 IRS 和主动 IRS 具有互补优势。具体来说，无源 IRS 比有源 IRS 具有更高的渐近波束形成增益（即 O(N2) 与 O(N)），因此当反射元件数量 N 较大时更有吸引力 [21]、[23]。相比之下，有源 IRS 提供额外的功率放大增益，当 N 相对较小时，这会导致比无源 IRS 高得多的信噪比 (SNR) [25]、[30]、[31]。此外，主动IRS通常比被动IRS产生更高的成本和功耗。因此，这些表明，给定IRS部署成本的总预算（或者等效地要部署的有源/无源反射元件的数量），仅具有无源或有源元件的传统IRS架构可能无法实现最佳通信性能。

受上述启发，我们在本文中提出了一种新的混合主动-被动IRS2架构，如图1（c）所示，以实现被动和主动IRS的优点，进一步提高比单独使用主动或被动IRS的性能。具体来说，混合IRS由两个位于同一位置的子表面组成，每个子表面分别由一定数量的无源和有源反射元件组成。

为了实现最佳性能，我们在给定的部署预算下设计了混合IRS的有源与无源反射元件分配，以最佳平衡有源IRS独特的功率放大增益和无源IRS比有源IRS更高的波束成形增益之间的权衡。为此，我们考虑如图 2 所示的混合主动-被动 IRS 辅助多用户通信系统，其中 BS 向用户集群传输独立数据。混合IRS被正确地部署在该用户集群的边缘，以在不同的时隙为其半空间反射区域中的用户提供服务。我们根据实际模型考虑混合 IRS 的给定部署预算，以及每个有源和无源元件的不同成本，其中有源元件通常比无源元件产生更高的成本3。为了减少实时信道估计开销并避免频繁的 IRS 反射调整，我们考虑一种实用的方法，即仅基于统计信道状态信息（CSI）设计混合 IRS 波束成形，假设实际的莱斯衰落信道模型（即仅假设信道路径损耗参数和莱斯衰落因子已知），而不需要了解所有相关链路的瞬时 CSI。下面，我们总结了本文的主要贡献。

从已有passive IRS算法拓展到active IRS算法

不需要考虑原来的被动相位约束，需要新增加Active IRS的发射功率约束。
passive IRS可以视为Active IRS的特例

这篇关于主动IRS（Active IRS）模型总结的文章就介绍到这儿，希望我们推荐的文章对编程师们有所帮助！

---