[从头学数学] 第192节 导数及其应用

2023-11-08 06:40
文章标签 应用 数学 导数 从头 192

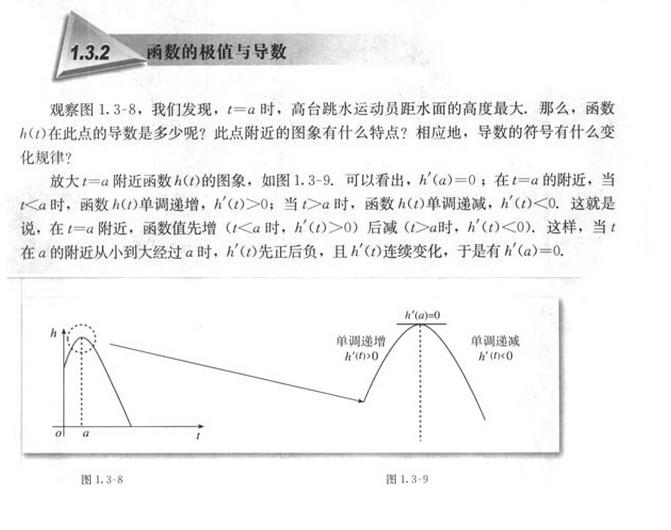
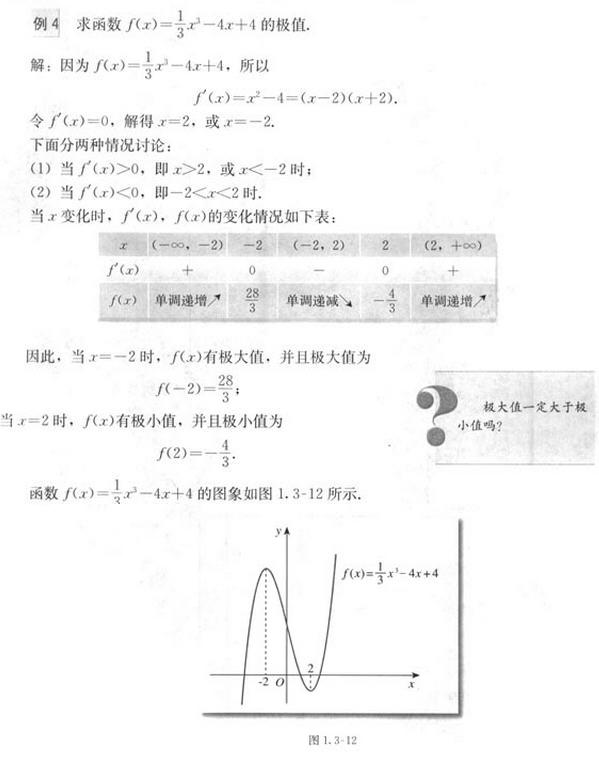
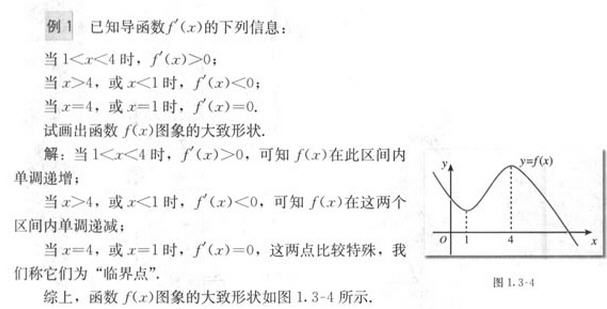
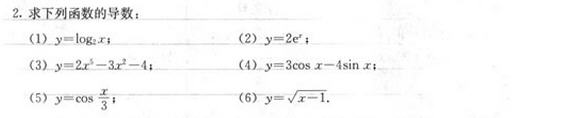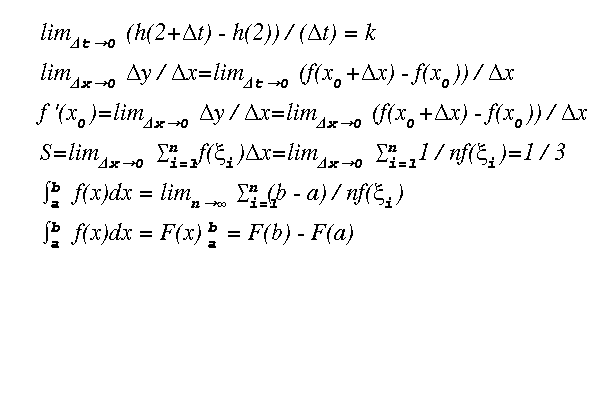本文主要是介绍[从头学数学] 第192节 导数及其应用，希望对大家解决编程问题提供一定的参考价值，需要的开发者们随着小编来一起学习吧！

剧情提要：
[机器小伟]在[工程师阿伟]的陪同下进入了[九转金丹]之第五转的修炼。
这次要研究的是[导数及其应用]。

正剧开始：

星历2016年04月23日 16:32:36, 银河系厄尔斯星球中华帝国江南行省。
[工程师阿伟]正在和[机器小伟]一起研究[导数及其应用]。





<span style="font-size:18px;">>>> 
[-3.000001001396413, -2.999998999442255]
[4.999998999721811, 5.000000996346898]def taskFun(x):return x**2-7*x+15;def derivatives(x):epsilon = 1e-6;leftValue = taskFun(x-epsilon);rightValue = taskFun(x+epsilon);value = taskFun(x);leftLimit = (value-leftValue)/epsilon;rightLimit = (rightValue-value)/epsilon;    return [leftLimit, rightLimit];def tmp():print(derivatives(2));print(derivatives(6));</span>


 




<span style="font-size:18px;">>>> 
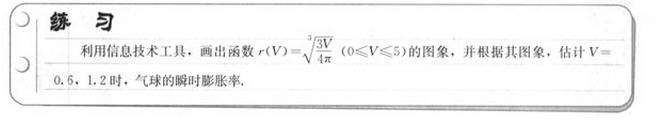
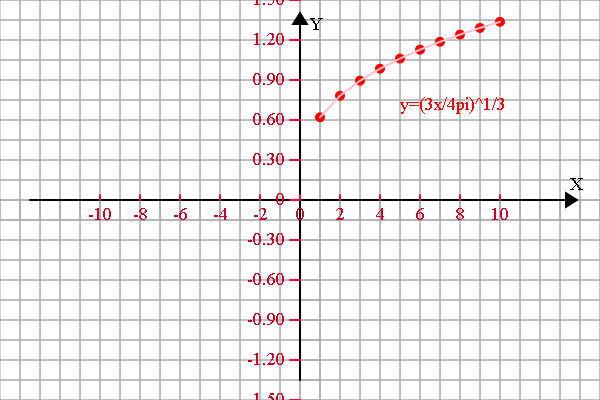
函数在0.6点的左、右导数分别是：[0.29068008811083956, 0.290679765257984]
函数在1.2点的左、右导数分别是：[0.1831169299526536, 0.18311682836724685]def taskFun(x):return (3*x/(4*math.pi))**(1/3);def derivatives(x):epsilon = 1e-6;leftValue = taskFun(x-epsilon);rightValue = taskFun(x+epsilon);value = taskFun(x);leftLimit = (value-leftValue)/epsilon;rightLimit = (rightValue-value)/epsilon;    return [leftLimit, rightLimit];def tmp():a = [0.6, 1.2];for i in range(len(a)):print('函数在{0}点的左、右导数分别是：{1}'.format(a[i],derivatives(a[i])));</span>


 







<span style="font-size:18px;">>>> 
函数在10点的左、右导数分别是：[0.07947403424246602, 0.07947403823926891]
>>> 1.05**10*math.log(1.05)
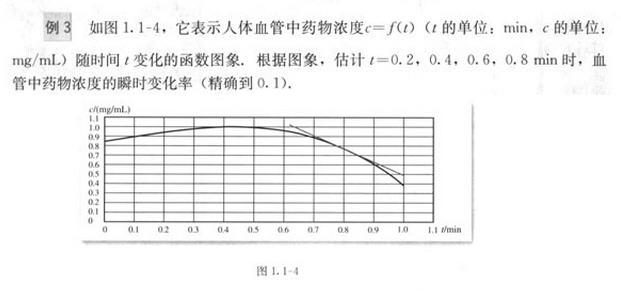
0.07947403625517714def taskFun(x):return (1+0.05)**x;def derivatives(x):epsilon = 1e-6;leftValue = taskFun(x-epsilon);rightValue = taskFun(x+epsilon);value = taskFun(x);leftLimit = (value-leftValue)/epsilon;rightLimit = (rightValue-value)/epsilon;    return [leftLimit, rightLimit];#例1
def tmp():a = [10];for i in range(len(a)):print('函数在{0}点的左、右导数分别是：{1}'.format(a[i],derivatives(a[i])));</span>


 



<span style="font-size:18px;">>>> 
函数在自变量取值为90处的左、右导数分别是：[52.83999450966803, 52.84000519623078]
函数在自变量取值为98处的左、右导数分别是：[1320.9993362579553, 1321.0006572990096]def taskFun(x):return 5284/(100-x);def derivatives(x):epsilon = 1e-6;leftValue = taskFun(x-epsilon);rightValue = taskFun(x+epsilon);value = taskFun(x);leftLimit = (value-leftValue)/epsilon;rightLimit = (rightValue-value)/epsilon;    return [leftLimit, rightLimit];#例3
def tmp():a = [90, 98];for i in range(len(a)):print('函数在自变量取值为{0}处的左、右导数分别是：{1}'.format(a[i],derivatives(a[i])));</span>


 




<span style="font-size:18px;">	if (1) {  var r = 20;        config.setSector(1,1,1,1);          config.graphPaper2D(0, 0, r);        config.axis2D(0, 0,180);          //坐标轴设定    var scaleX = 2*r, scaleY = 2*r;      var spaceX = 2, spaceY = 0.3;       var xS = -10, xE = 10;      var yS = -10, yE = 10;      config.axisSpacing(xS, xE, spaceX, scaleX, 'X');        config.axisSpacing(yS, yE, spaceY, scaleY, 'Y');        var transform = new Transform();        //存放函数图像上的点    var a = [], b = [], c = [], d = [];      //需要显示的函数说明    var f1 = 'y=log_[2]x的导数', f2 = '', f3 = '', f4 = '';    var epsilon = 0.000001;var derivative = 0;//函数描点    for (var x = xS; x <= xE; x+=1) {      derivative = (funTask(x+epsilon)-funTask(x))/epsilon;a.push([x, derivative]);      }      //存放临时数组    var tmp = [];    //显示变换    if (a.length > 0) {    a = transform.scale(transform.translate(a, 0, 0), scaleX/spaceX, scaleY/spaceY);     //函数1    tmp = [].concat(a);        shape.pointDraw(tmp, 'red');        tmp = [].concat(a);        shape.multiLineDraw(tmp, 'pink');      plot.setFillStyle('red');    plot.fillText(f1, 100, -90, 200);      }  }}function funTask(x) {return Math.log(x)/Math.log(2);
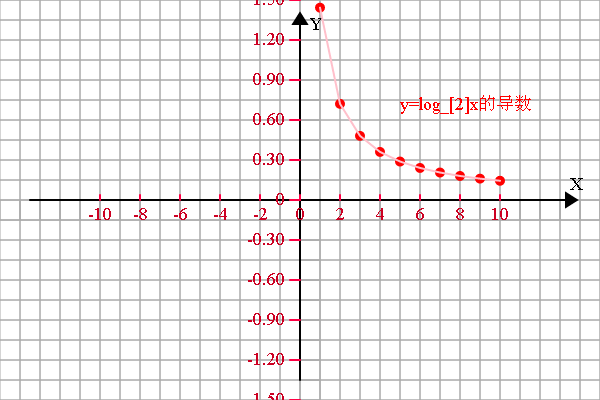
}function funTask(x) {return 2*Math.pow(Math.E, x);
}</span>


 


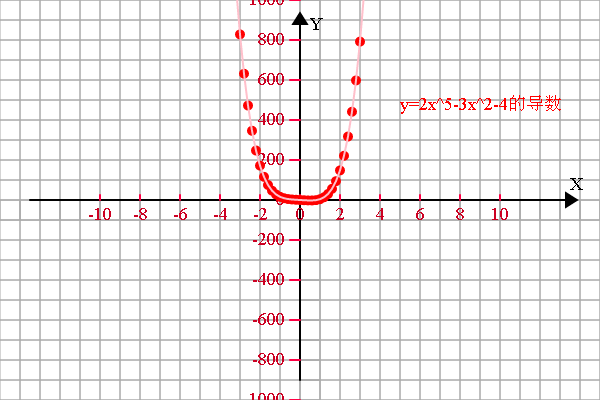
<span style="font-size:18px;">	if (1) {  var r = 20;        config.setSector(1,1,1,1);          config.graphPaper2D(0, 0, r);        config.axis2D(0, 0,180);          //坐标轴设定    var scaleX = 2*r, scaleY = 2*r;      var spaceX = 2, spaceY = 200;       var xS = -10, xE = 10;      var yS = -1000, yE = 1000;      config.axisSpacing(xS, xE, spaceX, scaleX, 'X');        config.axisSpacing(yS, yE, spaceY, scaleY, 'Y');        var transform = new Transform();        //存放函数图像上的点    var a = [], b = [], c = [], d = [];      //需要显示的函数说明    var f1 = 'y=2x^5-3x^2-4的导数', f2 = '10x^4-6x', f3 = '', f4 = '';    var epsilon = 0.000001;var derivative = 0;//函数描点    for (var x = xS; x <= xE; x+=0.2) {      //derivative = (funTask(x+epsilon)-funTask(x))/epsilon;// a.push([x, derivative]);      b.push([x, funTask_2(x)]);}      //存放临时数组    var tmp = [];    //显示变换    if (a.length > 0) {    a = transform.scale(transform.translate(a, 0, 0), scaleX/spaceX, scaleY/spaceY);     //函数1    tmp = [].concat(a);        shape.pointDraw(tmp, 'red');        tmp = [].concat(a);        shape.multiLineDraw(tmp, 'pink');      plot.setFillStyle('red');    plot.fillText(f1, 100, -90, 200);      }  //显示变换    if (b.length > 0) {    b = transform.scale(transform.translate(b, 0, 0), scaleX/spaceX, scaleY/spaceY);     //函数1    tmp = [].concat(b);        shape.pointDraw(tmp, 'blue');        tmp = [].concat(b);        shape.multiLineDraw(tmp, '#22ccFF');      plot.setFillStyle('blue');    plot.fillText(f2, 100, -90, 200);      } }}function funTask(x) {return 2*Math.pow(x, 5)-3*Math.pow(x, 2)-4;
}function funTask_2(x) {return 10*Math.pow(x, 4)-6*x;
}
</span>

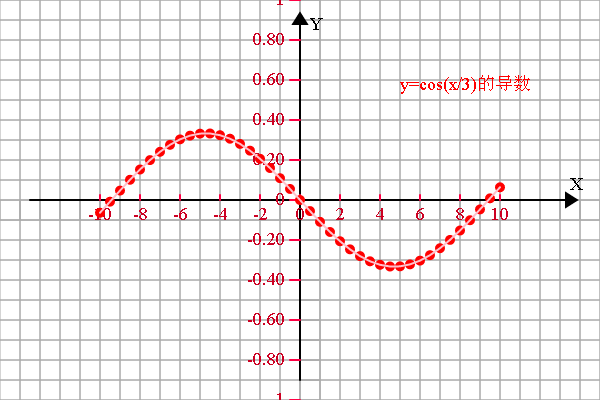
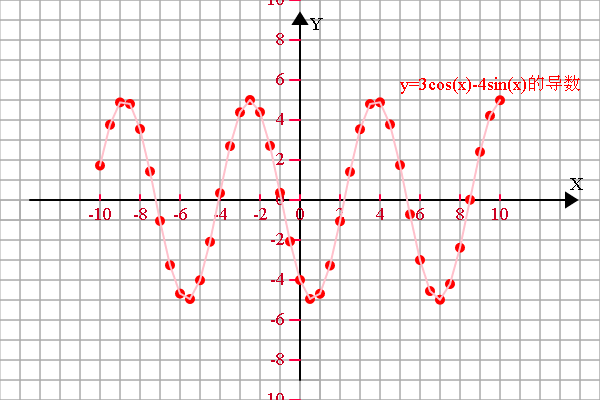
<span style="font-size:18px;">	if (1) {  var r = 20;        config.setSector(1,1,1,1);          config.graphPaper2D(0, 0, r);        config.axis2D(0, 0,180);          //坐标轴设定    var scaleX = 2*r, scaleY = 2*r;      var spaceX = 2, spaceY = 2;       var xS = -10, xE = 10;      var yS = -10, yE = 10;      config.axisSpacing(xS, xE, spaceX, scaleX, 'X');        config.axisSpacing(yS, yE, spaceY, scaleY, 'Y');        var transform = new Transform();        //存放函数图像上的点    var a = [], b = [], c = [], d = [];      //需要显示的函数说明    var f1 = 'y=3cos(x)-4sin(x)的导数', f2 = '10x^4-6x', f3 = '', f4 = '';    var epsilon = 0.000001;var derivative = 0;//函数描点    for (var x = xS; x <= xE; x+=0.5) {      derivative = (funTask(x+epsilon)-funTask(x))/epsilon;a.push([x, derivative]);      //b.push([x, funTask_2(x)]);}      //存放临时数组    var tmp = [];    //显示变换    if (a.length > 0) {    a = transform.scale(transform.translate(a, 0, 0), scaleX/spaceX, scaleY/spaceY);     //函数1    tmp = [].concat(a);        shape.pointDraw(tmp, 'red');        tmp = [].concat(a);        shape.multiLineDraw(tmp, 'pink');      plot.setFillStyle('red');    plot.fillText(f1, 100, -110, 200);      }  //显示变换    if (b.length > 0) {    b = transform.scale(transform.translate(b, 0, 0), scaleX/spaceX, scaleY/spaceY);     //函数1    tmp = [].concat(b);        shape.pointDraw(tmp, 'blue');        tmp = [].concat(b);        shape.multiLineDraw(tmp, '#22ccFF');      plot.setFillStyle('blue');    plot.fillText(f2, 100, -90, 200);      } }}function funTask(x) {return 3*Math.cos(x)-4*Math.sin(x);
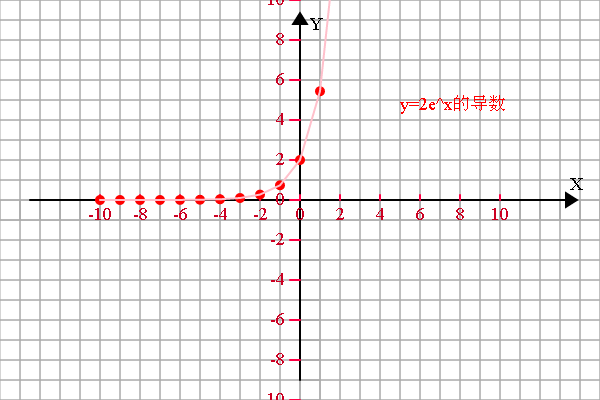
}function funTask(x) {return Math.cos(x/3);
}</span>



 





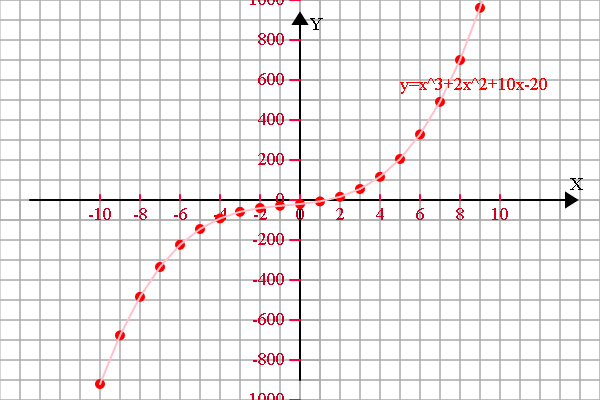
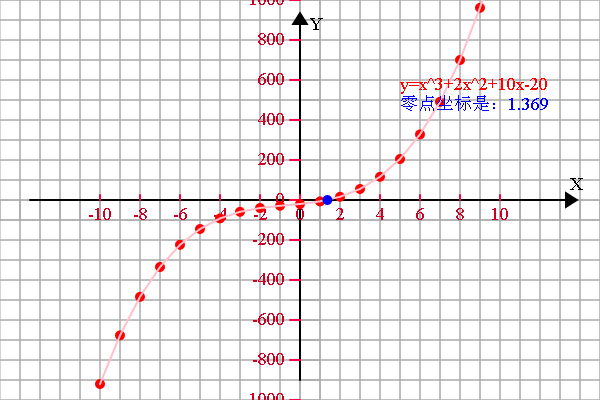
<span style="font-size:18px;">	if (1) {  var r = 20;        config.setSector(1,1,1,1);          config.graphPaper2D(0, 0, r);        config.axis2D(0, 0,180);          //坐标轴设定    var scaleX = 2*r, scaleY = 2*r;      var spaceX = 2, spaceY = 200;       var xS = -10, xE = 10;      var yS = -1000, yE = 1000;      config.axisSpacing(xS, xE, spaceX, scaleX, 'X');        config.axisSpacing(yS, yE, spaceY, scaleY, 'Y');        var transform = new Transform();        //存放函数图像上的点    var a = [], b = [], c = [], d = [];      //需要显示的函数说明    var f1 = 'y=x^3+2x^2+10x-20', f2 = '10x^4-6x', f3 = '', f4 = '';    var epsilon = 0.000001;var derivative = 0;//给定初始试探点var x0 = 4, xResult = 0;//函数描点    for (var x = xS; x <= xE; x++) {      a.push([x, funTask(x)]);   }      //牛顿法求零点derivative = (funTask(x0+epsilon)-funTask(x0))/epsilon;xResult = x0-funTask(x0)/derivative;while (Math.abs((xResult-x0)/x0)>epsilon) {x0 = xResult;derivative = (funTask(x0+epsilon)-funTask(x0))/epsilon;xResult = x0 -funTask(x0)/derivative;}b.push([xResult, 0]);//存放临时数组    var tmp = [];    //显示变换    if (a.length > 0) {    a = transform.scale(transform.translate(a, 0, 0), scaleX/spaceX, scaleY/spaceY);     //函数1    tmp = [].concat(a);        shape.pointDraw(tmp, 'red');        tmp = [].concat(a);        shape.multiLineDraw(tmp, 'pink');      plot.setFillStyle('red');    plot.fillText(f1, 100, -110, 200);      }  //显示变换    if (b.length > 0) {  plot.setFillStyle('blue');    plot.fillText('零点坐标是：'+b[0][0].toFixed(3), 100, -90, 200);  b = transform.scale(transform.translate(b, 0, 0), scaleX/spaceX, scaleY/spaceY);     //函数1    tmp = [].concat(b);        shape.pointDraw(tmp, 'blue');        } }}function funTask(x) {return Math.pow(x, 3)+2*Math.pow(x, 2)+10*x-20;
}
</span>

















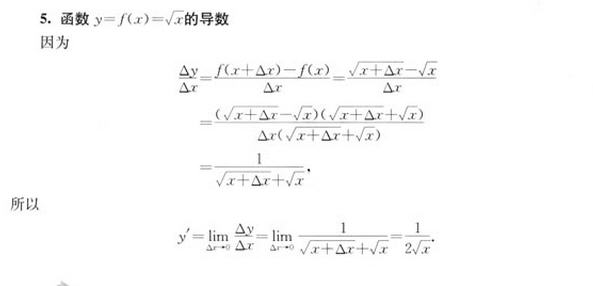
这是一张勉强能用的手写式，如果要想做成印刷上的多行立体形态，只怕是不容易。

当然，那就是公式编辑器的事了。


<span style="font-size:18px;">	if (1) {var mathText = new MathText();//希腊字母表(存此用于Ctrl C/V//ΑΒΓΔΕΖΗΘΙΚΛΜΝΞΟΠΡΣΤΥΦΧΨΩ//αβγδεζηθικλμνξοπρστυφχψωvar s = ['lim_[Δt->0](h(2+Δt)-h(2))/(Δt) = k','lim_[Δx->0]Δy/Δx=lim_[Δt->0](f(x_[0]+Δx)-f(x_[0]))/Δx','f \'(x_[0])=lim_[Δx->0]Δy/Δx=lim_[Δx->0](f(x_[0]+Δx)-f(x_[0]))/Δx','S=lim_[Δx->0]Ξ_[i=1]^[n]   f(ξ_[i])Δx=lim_[Δx->0]Ξ_[i=1]^[n]   1/nf(ξ_[i])=1/3','[S]_[a]^[b]  f(x)dx = lim_[n->[inf]]Ξ_[i=1]^[n] (b-a)/nf(ξ_[i])','[S]_[a]^[b]  f(x)dx = F(x) |_[a]^[b] = F(b)-F(a)',];var x =40, y=40;var r1 = 40;var len = s.length;for (var i = 0; i < len; i++) {if (s[i] == '') {if (x < 100) {x += 300;y-=r1*3;}else {x = 20;y += r1;}}else {			mathText.printIntegral(s[i], x, y, '@');y+=r1;}}		}/**
* @usage   数学表达式，代数式的书写
* @author  mw
* @date    2016年03月12日  星期六  11:05:12 
* @param
* @return
*
*/
function MathText() {//上标标记形式为...^[内容]...//分数不进行处理， 根式不进行处理，都转成指数式进行//特殊数学符号设想加\[alpha]进行转义，待续//可以进行指数上标代数式的书写//可扩展下标，待续this.setNormalFont = function() {plot.setFont("normal normal normal 24px Times Lt Std");	}this.setScriptFont = function() {plot.setFont("italic normal bold 16px Dark Courier ");}this.print = function(text, xPos, yPos, style, splitChar) {splitChar = splitChar ? splitChar : '|';xPos = xPos ? xPos : 0;yPos = yPos ? yPos : 0;style = style ? style : 'black';plot.save().setStrokeStyle(style).setFillStyle(style);var s = text ? text : '';if (s != '') {s = s.replace(/\/\//ig, '÷');s = s.replace(/\[>=\]/ig, '≥');s = s.replace(/\[<=\]/ig, '≤');s = s.replace(/\[!=\]/ig, '≠');s = s.replace(/\[PI\]/ig, 'π');s = s.replace(/\[+-\]/ig, '±');s = s.replace(/->/ig, '→');s = s.replace(/<-/ig, '←');s = s.replace(/<-/ig, '←');s = s.replace(/Ξ/g, '\u2211');}//字符串长度var len = s.length;//不同字体大小设置在此var r1 = 20;//单个字符暂存处var c;//文本显示位置var x = xPos, y = yPos;//正常文本暂存var s0 = '';//字符串打印长度var measure; //记录上一个x位置,可记录三层var xMem = [x, x, x];//记录每一层的左括号位置var bracketPos = [x, x, x];//记录括号层次var bracketsLevel = 0;//记录根号层次var radicalLevel = 0;//记录每一层根号的起始位置和层次数的数组...[[start, end, level], ...]var radicalSpan = [];//设置正常字体this.setNormalFont();				for (var i = 0; i < len; i++) {if (s[i] == '_' && s[i+1] == '[') {//下标开始//下标标记形式为..._[内容]...if (s0 != '') { //先把正常字符打印出if (r1 != 20) { //字体字号大小还在上标状态r1 = 20;this.setNormalFont();					}measure = plot.measureText(s0);plot.fillText(s0, x, y, measure);s0 = '';x += measure;}var subScript = '';var j = 0;for (j = i+1; s[j]!=']'; j++) {if (s[j] != '[') {subScript+=s[j];}}if (r1 != 10) {//正常字体状态，需要改为上标字体r1 = 10;this.setScriptFont();}measure = plot.measureText(subScript);plot.fillText(subScript, x, y+8, measure);if (j < len-1 && s[j+1] == '^') {}else {x += 1.2*measure;}i = j;}else if (s[i] == '^'&&s[i+1] == '[') {//上标开始//上标标记形式为...^[内容]...if (s0 != '') { //先把正常字符打印出if (r1 != 20) { //字体字号大小还在上标状态r1 = 20;this.setNormalFont();					}measure = plot.measureText(s0);plot.fillText(s0, x, y, measure);s0 = '';x += measure;}var upperScript = '';var j = 0;for (j = i+1; s[j]!=']'; j++) {if (s[j] != '[') {upperScript+=s[j];}}//二次根式if (upperScript == '1/2' || upperScript == '0.5') {		var x1, y1;if (i > 0 && s[i-1] == ')') {x1 = bracketPos[bracketsLevel];}else {x1 = xMem[bracketsLevel];}/* 存疑代码if (radicalSpan == []) {radicalLevel = 0;radicalSpan.push([x1, x, radicalLevel]);}else {var len = radicalSpan.length;for (var k = 0; k < len; k++) {if (x1 < radicalSpan[k][0]) {radicalLevel = radicalSpan[k][2]+1;break;}if (k >= len-1) {radicalLevel = 0;}}radicalSpan.push([x1, x, radicalLevel]);}*/y1 = y-20-5*radicalLevel;			plot.save().setLineWidth(1);plot.beginPath().moveTo(x1-5, y+5).lineTo(x1-8, y-3).moveTo(x1-5, y+5).lineTo(x1+5, y1).moveTo(x1+5, y1).lineTo(x, y1).closePath().stroke();plot.restore();}//向量符号else if (upperScript == '->') {var x1, y1;if (i > 0 && s[i-1] == ')') {x1 = bracketPos[bracketsLevel];}else {x1 = xMem[bracketsLevel];}y1 = y-18-5*radicalLevel;			plot.save().setLineWidth(1);plot.beginPath().moveTo(x1, y1).lineTo(x+2, y1).moveTo(x+2, y1).lineTo(x-5, y1-3).moveTo(x+2, y1).lineTo(x-5, y1+3).closePath().stroke();plot.restore();}else {if (r1 != 10) {//正常字体状态，需要改为上标字体r1 = 10;this.setScriptFont();}measure = plot.measureText(upperScript);plot.fillText(upperScript, x, y-8, measure);if (j < len-1 && s[j+1] == '_') {}else {x += 1.2*measure;}}//直接跳跃过上标字符区段i = j;}else {c = s[i];if (c == ')') {s0 += c;bracketsLevel -= 1;}else if (c == '(') {//如果整个括号被开根式，根号在括号左边bracketPos[bracketsLevel] = x + plot.measureText(s0);					s0 += c;bracketsLevel+=1;//过了括号就是过了一道关，要刷新坐标xMem[bracketsLevel] = x + plot.measureText(s0);}else if (c == '+' || c == '-' || c == '*' || c == '/' || c == '÷'|| c == '=' || c == ' ') {if (c == '*') {if (i > 0 && ((s[i-1] == ')' && /[0-9]/.test(s[i-2]) ||/[0-9]/.test(s[i-1]))) &&((s[i+1] == '(' && /[0-9]/.test(s[i+2])) || /[0-9]/.test(s[i+1]))) {//对于乘号前后都是数字的情况，把乘号改成叉号c = ' \u00D7 ';}else {//对于代数式中，乘号改为点号c = ' \u00B7 ';}}//如果是运算符后的数被开根式，根号在运算符右边if (c == '-' || c == '/') {s0 += ' '+c+' ';}else {s0 += c;}if (bracketsLevel < 3) {xMem[bracketsLevel] = x+plot.measureText(s0);}						}else if (c == splitChar) { //隔字符if (bracketsLevel < 3) {xMem[bracketsLevel] = x+plot.measureText(s0)-3;}}else {s0 += c;				}				}}if (s0 != '') { //先把正常字符打印出if (r1 != 20) { //字体字号大小还在上标状态r1 = 20;this.setNormalFont();				}measure = plot.measureText(s0);plot.fillText(s0, x, y, measure);x += measure;}plot.restore();}//集合符号，集合表达式的书写this.printSet = function(text, xPos, yPos, style, splitChar) {//隔字符splitChar = splitChar ? splitChar : '|';var s = text ? text : '';xPos = xPos ? xPos : 0;yPos = yPos ? yPos : 0;style = style ? style : 'black';if (s != '') {//希腊字母表(存此用于Ctrl C/V//ΑΒΓΔΕΖΗΘΙΚΛΜΝΞΟΠΡΣΤΥΦΧΨΩ//αβγδεζηθικλμνξοπρστυφχψω//s = s.replace(/\[B\]/ig, '\u2208'); //∈s = s.replace(/\[NB\]/ig, '\u2209'); //不属于s = s.replace(/\[S\]/ig, '\u2286'); //包含于(是子集)s = s.replace(/\[SS\]/ig, '\u2287'); //包含s = s.replace(/\[ST\]/ig, '\u228A'); //真包含于(是真子集)s = s.replace(/\[SST\]/ig, '\u228B'); //真包含s = s.replace(/\[UU\]/ig, '\u222A'); //并集 ，由于U表示全集，又常为下标，此处错开s = s.replace(/\[I\]/ig, '\u2229'); //交集s = s.replace(/\[C\]/ig, '\u2201'); //补集s = s.replace(/\[INF\]/ig, '\u221E'); //无穷大s = s.replace(/\[NULL\]/ig, '\u2205');//空集s = s.replace(/\[&\]/ig, '\u2227');//且s = s.replace(/\[\|\]/ig, '\u2228');//或s = s.replace(/\[~\]/ig, '﹁');//非s = s.replace(/\[ALL\]/ig, '\u2200');//全称量词 Universal quantifiers = s.replace(/\[Exist\]/ig, '\u2203');//存在量词 Existential quantifier}return this.print(s, xPos, yPos, style, splitChar);}//微积分符号this.printIntegral = function(text, xPos, yPos, style, splitChar) {//隔字符splitChar = splitChar ? splitChar : '|';var s = text ? text : '';xPos = xPos ? xPos : 0;yPos = yPos ? yPos : 0;style = style ? style : 'black';if (s != '') {//希腊字母表(存此用于Ctrl C/V//ΑΒΓΔΕΖΗΘΙΚΛΜΝΞΟΠΡΣΤΥΦΧΨΩ//αβγδεζηθικλμνξοπρστυφχψω//s = s.replace(/\[S\]/ig, '\u222B'); //定积分符号 一次s = s.replace(/\[SS\]/ig, '\u222C');//定积分符号 二次s = s.replace(/\[SSS\]/ig, '\u222C');//定积分符号 三次s = s.replace(/\[INF\]/ig, '\u221E'); //无穷大}return this.print(s, xPos, yPos, style, splitChar);}}</span>

本节到此结束，欲知后事如何，请看下回分解。


这篇关于[从头学数学] 第192节 导数及其应用的文章就介绍到这儿，希望我们推荐的文章对编程师们有所帮助！


http://www.chinasem.cn/article/368403。

相关文章

Python使用Tkinter打造一个完整的桌面应用

Python使用Tkinter打造一个完整的桌面应用

《Python使用Tkinter打造一个完整的桌面应用》在Python生态中,Tkinter就像一把瑞士军刀,它没有花哨的特效,却能快速搭建出实用的图形界面,作为Python自带的标准库,无需安装即可... 目录一、界面搭建：像搭积木一样组合控件二、菜单系统：给应用装上“控制中枢”三、事件驱动：让界面“活”
阅读更多...
如何确定哪些软件是Mac系统自带的? Mac系统内置应用查看技巧

如何确定哪些软件是Mac系统自带的? Mac系统内置应用查看技巧

《如何确定哪些软件是Mac系统自带的?Mac系统内置应用查看技巧》如何确定哪些软件是Mac系统自带的？mac系统中有很多自带的应用，想要看看哪些是系统自带，该怎么查看呢？下面我们就来看看Mac系统内... 在MAC电脑上，可以使用以下方法来确定哪些软件是系统自带的：1.应用程序文件夹打开应用程序文件夹
阅读更多...
Python Flask 库及应用场景

Python Flask 库及应用场景

《PythonFlask库及应用场景》Flask是Python生态中​轻量级且高度灵活的Web开发框架,基于WerkzeugWSGI工具库和Jinja2模板引擎构建,下面给大家介绍PythonFl... 目录一、Flask 库简介二、核心组件与架构三、常用函数与核心操作 ​1. 基础应用搭建​2. 路由与参
阅读更多...
Spring Boot中的YML配置列表及应用小结

Spring Boot中的YML配置列表及应用小结

《SpringBoot中的YML配置列表及应用小结》在SpringBoot中使用YAML进行列表的配置不仅简洁明了,还能提高代码的可读性和可维护性,：本文主要介绍SpringBoot中的YML配... 目录YAML列表的基础语法在Spring Boot中的应用从YAML读取列表列表中的复杂对象其他注意事项总
阅读更多...
电脑系统Hosts文件原理和应用分享

电脑系统Hosts文件原理和应用分享

《电脑系统Hosts文件原理和应用分享》Hosts是一个没有扩展名的系统文件，当用户在浏览器中输入一个需要登录的网址时，系统会首先自动从Hosts文件中寻找对应的IP地址，一旦找到，系统会立即打开对应... Hosts是一个没有扩展名的系统文件，可以用记事本等工具打开，其作用就是将一些常用的网址域名与其对应
阅读更多...
CSS 样式表的四种应用方式及css注释的应用小结

CSS 样式表的四种应用方式及css注释的应用小结

《CSS样式表的四种应用方式及css注释的应用小结》：本文主要介绍了CSS样式表的四种应用方式及css注释的应用小结，本文通过实例代码给大家介绍的非常详细，详细内容请阅读本文，希望能对你有所帮助... 一、外部 css（推荐方式）定义：将 CSS 代码保存为独立的 .css 文件，通过 <link> 标签
阅读更多...
Python使用Reflex构建现代Web应用的完全指南

Python使用Reflex构建现代Web应用的完全指南

《Python使用Reflex构建现代Web应用的完全指南》这篇文章为大家深入介绍了Reflex框架的设计理念,技术特性,项目结构,核心API,实际开发流程以及与其他框架的对比和部署建议,感兴趣的小伙... 目录什么是 ReFlex？为什么选择 Reflex？安装与环境配置构建你的第一个应用核心概念解析组件
阅读更多...
C#通过进程调用外部应用的实现示例

C#通过进程调用外部应用的实现示例

《C#通过进程调用外部应用的实现示例》本文主要介绍了C#通过进程调用外部应用的实现示例,以WINFORM应用程序为例,在C#应用程序中调用PYTHON程序,具有一定的参考价值,感兴趣的可以了解一下... 目录窗口程序类进程信息类 系统设置类 以WINFORM应用程序为例，在C#应用程序中调用python程序
阅读更多...
Java应用如何防止恶意文件上传

Java应用如何防止恶意文件上传

《Java应用如何防止恶意文件上传》恶意文件上传可能导致服务器被入侵,数据泄露甚至服务瘫痪,因此我们必须采取全面且有效的防范措施来保护Java应用的安全,下面我们就来看看具体的实现方法吧... 目录恶意文件上传的潜在风险常见的恶意文件上传手段防范恶意文件上传的关键策略严格验证文件类型检查文件内容控制文件存储
阅读更多...
CSS3 布局样式及其应用举例

CSS3 布局样式及其应用举例

《CSS3布局样式及其应用举例》CSS3的布局特性为前端开发者提供了无限可能，无论是Flexbox的一维布局还是Grid的二维布局，它们都能够帮助开发者以更清晰、简洁的方式实现复杂的网页布局，本文给... 目录深入探讨 css3 布局样式及其应用引言一、CSS布局的历史与发展1.1 早期布局的局限性1.2
阅读更多...

Copyright China编程 23002807@qq.com

沪ICP备2023033072号-2