本文主要是介绍TH方程学习 (7),希望对大家解决编程问题提供一定的参考价值,需要的开发者们随着小编来一起学习吧!
一、内容介绍
TH存在广泛应用,在下面案例中,将介绍几种相对运动模型,斜滑接近模型,本节学习斜滑接近制导方法能够对接近时间、接近方向以及自主接近过程的相对速度进行控制。施加脉冲时刻追踪器的位置连线可构成一条直线,即理想轨道,实际接近轨道和理想接近轨道在脉冲施加时刻相交。脉冲施加的次数越多,则实际轨道偏离理想轨道越少;脉冲施加次数趋近于无穷大时,实际交会轨道将会和理想轨道重合,接近一条直线,从大范围看,多脉冲斜滑接近整个过程基本是沿直线运动的。
设追踪器在接近段的初始时刻,相对运动状态为和
,接近段终端时刻
的相对状态为
和
。设追踪器沿准直线完成对目标器的接近,由
指向
的直线矢量
为规划轨迹。所以,在任意时刻
有
其中,为追踪器在该直线上某点处的位置矢量。
矢量的单位矢量为
其中,表示矢量
在VVLH坐标系中投影与三个坐标轴的夹角,从而决定了接近方向。矢量可以表示为
在接近过程中,可根据接近轨迹快速性和安全性等多种需求来确定的变化关系,即设计理想的交会轨迹。典型的相对运动速度变化模式有指数型,这里采用指数型,
和
为线性关系
上式中为斜率。则整个接近段的转移时间T为
设接近段采用多脉冲分段控制,作用次速度脉冲使绕飞卫星在时间T内从初始位置
转移到终点位置
。任意控制段的两次脉冲作用的时间间隔是相同的,即
。
在时刻,经过第m次速度脉冲后,绕飞卫星从
转移到
,有
每个阶段相当于一次双脉冲轨道转移,可通过如下编程
% 本节旨在利用TH方程实现椭圆的轨道的滑行制导
clc;clear
% 初始化条件
Ecc = 0.1;
Perigee= 500;
TA = 45;
N = 5 ; %施加脉冲次数
r_i = [1;1;1];
v_i = [0.01;0.01;0.01];
% 期望末端条件
r_f = [0.1;0;0];
v_f = [0;0;0];
% 求出初始相对距离
rho0 = norm(r_i-r_f);
rhof = 0;%设计初始和结束沿着rho方向的速度
drho0 = -0.005;
drhof = -0.0001;
a = (drho0-drhof)/rho0;
t = 1/a *log(drhof/drho0);
% 求出单位矢量
u_rho = (r_i-r_f)/rho0;rho1_vec = zeros(3,N);
rho1_vec(:,1)= r_i;
% 记录每次施加脉冲前的速度
vvff = zeros(3,N);
vvff(:,1) = v_i;
% 记录每次施加脉冲后的速度
vvrr = zeros(3,N);
vvrr(:,N) = v_f;
delta_t = t/(N-1);
% 脉冲希望到达的位置
for i=1:N-1t1 = i*t/(N-1);rho1 = rho0*exp(a*t1)+drhof/a*(exp(a*t1)-1);rho1_vec(:,i+1) = r_f+rho1*u_rho; [v,Phi,vv] = TH_solver(Ecc,Perigee,TA,rho1_vec(:,i),vvff(:,i),t/(N-1));Phiall{i} = Phi;Phi_rr = Phi(1:3,1:3);Phi_rv = Phi(1:3,4:6);vvrr(:,i) = inv(Phi_rv)*(rho1_vec(:,i+1)-Phi_rr*rho1_vec(:,i));[x,Phi0,xx] = TH_solver(Ecc,Perigee,TA,rho1_vec(:,i),vvrr(:,i),t/(N-1));yy(:,i) = x(1:3);vvff(:,i+1) = x(4:6);
end
dv=vvrr-vvff;
Phiall{4}*[rho1_vec(:,4);vvrr(:,4)];
tt = linspace(0,delta_t,1000);
for ss=1:Nfor j=1:length(tt)[mm,Phi00,zz0]= TH_solver(Ecc,Perigee,TA,rho1_vec(:,ss),vvrr(:,ss),tt(j));tarx((ss-1)*1000+j) = mm(1);tary((ss-1)*1000+j) = mm(2);tarz((ss-1)*1000+j) = mm(3);end
end
% 使用STK验证VVLH坐标系
uiApplication = actxGetRunningServer('STK12.application');
root = uiApplication.Personality2;
checkempty = root.Children.Count;
if checkempty ~= 0root.CurrentScenario.Unloadroot.CloseScenario;
end
root.NewScenario('VVLH');
StartTime = '26 Jan 2024 04:00:00.000'; % 场景开始时间
StopTime = '10 Feb 2024 04:00:00.000'; % 场景结束时间
root.ExecuteCommand(['SetAnalysisTimePeriod * "',StartTime,'" "',StopTime,'"']);
root.ExecuteCommand(' Animate * Reset');
SatName = 'Target'; % SAR_ GX_ Sat_ GX_1_ SAR_1_
satellite = root.CurrentScenario.Children.New('eSatellite', SatName);
satellite.SetPropagatorType('ePropagatorAstrogator'); % 不设置的时候默认为二体模型 ePropagatorJ4Perturbation
satellite.Propagator;
% 目标星初始状态
Perigee = 500;
T = 60;
% 追踪星在VVLH坐下的相对位置
delta_r = [1;1;1];
delta_v = [0.01;0.01;0.01];
Perige = 6378.137+Perigee;
ecc = 0.1;
sma = Perige/(1-ecc);
Inc = 30;
w = 0;
RAAN = 0;
TA = 45;
root.ExecuteCommand(['Astrogator */Satellite/',SatName,' SetValue MainSequence.SegmentList Initial_State Propagate']);
InitialState=satellite.Propagator.MainSequence.Item(0);
%% 初始化卫星参数
root.ExecuteCommand(['Astrogator */Satellite/',SatName,' SetValue MainSequence.SegmentList.Initial_State.CoordinateType Modified Keplerian']);
root.ExecuteCommand(['Astrogator */Satellite/',SatName,' SetValue MainSequence.SegmentList.Initial_State.InitialState.Epoch ',StartTime,' UTCG']);
root.ExecuteCommand(['Astrogator */Satellite/',SatName,' SetValue MainSequence.SegmentList.Initial_State.InitialState.Keplerian.sma ',num2str(sma),' km']);
root.ExecuteCommand(['Astrogator */Satellite/',SatName,' SetValue MainSequence.SegmentList.Initial_State.InitialState.Keplerian.ecc ',num2str(ecc)]);
root.ExecuteCommand(['Astrogator */Satellite/',SatName,' SetValue MainSequence.SegmentList.Initial_State.InitialState.Keplerian.inc ',num2str(Inc),' deg']);
root.ExecuteCommand(['Astrogator */Satellite/',SatName,' SetValue MainSequence.SegmentList.Initial_State.InitialState.Keplerian.w ',num2str(w),' deg']);
root.ExecuteCommand(['Astrogator */Satellite/',SatName,' SetValue MainSequence.SegmentList.Initial_State.InitialState.Keplerian.RAAN ',num2str(RAAN),' deg']);
root.ExecuteCommand(['Astrogator */Satellite/',SatName,' SetValue MainSequence.SegmentList.Initial_State.InitialState.Keplerian.TA ',num2str(TA),' deg']);
%% 二体传播
Propagate=satellite.Propagator.MainSequence.Item(1);
Propagate.PropagatorName='Earth Point Mass';
root.ExecuteCommand(['Astrogator */Satellite/',SatName,' RunMCS']);% 插入目标星
SatName2 = 'Chaser';
satellite2 = root.CurrentScenario.Children.New('eSatellite', SatName2);
satellite2.SetPropagatorType('ePropagatorAstrogator'); % 不设置的时候默认为二体模型 ePropagatorJ4Perturbation
satellite2.Propagator;
InitialState2=satellite2.Propagator.MainSequence.Item(0);
InitialState2.CoordSystemName='Satellite/Target VVLH';
InitialState2.Element.X=delta_r(1);
InitialState2.Element.Y=delta_r(2);
InitialState2.Element.Z=delta_r(3);
InitialState2.Element.Vx=delta_v(1);
InitialState2.Element.Vy=delta_v(2);
InitialState2.Element.Vz=delta_v(3);
Propagate2=satellite2.Propagator.MainSequence.Item(1);
Propagate2.PropagatorName='Earth Point Mass';
for j=1:NManeuverName=['Maneuver',num2str(j)];PropagateName=['Propagate',num2str(j)];satellite2.Propagator.MainSequence.Insert('eVASegmentTypeManeuver',ManeuverName,'Propagate');Maneuver=satellite2.Propagator.MainSequence.Item(ManeuverName);root.ExecuteCommand(['Astrogator */Satellite/',SatName2,' SetValue MainSequence.SegmentList.',ManeuverName,'.ImpulsiveMnvr.AttitudeControl Thrust Vector']);Maneuver.Maneuver.AttitudeControl.ThrustAxesName='Satellite VVLH.Axes';Maneuver.Maneuver.AttitudeControl.X=dv(1,j)*1000;Maneuver.Maneuver.AttitudeControl.Y=dv(2,j)*1000;Maneuver.Maneuver.AttitudeControl.Z=dv(3,j)*1000;satellite2.Propagator.MainSequence.Insert('eVASegmentTypePropagate',PropagateName,'Propagate');Propagate3=satellite2.Propagator.MainSequence.Item(PropagateName);Propagate3.PropagatorName='Earth Point Mass';Propagate3.Properties.Color=255;Propagate3.StoppingConditions.Item(0).Properties.Trip = delta_t;
end
satellite2.Propagator.MainSequence.Cut('Propagate')
root.ExecuteCommand(['Astrogator */Satellite/',SatName2,' RunMCS']);
% 报告二颗卫星的三维关系
satellite.VO.OrbitSystems.InertialByWindow.IsVisible=0;
satellite2.VO.OrbitSystems.InertialByWindow.IsVisible=0;
satellite2.VO.OrbitSystems.Add('Satellite/Target VVLH System')
satellite.VO.Vector.RefCrdns.Item(2).Visible=1;targetdata=root.ExecuteCommand(['Report_RM */Satellite/Target Style "VVLH" TimePeriod "26 Jan 2024 04:00:00.000" "26 Jan 2024 4:30:00.000" TimeStep 1']);
Num=targetdata.Count;
root.ExecuteCommand('Astrogator */Satellite/Target ClearDWCGraphics');
root.ExecuteCommand('Astrogator */Satellite/Chaser ClearDWCGraphics');
for j=1:Num-2struct=regexp(targetdata.Item(j),',','split');Tar_x(j)=str2double(struct{2});Tar_y(j)=str2double(struct{3});Tar_z(j)=str2double(struct{4});
endfigure(1)
plot3(Tar_x(1:floor(t)),Tar_y(1:floor(t)),Tar_z(1:floor(t)),'LineWidth',1);
hold on
plot3(tarx,tary,tarz,'LineWidth',1)
axis([-1.5 1.5 -1.5 1.5 -1.5 1.5])
set(gca,'XDir','reverse');
set(gca,'YDir','reverse');
set(gca,'ZDir','reverse');
xlabel('X axis(km)','FontName','Times New Roman')
ylabel('Y axis(km)','FontName','Times New Roman')
zlabel('Z axis(km)','FontName','Times New Roman')
title('e=0.1,Perigee=500km','FontName','Times New Roman')
grid onplot3(rho1_vec(1,:),rho1_vec(2,:),rho1_vec(3,:),'g.')
plot3(delta_r(1),delta_r(2),delta_r(3),'r.')legend('transfer trajectory(STK)','trasnfer trajecoty(TH)','Impulsive Point','Original','Location','Northeast')得到最终的结果,滑移的曲线如图所示
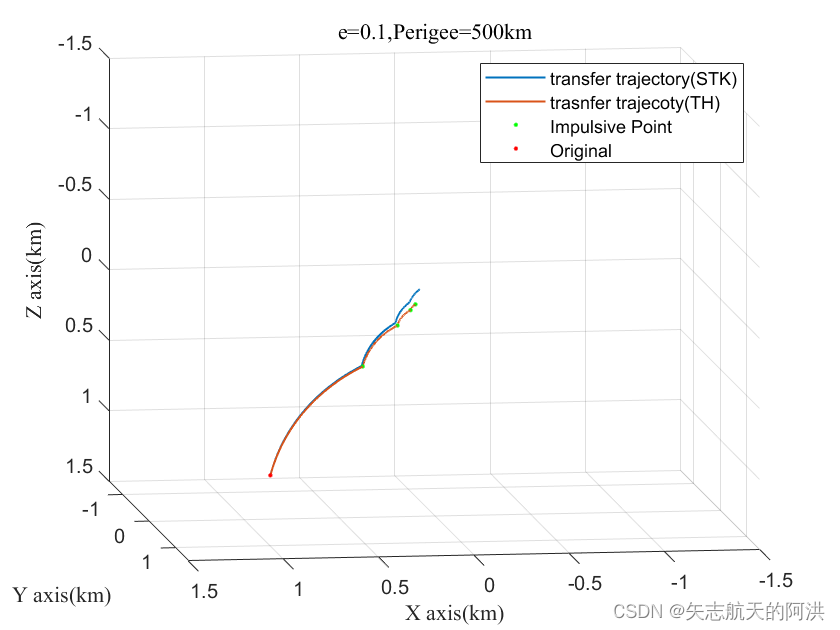
下图是每次施加脉冲前和施加脉冲后的位置速度,将上述脉冲形式写入STK,使用二体预报,发现计算出来的脉冲曲线与TH状态转移方程计算出来的曲线不一致,存在微小的误差。并且随着脉冲次数的增多,该误差会更加明显。
这篇关于TH方程学习 (7)的文章就介绍到这儿,希望我们推荐的文章对编程师们有所帮助!









