本文主要是介绍Codeforces 798C Mike and gcd problem (贪心)，希望对大家解决编程问题提供一定的参考价值，需要的开发者们随着小编来一起学习吧！
Mike has a sequence A = [a1, a2, ..., an] of length n. He considers the sequence B = [b1, b2, ..., bn] beautiful if the gcd of all its elements is bigger than 1, i.e.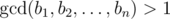 
.
Mike wants to change his sequence in order to make it beautiful. In one move he can choose an index i (1 ≤ i < n), delete numbers ai, ai + 1 and put numbers ai - ai + 1, ai + ai + 1 in their place instead, in this order. He wants perform as few operations as possible. Find the minimal number of operations to make sequence A beautiful if it's possible, or tell him that it is impossible to do so.
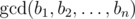is the biggest non-negative number d such that d divides bi for every i (1 ≤ i ≤ n).
The first line contains a single integer n (2 ≤ n ≤ 100 000) — length of sequence A.
The second line contains n space-separated integers a1, a2, ..., an (1 ≤ ai ≤ 109) — elements of sequence A.
Output on the first line "YES" (without quotes) if it is possible to make sequence A beautiful by performing operations described above, and "NO" (without quotes) otherwise.
If the answer was "YES", output the minimal number of moves needed to make sequence A beautiful.
2 1 1
YES 1
3 6 2 4
YES 0
2 1 3
YES 1
In the first example you can simply make one move to obtain sequence [0, 2] with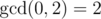 
.
In the second example the gcd of the sequence is already greater than 1.
题目链接：http://codeforces.com/contest/798/problem/C
题目大意：n个数，每次变换可以将a[i]和a[i+1]变成a[i] - a[i+1]和a[i] + a[i+1]，问变换多少次可以使这n个数的最大公约数大于1。
题目分析：若这n个数原本的最大公约数就大于1则输出，否则变换后的最大公约数必然是个偶数，证明分奇偶讨论下即可，对于两个相邻的奇数，一次变换即可变成两个偶数，一奇一偶需要变换两次。因此先处理两奇再处理一奇一偶。
#include <cstdio>
#include <cstring>
#include <algorithm>
using namespace std;
int const MAX = 1e5 + 5;
int a[MAX], n;int gcd(int a, int b) {return b == 0 ? a : gcd(b, a % b);
}int main() {scanf("%d", &n);int g = 0;for (int i = 0; i < n; i ++) {scanf("%d", &a[i]);g = gcd(g, a[i]);}if (g > 1) {printf("YES\n0\n");return 0;}int ans = 0;for (int i = 0; i + 1 < n; i ++) {if ((a[i] & 1) && (a[i + 1] & 1)) {a[i] = 2;a[i + 1] = 2;ans ++;i ++;}}for (int i = 0; i < n; i ++) {if (a[i] & 1) {ans += 2;}}printf("YES\n%d\n", ans);
}
这篇关于Codeforces 798C Mike and gcd problem (贪心)的文章就介绍到这儿，希望我们推荐的文章对编程师们有所帮助！
